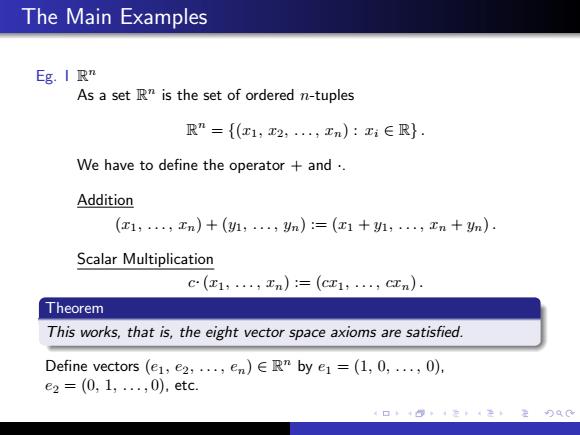
The Main Examples Eg.I Rm As a set R"is the set of ordered n-tuples R”={(x1,2,,xn):xi∈R} We have to define the operator and.. Addition (r1,,xn)+(1,,n)=(1+y1,,xn+n). Scalar Multiplication c.(1,...,n):=(c1;...,cUn). Theorem This works,that is,the eight vector space axioms are satisfied. Define vectors (e1,e2,...,en)E R"by e1=(1,0,...,0), e2=(0,1,.,0),etc. 4口++心++左+4生+定QCThe Main Examples Eg. I R n As a set R n is the set of ordered n-tuples R n = {(x1, x2, . . . , xn) : xi ∈ R} . We have to define the operator + and ·. Addition (x1, . . . , xn) + (y1, . . . , yn) := (x1 + y1, . . . , xn + yn). Scalar Multiplication c·(x1, . . . , xn) := (cx1, . . . , cxn). Theorem This works, that is, the eight vector space axioms are satisfied. Define vectors (e1, e2, . . . , en) ∈ R n by e1 = (1, 0, . . . , 0), e2 = (0, 1, . . . , 0), etc