
Abstract Vector Spaces Li Zhang Department of Mathematics Tongji University 4口,+心,4左4生+定QC
Abstract Vector Spaces Li Zhang Department of Mathematics Tongji University

Real Vector Space We will skip Chapter 1. Definition A vector space over R or(for short)a real vector space is a triple (V,+,where. 1 V is a set, 2 +is a binary operator that assigns to any pair v1,v2E V a new element vr+v2∈V, 3·is a binary operation that assigns to any pair c∈R and c∈Va new vector c.u∈V The operation satisfies 5 axioms. 4口++心++左+4生+定QC
Real Vector Space We will skip Chapter 1. Definition A vector space over R or (for short) a real vector space is a triple (V, +, ·) where, 1 V is a set, 2 + is a binary operator that assigns to any pair v1, v2 ∈ V a new element v1 + v2 ∈ V , 3 · is a binary operation that assigns to any pair c ∈ R and c ∈ V a new vector c·v ∈ V . The operation + satisfies 5 axioms

Axioms for Addition Al Commutativity u+v=v+u. A2 Associativity (u+v)+0=u+(v+w) A3 Existence of the zero vector There exists a unique element 0 of V such that v+0=v,for all v∈V A4 Existence of an additive inverse For each v E V,there exists a vector -v such that v+(-v)=0. We will abbreviate u+(-v)for u-v,so we have defined subtraction
Axioms for Addition + A1 Commutativity u + v = v + u. A2 Associativity (u + v) + w = u + (v + w). A3 Existence of the zero vector There exists a unique element 0 of V such that v + 0 = v, for all v ∈ V. A4 Existence of an additive inverse For each v ∈ V , there exists a vector −v such that v + (−v) = 0. We will abbreviate u + (−v) for u − v, so we have defined subtraction
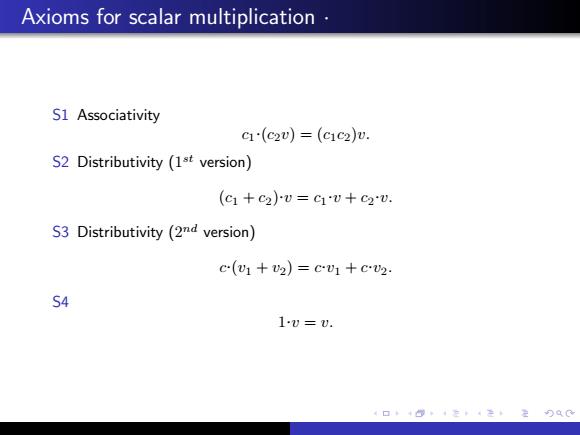
Axioms for scalar multiplication. S1 Associativity c·(c2w)=(c1c2)v. S2 Distributivity (1st version) (C1+C2)v=cv+C2v. S3 Distributivity (2nd version) c(v1+2)=CU1+C2 S4 1U=U. 4口+心左4生主9QG
Axioms for scalar multiplication · S1 Associativity c1·(c2v) = (c1c2)v. S2 Distributivity (1 st version) (c1 + c2)·v = c1·v + c2·v. S3 Distributivity (2 nd version) c·(v1 + v2) = c·v1 + c·v2. S4 1·v = v

Vector Space Axioms We will call the axioms A1,A2,A3,A4 and S1,S2,S3,S4 the vector space axioms. We will prove shortly that 0v=0, and (-1)w=-w. 4口+++左+4生+定QC
Vector Space Axioms We will call the axioms A1, A2, A3, A4 and S1, S2, S3, S4 the vector space axioms. We will prove shortly that 0·v = 0, and (−1)v = −v
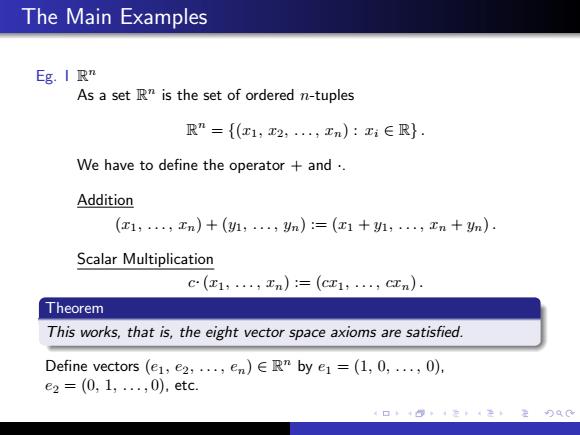
The Main Examples Eg.I Rm As a set R"is the set of ordered n-tuples R”={(x1,2,,xn):xi∈R} We have to define the operator and.. Addition (r1,,xn)+(1,,n)=(1+y1,,xn+n). Scalar Multiplication c.(1,...,n):=(c1;...,cUn). Theorem This works,that is,the eight vector space axioms are satisfied. Define vectors (e1,e2,...,en)E R"by e1=(1,0,...,0), e2=(0,1,.,0),etc. 4口++心++左+4生+定QC
The Main Examples Eg. I R n As a set R n is the set of ordered n-tuples R n = {(x1, x2, . . . , xn) : xi ∈ R} . We have to define the operator + and ·. Addition (x1, . . . , xn) + (y1, . . . , yn) := (x1 + y1, . . . , xn + yn). Scalar Multiplication c·(x1, . . . , xn) := (cx1, . . . , cxn). Theorem This works, that is, the eight vector space axioms are satisfied. Define vectors (e1, e2, . . . , en) ∈ R n by e1 = (1, 0, . . . , 0), e2 = (0, 1, . . . , 0), etc

The Main Examples Eg.II The space of real-valued functions on a set X Let X be a set and FR(X)be the set of real-valued function on the set X.Ve define+and·by (f+g)(x)=f(x)+g(x) (cf)(z):=cf(x). Exercise Show that Example ll includes Example I. Hint:Take X to be the n-element set {1,2,...,n}. 4口++左4生+定QC
The Main Examples Eg. II The space of real-valued functions on a set X Let X be a set and FR(X) be the set of real-valued function on the set X. We define + and · by (f + g)(x) := f(x) + g(x) (c·f)(x) := cf(x). Exercise Show that Example II includes Example I. Hint: Take X to be the n-element set {1, 2, . . . , n}
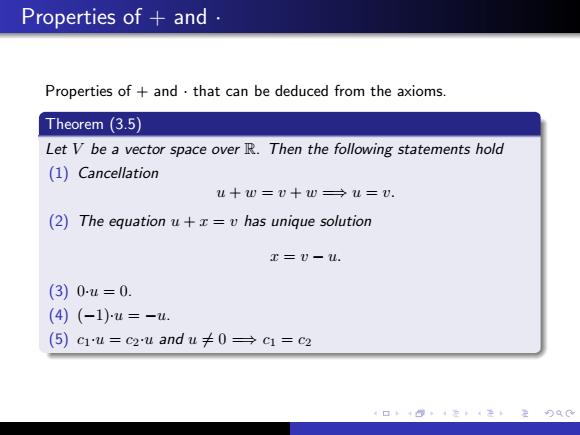
Properties of+and· Properties of+and.that can be deduced from the axioms. Theorem (3.5) Let V be a vector space over R.Then the following statements hold (1)Cancellation u十w=U+w→u=U. (2)The equation u+x=v has unique solution I=U-u. (3)0-u=0. (4)(-1)u=-u. (5)c1u=c2 u and u≠0→c1=c2 4口+心左4生主9QC
Properties of + and · Properties of + and · that can be deduced from the axioms. Theorem (3.5) Let V be a vector space over R. Then the following statements hold (1) Cancellation u + w = v + w =⇒ u = v. (2) The equation u + x = v has unique solution x = v − u. (3) 0·u = 0. (4) (−1)·u = −u. (5) c1·u = c2·u and u 6= 0 =⇒ c1 = c2

Properties of+and· Proof. (1)Add -w to each side. (2)Add -u to each side. (3)This one is tricky! Let 0 be the zero element in R(!!not the zero element in V).Then 0+0=0 (0+0)u=0u 0u+0u=0u. Subtract the vector 0.u from each side to get 0u=0. 4口+++左+4生+定QC
Properties of + and · Proof. (1) Add −w to each side. (2) Add −u to each side. (3) This one is tricky! Let 0 be the zero element in R (!! not the zero element in V ). Then 0 + 0 = 0 (0 + 0)·u = 0·u 0·u + 0·u = 0·u. Subtract the vector 0·u from each side to get 0·u = 0

Properties of+and· Proof(continued). (4)We want to show u+(-1)u=0 () From S4,(1.)uu,so LHS(*)=(1)u+(-1)u=(1+(-1)u 0-u=0rom(3). 4口+++左+4生+定QC
Properties of + and · Proof (continued). (4) We want to show u + (−1)·u = 0 (∗) From S4, (1·)u = u, so LHS(∗) = (1)·u + (−1)u = (1 + (−1))u 0·u = 0 from (3)