正在加载图片...
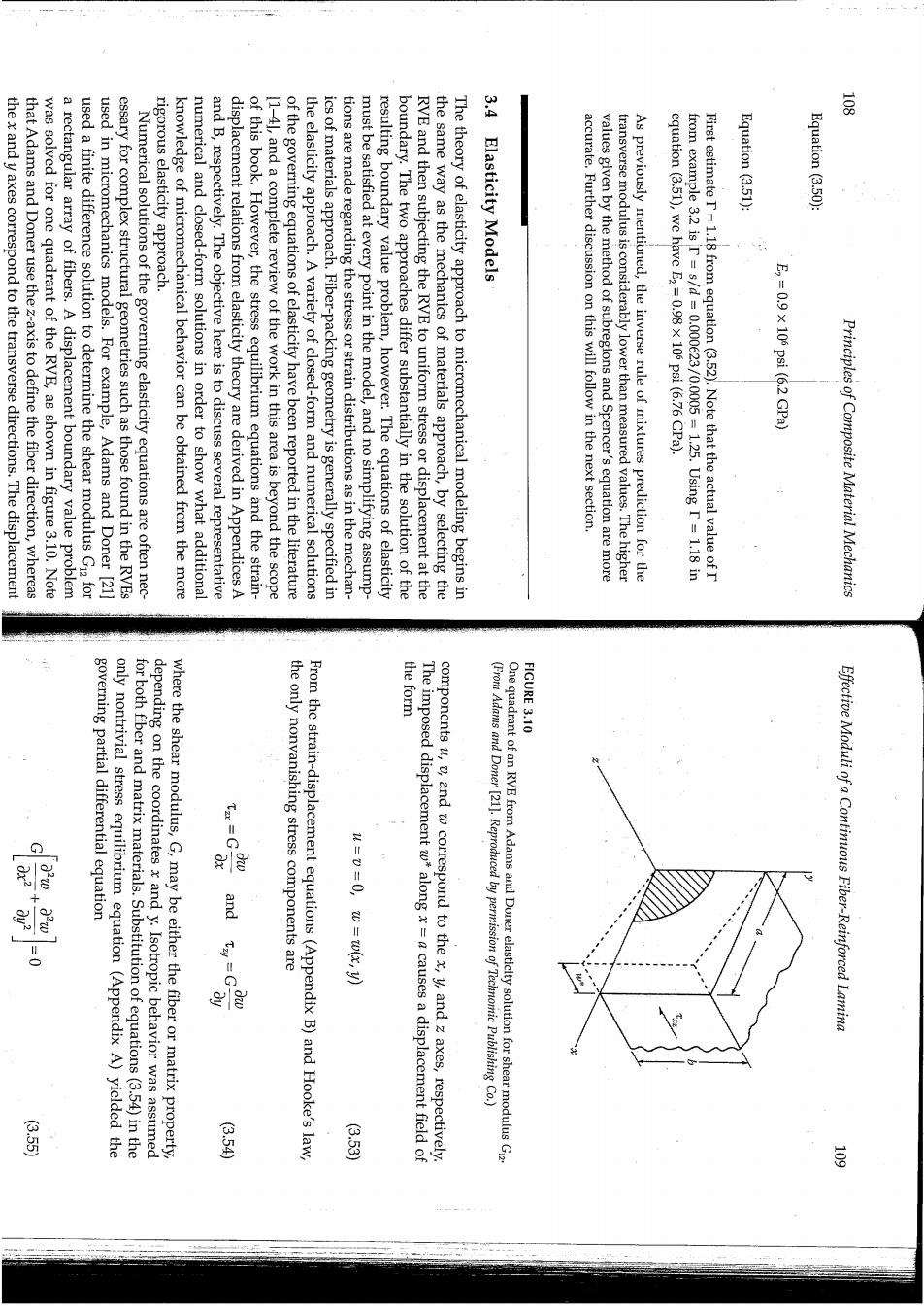
易 Equation (3.51): Equation (3.50): the x and y axes correspond to the transverse directions.The displacement that Adams and Doner use the z-axis to define the fiber direction,whereas was solved for one quadrant of the RVE,as shown in figure 3.10.Note a rectangular array of fibers.A displacement boundary value problem used a finite difference solution to determine the shear modulus Gi2 for used in micromechanics models.For example,Adams and Doner [21] essary for complex structural geometries such as those found in the RVEs Numerical solutions of the governing elasticity equations are often nec- rigorous elasticity approach. knowledge of micromechanical behavior can be obtained from the more numerical and closed-form solutions in order to show what additional and B,respectively.The objective here is to discuss several representative displacement relations from elasticity theory are derived in Appendices A of this book.However,the stress equilibrium equations and the strain- [1-41,and a complete review of the work in this area is beyond the scope of the governing equations of elasticity have been reported in the literature the elasticity approach.A variety of closed-form and numerical solutions ics of materials approach.Fiber-packing geometry is generally specified in tions are made regarding the stress or strain distributions as in the mechan- must be satisfied at every point in the model,and no simplifying assump- resulting boundary value problem,however.The equations of elasticity boundary.The two approaches differ substantially in the solution of the RVE and then subjecting the RVE to uniform stress or displacement at the the same way as the mechanics of materials approach,by selecting the The theory of elasticity approach to micromechanical modeling begins in Elasticity Models accurate.Further discussion on this will follow in the next section. values given by the method of subregions and Spencer's equation are more transverse modulus is considerably lower than measured values.The higher As previously mentioned,the inverse rule of mixtures prediction for the equation (3.51),we have E2=0.98 X 106 psi(6.76 GPa). 13/00005-e25.·1Bn6-3251-8/1-00002 fro fro First estimateT=1.18 from equation(3.52).Note that the actual value of I E2 =0.9 x 106 psi (6.2 GPa) Principles of Composite Material Mechanics the form governing partial differential equation FIGURE 3.10 only nontrivial stress equilibrium equation (Appendix A)yielded the for both fiber and matrix materials.Substitution of equations(3.54)in the depending on the coordinates x and y.Isotropic behavior was assumed where the shear modulus,G,may be either the fiber or matrix property, the only nonvanishing stress components are From the strain-displacement equations(Appendix B)and Hooke's law, The imposed displacement w*along x=a causes a displacement field of components u,v,and w correspond to the x,y,and z axes,respectively. (From Adams and Doner [21].Reproduced by permission of Technomic Publishing Co.) One quadrant of an RVE from Adams and Doner elasticity solution for shear modulus G. 2 Effective Moduli of a Continuous Fiber-Reinforced Lamina 854) e53 109