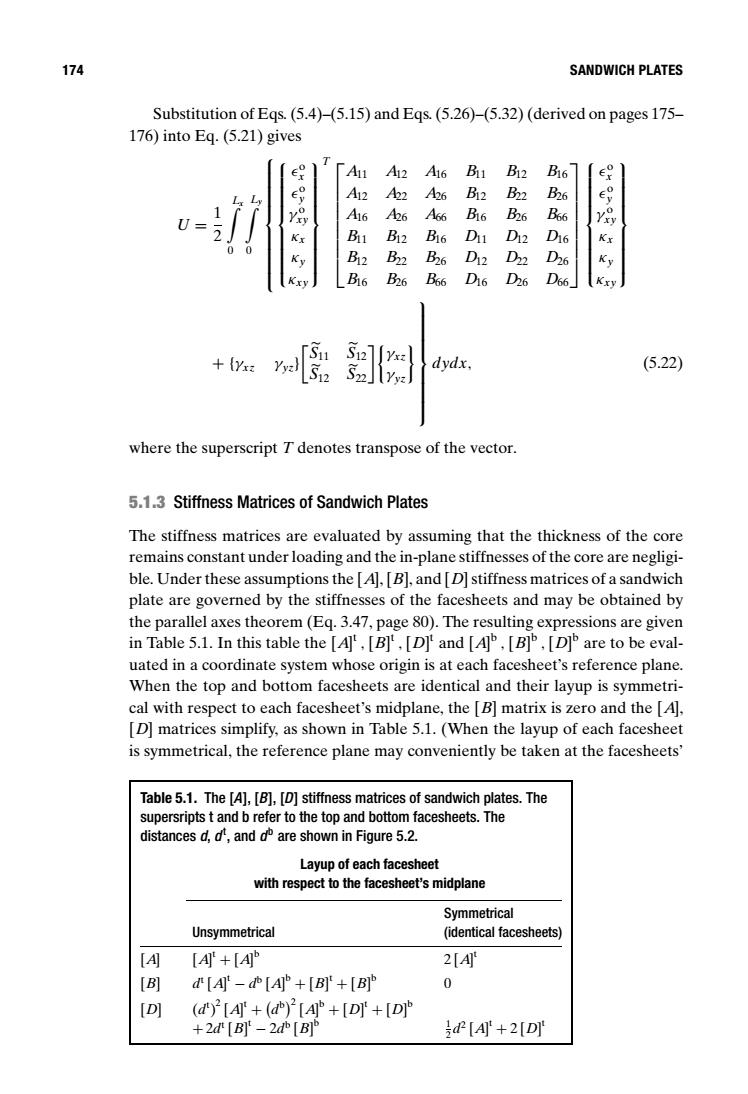
174 SANDWICH PLATES Substitution of Eqs.(5.4)-(5.15)and Egs.(5.26)-(5.32)(derived on pages 175- 176)into Eq.(5.21)gives e Au 42 A6 B11 B12 B16 42 A26 B12 B22 B26 A16 6 As6 B26 B B1 B12 B16 D D16 B2 B2 B26 D2 D26 Ky KxY B26 B66 D16 26 D66 +{ dydx, (5.22) where the superscript T denotes transpose of the vector. 5.1.3 Stiffness Matrices of Sandwich Plates The stiffness matrices are evaluated by assuming that the thickness of the core remains constant under loading and the in-plane stiffnesses of the core are negligi- ble.Under these assumptions the [A],[B],and D]stiffness matrices of a sandwich plate are governed by the stiffnesses of the facesheets and may be obtained by the parallel axes theorem(Eq.3.47,page 80).The resulting expressions are given in Table 5.1.In this table the [A],[B]',[D]'and [A],[B],[D]are to be eval- uated in a coordinate system whose origin is at each facesheet's reference plane. When the top and bottom facesheets are identical and their layup is symmetri- cal with respect to each facesheet's midplane,the B]matrix is zero and the [A], [D]matrices simplify,as shown in Table 5.1.(When the layup of each facesheet is symmetrical,the reference plane may conveniently be taken at the facesheets' Table 5.1.The [A],[B],[D]stiffness matrices of sandwich plates.The supersripts t and b refer to the top and bottom facesheets.The distances d,d,and d are shown in Figure 5.2. Layup of each facesheet with respect to the facesheet's midplane Symmetrical Unsymmetrical (identical facesheets) [4 [A+[A 2[A (B] d'[4-P[A+[B+[B 0 [D] (d2[4+(d)2[4+[D+[DP +2d [B]-2db [B] P[A'+2[D174 SANDWICH PLATES Substitution of Eqs. (5.4)–(5.15) and Eqs. (5.26)–(5.32) (derived on pages 175– 176) into Eq. (5.21) gives U = 1 2 ) Lx 0 ) Ly 0       o x o y γ o xy κx κy κxy    T          A11 A12 A16 B11 B12 B16 A12 A22 A26 B12 B22 B26 A16 A26 A66 B16 B26 B66 B11 B12 B16 D11 D12 D16 B12 B22 B26 D12 D22 D26 B16 B26 B66 D16 D26 D66             o x o y γ o xy κx κy κxy    + {γxz γyz} S 11 S 12 S 12 S 22!γxz γyz    dydx, (5.22) where the superscript T denotes transpose of the vector. 5.1.3 Stiffness Matrices of Sandwich Plates The stiffness matrices are evaluated by assuming that the thickness of the core remains constant under loading and the in-plane stiffnesses of the core are negligi￾ble. Under these assumptions the [A], [B], and [D]stiffness matrices of a sandwich plate are governed by the stiffnesses of the facesheets and may be obtained by the parallel axes theorem (Eq. 3.47, page 80). The resulting expressions are given in Table 5.1. In this table the [A] t , [B] t , [D] t and [A] b , [B] b , [D] b are to be eval￾uated in a coordinate system whose origin is at each facesheet’s reference plane. When the top and bottom facesheets are identical and their layup is symmetri￾cal with respect to each facesheet’s midplane, the [B] matrix is zero and the [A], [D] matrices simplify, as shown in Table 5.1. (When the layup of each facesheet is symmetrical, the reference plane may conveniently be taken at the facesheets’ Table 5.1. The [A], [B], [D] stiffness matrices of sandwich plates. The supersripts t and b refer to the top and bottom facesheets. The distances d, dt , and db are shown in Figure 5.2. Layup of each facesheet with respect to the facesheet’s midplane Symmetrical Unsymmetrical (identical facesheets) [A] [A] t + [A] b 2 [A] t [B] dt [A] t − db [A] b + [B] t + [B] b 0 [D] (dt ) 2 [A] t +  db 2 [A] b + [D] t + [D] b + 2dt [B] t − 2db [B] b 1 2d2 [A] t + 2 [D] t