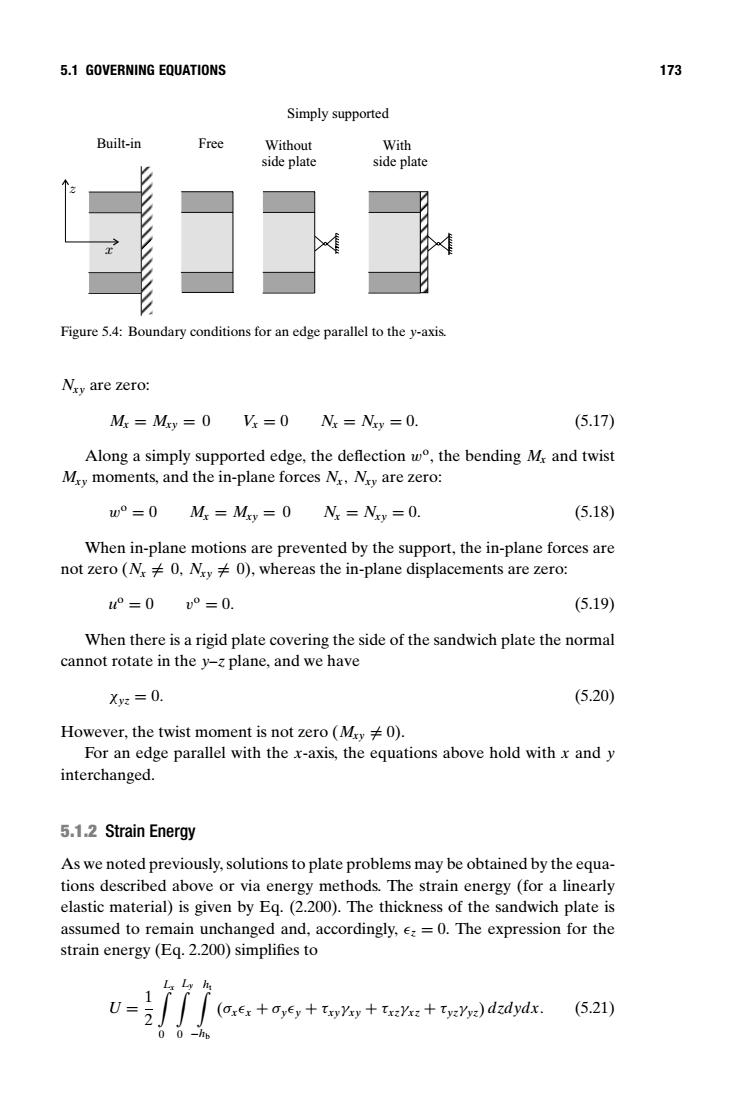
5.1 GOVERNING EQUATIONS 173 Simply supported Built-in Free Without With side plate side plate Figure 5.4:Boundary conditions for an edge parallel to the y-axis. Nry are zero: M=Mry=0 Vt=0 Nt=Nty=0. (5.17) Along a simply supported edge,the deflection wo,the bending Mr and twist Mry moments,and the in-plane forces N,Nry are zero: w°=0M=My=0N=Nxy=0. (5.18) When in-plane motions are prevented by the support,the in-plane forces are not zero(N 0,Ny0),whereas the in-plane displacements are zero: °=0v°=0. (5.19) When there is a rigid plate covering the side of the sandwich plate the normal cannot rotate in the y-z plane,and we have Xz=0. (5.20) However,the twist moment is not zero (My0). For an edge parallel with the x-axis,the equations above hold with x and y interchanged. 5.1.2 Strain Energy As we noted previously,solutions to plate problems may be obtained by the equa- tions described above or via energy methods.The strain energy(for a linearly elastic material)is given by Eq.(2.200).The thickness of the sandwich plate is assumed to remain unchanged and,accordingly,e=0.The expression for the strain energy(Eq.2.200)simplifies to L Ly h (oxex +oyey+txyrxy +txzYxz+tyzryz)dzdydx. (5.21)5.1 GOVERNING EQUATIONS 173 Built-in Free Simply supported Without With side plate side plate z x Figure 5.4: Boundary conditions for an edge parallel to the y-axis. Nxy are zero: Mx = Mxy = 0 Vx = 0 Nx = Nxy = 0. (5.17) Along a simply supported edge, the deflection wo, the bending Mx and twist Mxy moments, and the in-plane forces Nx, Nxy are zero: wo = 0 Mx = Mxy = 0 Nx = Nxy = 0. (5.18) When in-plane motions are prevented by the support, the in-plane forces are not zero (Nx = 0, Nxy = 0), whereas the in-plane displacements are zero: uo = 0 vo = 0. (5.19) When there is a rigid plate covering the side of the sandwich plate the normal cannot rotate in the y–z plane, and we have χyz = 0. (5.20) However, the twist moment is not zero (Mxy = 0). For an edge parallel with the x-axis, the equations above hold with x and y interchanged. 5.1.2 Strain Energy As we noted previously, solutions to plate problems may be obtained by the equa￾tions described above or via energy methods. The strain energy (for a linearly elastic material) is given by Eq. (2.200). The thickness of the sandwich plate is assumed to remain unchanged and, accordingly, z = 0. The expression for the strain energy (Eq. 2.200) simplifies to U = 1 2 ) Lx 0 ) Ly 0 ) ht −hb (σxx + σyy + τxyγxy + τxzγxz + τyzγyz) dzdydx. (5.21)