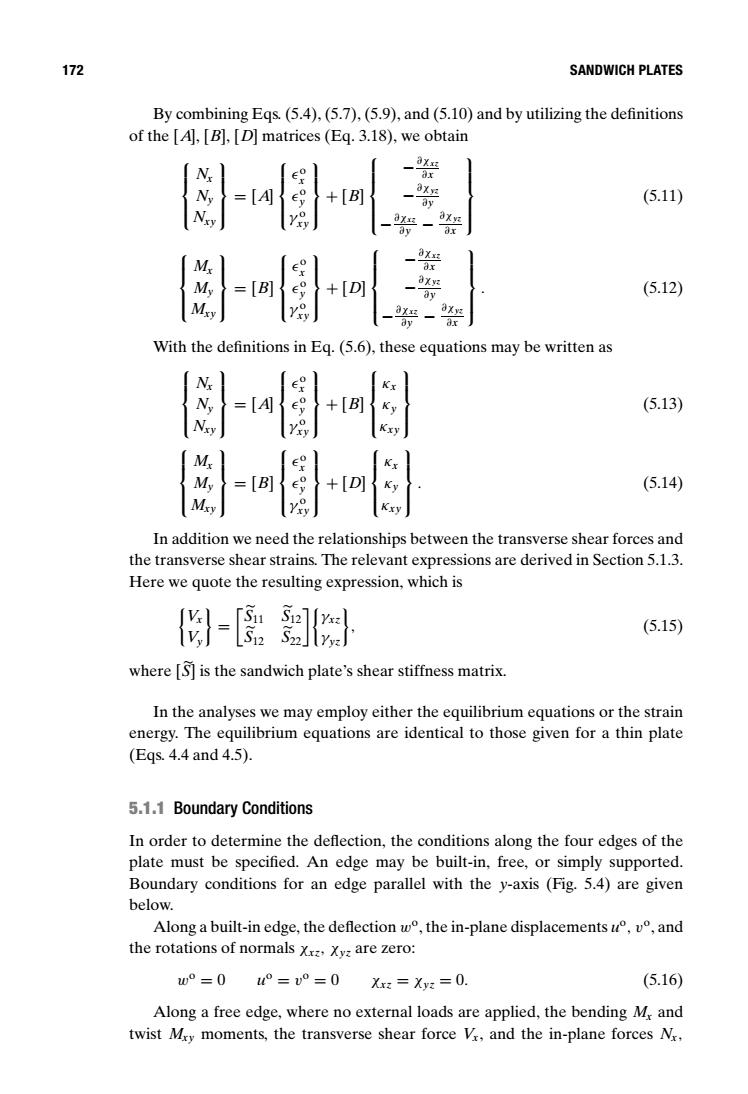
172 SANDWICH PLATES By combining Eqs.(5.4),(5.7),(5.9),and(5.10)and by utilizing the definitions of the [A],[B],[D]matrices(Eq.3.18),we obtain ax [4 +[B aXy ay (5.11) 8Xx ay ax =[B +[D (5.12) With the definitions in Eq.(5.6),these equations may be written as N Kx [4 +[B (5.13) Kxy M M [B +[D Ky (5.14) Mxy 8 Kxy In addition we need the relationships between the transverse shear forces and the transverse shear strains.The relevant expressions are derived in Section 5.1.3. Here we quote the resulting expression,which is -[原 (5.15) where [S]is the sandwich plate's shear stiffness matrix. In the analyses we may employ either the equilibrium equations or the strain energy.The equilibrium equations are identical to those given for a thin plate (Eqs.4.4and4.5). 5.1.1 Boundary Conditions In order to determine the deflection,the conditions along the four edges of the plate must be specified.An edge may be built-in,free,or simply supported. Boundary conditions for an edge parallel with the y-axis (Fig.5.4)are given below. Along a built-in edge,the deflection w°，the in-plane displacements.°，v°，and the rotations of normals xr,Xyz are zero: w°=0°=v°=0Xr:=Xz=0. (5.16) Along a free edge,where no external loads are applied,the bending M and twist Mry moments,the transverse shear force V,and the in-plane forces Mr,172 SANDWICH PLATES By combining Eqs. (5.4), (5.7), (5.9), and (5.10) and by utilizing the definitions of the [A], [B], [D] matrices (Eq. 3.18), we obtain    Nx Ny Nxy    = [A]    o x o y γ o xy    + [B]    −∂χxz ∂x −∂χyz ∂y −∂χxz ∂y − ∂χyz ∂x    (5.11)    Mx My Mxy    = [B]    o x o y γ o xy    + [D]    −∂χxz ∂x −∂χyz ∂y −∂χxz ∂y − ∂χyz ∂x    . (5.12) With the definitions in Eq. (5.6), these equations may be written as    Nx Ny Nxy    = [A]    o x o y γ o xy    + [B]    κx κy κxy    (5.13)    Mx My Mxy    = [B]    o x o y γ o xy    + [D]    κx κy κxy    . (5.14) In addition we need the relationships between the transverse shear forces and the transverse shear strains. The relevant expressions are derived in Section 5.1.3. Here we quote the resulting expression, which is  Vx Vy  = S 11 S 12 S 12 S 22!γxz γyz , (5.15) where [S ] is the sandwich plate’s shear stiffness matrix. In the analyses we may employ either the equilibrium equations or the strain energy. The equilibrium equations are identical to those given for a thin plate (Eqs. 4.4 and 4.5). 5.1.1 Boundary Conditions In order to determine the deflection, the conditions along the four edges of the plate must be specified. An edge may be built-in, free, or simply supported. Boundary conditions for an edge parallel with the y-axis (Fig. 5.4) are given below. Along a built-in edge, the deflection wo, the in-plane displacements uo, vo, and the rotations of normals χxz, χyz are zero: wo = 0 uo = vo = 0 χxz = χyz = 0. (5.16) Along a free edge, where no external loads are applied, the bending Mx and twist Mxy moments, the transverse shear force Vx, and the in-plane forces Nx,