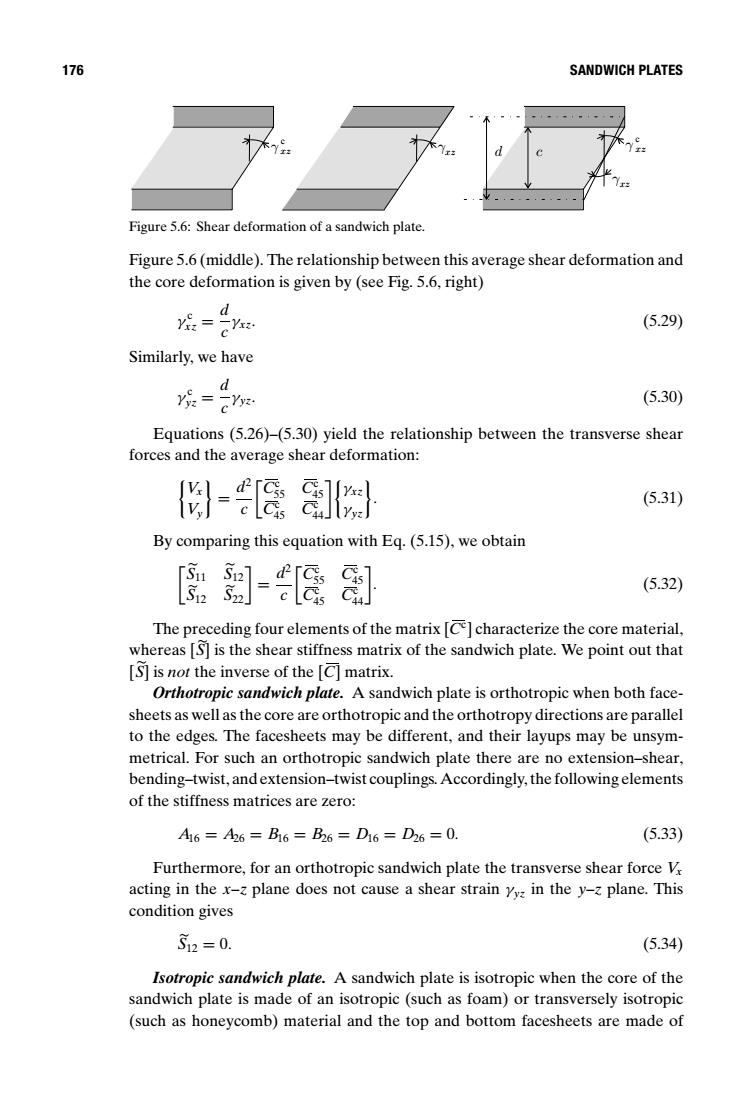
176 SANDWICH PLATES Figure 5.6:Shear deformation of a sandwich plate. Figure 5.6(middle).The relationship between this average shear deformation and the core deformation is given by (see Fig.5.6,right) d Y:=cYaz (5.29) Similarly,we have d i=cYys (5.30) Equations(5.26)-(5.30)yield the relationship between the transverse shear forces and the average shear deformation: (5.31) By comparing this equation with Eq.(5.15),we obtain [-[剧 (5.32) The preceding four elements of the matrix [Ce]characterize the core material, whereas [is the shear stiffness matrix of the sandwich plate.We point out that [S]is not the inverse of the [C]matrix. Orthotropic sandwich plate.A sandwich plate is orthotropic when both face- sheets as well as the core are orthotropic and the orthotropy directions are parallel to the edges.The facesheets may be different,and their layups may be unsym- metrical.For such an orthotropic sandwich plate there are no extension-shear, bending-twist,and extension-twist couplings.Accordingly,the following elements of the stiffness matrices are zero: A6=A26=B16=B26=D16=D26=0. (5.33) Furthermore,for an orthotropic sandwich plate the transverse shear force V acting in the x-z plane does not cause a shear strain yy in the y-z plane.This condition gives 5i2=0. (5.34) Isotropic sandwich plate.A sandwich plate is isotropic when the core of the sandwich plate is made of an isotropic (such as foam)or transversely isotropic (such as honeycomb)material and the top and bottom facesheets are made of176 SANDWICH PLATES γxz d c γxz γ c xz γ c xz Figure 5.6: Shear deformation of a sandwich plate. Figure 5.6 (middle). The relationship between this average shear deformation and the core deformation is given by (see Fig. 5.6, right) γ c xz = d c γxz. (5.29) Similarly, we have γ c yz = d c γyz. (5.30) Equations (5.26)–(5.30) yield the relationship between the transverse shear forces and the average shear deformation:  Vx Vy  = d2 c Cc 55 Cc 45 Cc 45 Cc 44 !γxz γyz . (5.31) By comparing this equation with Eq. (5.15), we obtain S 11 S 12 S 12 S 22! = d2 c Cc 55 Cc 45 Cc 45 Cc 44 ! . (5.32) The preceding four elements of the matrix [Cc ] characterize the core material, whereas [S ] is the shear stiffness matrix of the sandwich plate. We point out that [S ] is not the inverse of the [C] matrix. Orthotropic sandwich plate. A sandwich plate is orthotropic when both face￾sheets as well as the core are orthotropic and the orthotropy directions are parallel to the edges. The facesheets may be different, and their layups may be unsym￾metrical. For such an orthotropic sandwich plate there are no extension–shear, bending–twist, and extension–twist couplings. Accordingly, the following elements of the stiffness matrices are zero: A16 = A26 = B16 = B26 = D16 = D26 = 0. (5.33) Furthermore, for an orthotropic sandwich plate the transverse shear force Vx acting in the x–z plane does not cause a shear strain γyz in the y–z plane. This condition gives S 12 = 0. (5.34) Isotropic sandwich plate. A sandwich plate is isotropic when the core of the sandwich plate is made of an isotropic (such as foam) or transversely isotropic (such as honeycomb) material and the top and bottom facesheets are made of