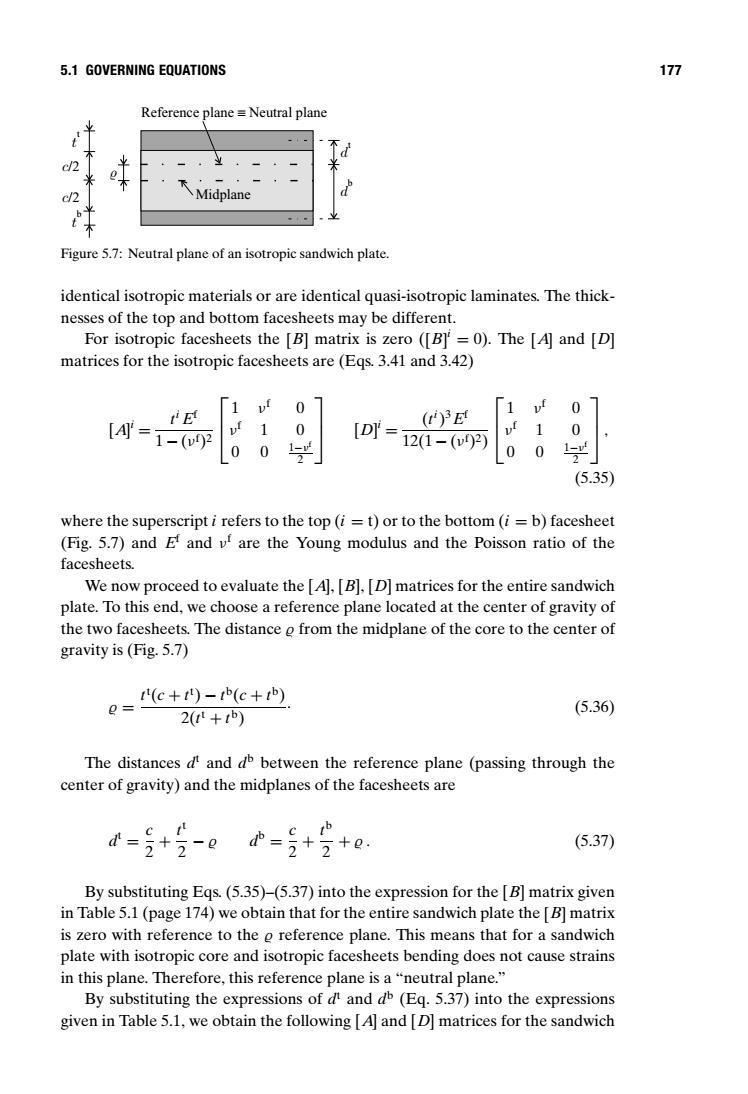
5.1 GOVERNING EQUATIONS 177 Reference plane Neutral plane 不 d 米 e c/2 Midplane Figure 5.7:Neutral plane of an isotropic sandwich plate. identical isotropic materials or are identical quasi-isotropic laminates.The thick- nesses of the top and bottom facesheets may be different. For isotropic facesheets the [B]matrix is zero ([B]=0).The [A]and [D] matrices for the isotropic facesheets are (Egs.3.41 and 3.42) 1 0 0 A= 1 1-(v02 00 00号 (5.35) where the superscript i refers to the top (i =t)or to the bottom (i b)facesheet (Fig.5.7)and Ef and v are the Young modulus and the Poisson ratio of the facesheets. We now proceed to evaluate the [A],[B],[D]matrices for the entire sandwich plate.To this end,we choose a reference plane located at the center of gravity of the two facesheets.The distance o from the midplane of the core to the center of gravity is (Fig.5.7) 1(c+1)-1b(c+1b) (5.36) 2(+1) The distances dt and db between the reference plane (passing through the center of gravity)and the midplanes of the facesheets are t=5+写-e=+5+e (5.37) By substituting Eqs.(5.35)-(5.37)into the expression for the B]matrix given in Table 5.1(page 174)we obtain that for the entire sandwich plate the [B]matrix is zero with reference to the o reference plane.This means that for a sandwich plate with isotropic core and isotropic facesheets bending does not cause strains in this plane.Therefore,this reference plane is a"neutral plane." By substituting the expressions of d and db(Eq.5.37)into the expressions given in Table 5.1,we obtain the following [A]and [D]matrices for the sandwich5.1 GOVERNING EQUATIONS 177 Reference plane Neutral plane ≡ t b t t d b d t c/2 c/2  Midplane Figure 5.7: Neutral plane of an isotropic sandwich plate. identical isotropic materials or are identical quasi-isotropic laminates. The thick￾nesses of the top and bottom facesheets may be different. For isotropic facesheets the [B] matrix is zero ([B] i = 0). The [A] and [D] matrices for the isotropic facesheets are (Eqs. 3.41 and 3.42) [A] i = ti Ef 1 − (νf )2    1 νf 0 νf 1 0 0 0 1−νf 2    [D] i = (ti )3Ef 12(1 − (νf )2)    1 νf 0 νf 1 0 0 0 1−νf 2    , (5.35) where the superscript i refers to the top (i = t) or to the bottom (i = b) facesheet (Fig. 5.7) and Ef and νf are the Young modulus and the Poisson ratio of the facesheets. We now proceed to evaluate the [A], [B], [D] matrices for the entire sandwich plate. To this end, we choose a reference plane located at the center of gravity of the two facesheets. The distance  from the midplane of the core to the center of gravity is (Fig. 5.7)  = tt (c + tt ) − tb(c + tb) 2(tt + tb) . (5.36) The distances dt and db between the reference plane (passing through the center of gravity) and the midplanes of the facesheets are dt = c 2 + tt 2 −  db = c 2 + tb 2 +  . (5.37) By substituting Eqs. (5.35)–(5.37) into the expression for the [B] matrix given in Table 5.1 (page 174) we obtain that for the entire sandwich plate the [B] matrix is zero with reference to the  reference plane. This means that for a sandwich plate with isotropic core and isotropic facesheets bending does not cause strains in this plane. Therefore, this reference plane is a “neutral plane.” By substituting the expressions of dt and db (Eq. 5.37) into the expressions given in Table 5.1, we obtain the following [A] and [D] matrices for the sandwich