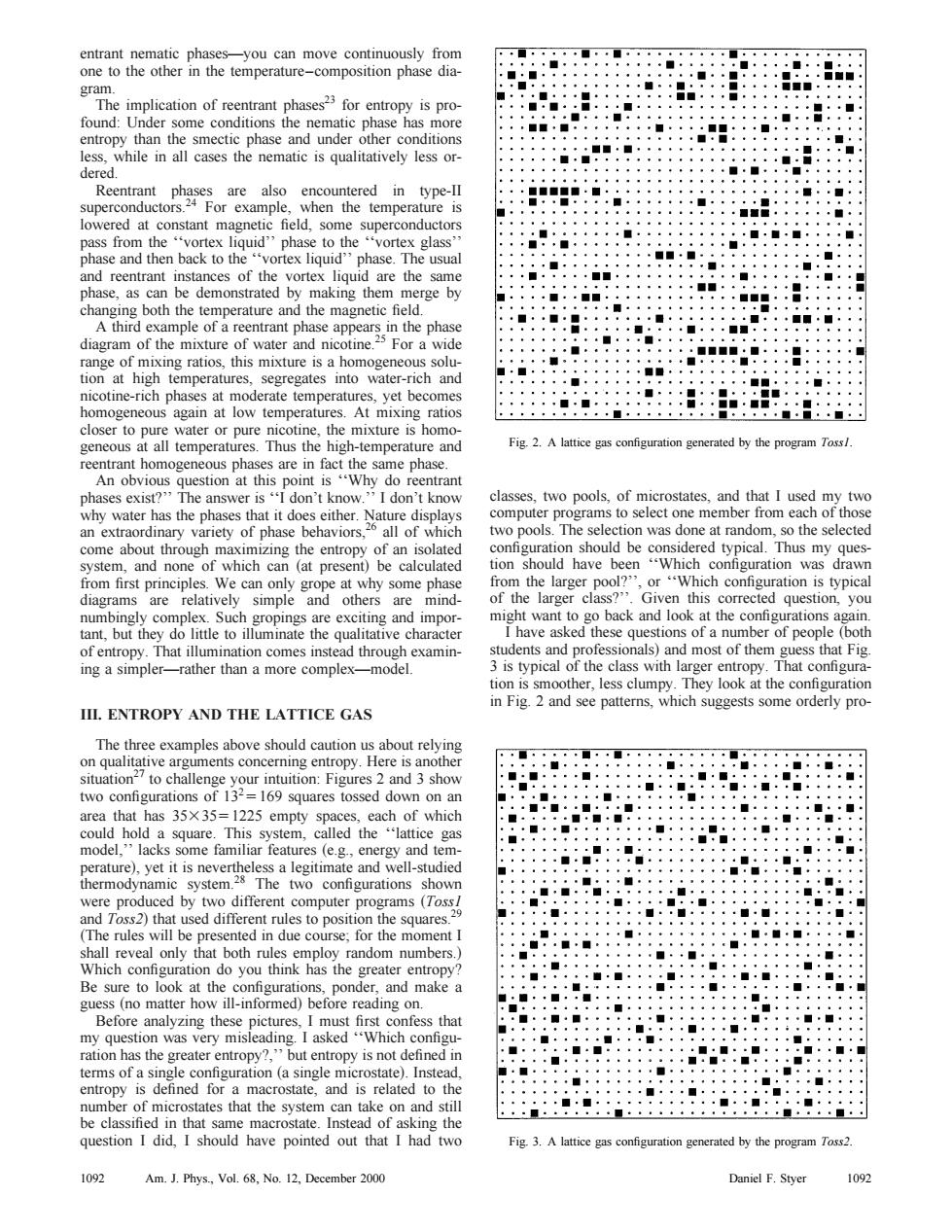
entrant nematic phases-you can move continuously from one to the other in the temperature-composition phase dia- gram. The implication of reentrant phases23 for entropy is pro- found:Under some conditions the nematic phase has more entropy than the smectic phase and under other conditions less,while in all cases the nematic is qualitatively less or- dered. Reentrant phases are also encountered in type-II superconductors.24 For example,when the temperature is lowered at constant magnetic field,some superconductors pass from the "vortex liquid"phase to the "vortex glass" phase and then back to the vortex liquid''phase.The usual and reentrant instances of the vortex liquid are the same phase,as can be demonstrated by making them merge by changing both the temperature and the magnetic field. A third example of a reentrant phase appears in the phase diagram of the mixture of water and nicotine.25 For a wide range of mixing ratios,this mixture is a homogeneous solu- tion at high temperatures,segregates into water-rich and nicotine-rich phases at moderate temperatures,yet becomes homogeneous again at low temperatures.At mixing ratios closer to pure water or pure nicotine,the mixture is homo- geneous at all temperatures.Thus the high-temperature and Fig.2.A lattice gas configuration generated by the program Toss/. reentrant homogeneous phases are in fact the same phase. An obvious question at this point is "Why do reentrant phases exist?"The answer is "I don't know."I don't know classes,two pools,of microstates,and that I used my two why water has the phases that it does either.Nature displays computer programs to select one member from each of those an extraordinary variety of phase behaviors,26 all of which two pools.The selection was done at random,so the selected come about through maximizing the entropy of an isolated configuration should be considered typical.Thus my ques- system,and none of which can (at present)be calculated tion should have been "Which configuration was drawn from first principles.We can only grope at why some phase from the larger pool?,orWhich configuration is typical diagrams are relatively simple and others are mind- of the larger class?".Given this corrected question,you numbingly complex.Such gropings are exciting and impor- might want to go back and look at the configurations again. tant,but they do little to illuminate the qualitative character I have asked these questions of a number of people (both of entropy.That illumination comes instead through examin- students and professionals)and most of them guess that Fig. ing a simpler-rather than a more complex-model. 3 is typical of the class with larger entropy.That configura- tion is smoother,less clumpy.They look at the configuration in Fig.2 and see patterns,which suggests some orderly pro- IIL.ENTROPY AND THE LATTICE GAS The three examples above should caution us about relying on qualitative arguments concerning entropy.Here is another situation2to challenge your intuition:Figures 2 and 3 show 。 two configurations of 132=169 squares tossed down on an area that has 35x35=1225 empty spaces,each of which could hold a square.This system,called the "lattice gas model,"lacks some familiar features (e.g.,energy and tem- perature),yet it is nevertheless a legitimate and well-studied thermodynamic system.28 The two configurations shown were produced by two different computer programs(Toss/ and Toss)that used different rules to position the squares2 (The rules will be presented in due course;for the moment I shall reveal only that both rules employ random numbers.) Which configuration do you think has the greater entropy? Be sure to look at the configurations,ponder,and make a guess(no matter how ill-informed)before reading on. Before analyzing these pictures,I must first confess that my question was very misleading.I asked "Which configu- ration has the greater entropy?,"but entropy is not defined in terms of a single configuration (a single microstate).Instead, entropy is defined for a macrostate,and is related to the number of microstates that the system can take on and still be classified in that same macrostate.Instead of asking the question I did,I should have pointed out that I had two Fig.3.A lattice gas configuration generated by the program Toss2. 1092 Am.J.Phys.,Vol.68,No.12,December 2000 Daniel F.Styer 1092entrant nematic phases—you can move continuously from one to the other in the temperature–composition phase dia￾gram. The implication of reentrant phases23 for entropy is pro￾found: Under some conditions the nematic phase has more entropy than the smectic phase and under other conditions less, while in all cases the nematic is qualitatively less or￾dered. Reentrant phases are also encountered in type-II superconductors.24 For example, when the temperature is lowered at constant magnetic field, some superconductors pass from the ‘‘vortex liquid’’ phase to the ‘‘vortex glass’’ phase and then back to the ‘‘vortex liquid’’ phase. The usual and reentrant instances of the vortex liquid are the same phase, as can be demonstrated by making them merge by changing both the temperature and the magnetic field. A third example of a reentrant phase appears in the phase diagram of the mixture of water and nicotine.25 For a wide range of mixing ratios, this mixture is a homogeneous solu￾tion at high temperatures, segregates into water-rich and nicotine-rich phases at moderate temperatures, yet becomes homogeneous again at low temperatures. At mixing ratios closer to pure water or pure nicotine, the mixture is homo￾geneous at all temperatures. Thus the high-temperature and reentrant homogeneous phases are in fact the same phase. An obvious question at this point is ‘‘Why do reentrant phases exist?’’ The answer is ‘‘I don’t know.’’ I don’t know why water has the phases that it does either. Nature displays an extraordinary variety of phase behaviors,26 all of which come about through maximizing the entropy of an isolated system, and none of which can ~at present! be calculated from first principles. We can only grope at why some phase diagrams are relatively simple and others are mind￾numbingly complex. Such gropings are exciting and impor￾tant, but they do little to illuminate the qualitative character of entropy. That illumination comes instead through examin￾ing a simpler—rather than a more complex—model. III. ENTROPY AND THE LATTICE GAS The three examples above should caution us about relying on qualitative arguments concerning entropy. Here is another situation27 to challenge your intuition: Figures 2 and 3 show two configurations of 1325169 squares tossed down on an area that has 3533551225 empty spaces, each of which could hold a square. This system, called the ‘‘lattice gas model,’’ lacks some familiar features ~e.g., energy and tem￾perature!, yet it is nevertheless a legitimate and well-studied thermodynamic system.28 The two configurations shown were produced by two different computer programs ~Toss1 and Toss2! that used different rules to position the squares.29 ~The rules will be presented in due course; for the moment I shall reveal only that both rules employ random numbers.! Which configuration do you think has the greater entropy? Be sure to look at the configurations, ponder, and make a guess ~no matter how ill-informed! before reading on. Before analyzing these pictures, I must first confess that my question was very misleading. I asked ‘‘Which configu￾ration has the greater entropy?,’’ but entropy is not defined in terms of a single configuration ~a single microstate!. Instead, entropy is defined for a macrostate, and is related to the number of microstates that the system can take on and still be classified in that same macrostate. Instead of asking the question I did, I should have pointed out that I had two classes, two pools, of microstates, and that I used my two computer programs to select one member from each of those two pools. The selection was done at random, so the selected configuration should be considered typical. Thus my ques￾tion should have been ‘‘Which configuration was drawn from the larger pool?’’, or ‘‘Which configuration is typical of the larger class?’’. Given this corrected question, you might want to go back and look at the configurations again. I have asked these questions of a number of people ~both students and professionals! and most of them guess that Fig. 3 is typical of the class with larger entropy. That configura￾tion is smoother, less clumpy. They look at the configuration in Fig. 2 and see patterns, which suggests some orderly pro￾Fig. 2. A lattice gas configuration generated by the program Toss1. Fig. 3. A lattice gas configuration generated by the program Toss2. 1092 Am. J. Phys., Vol. 68, No. 12, December 2000 Daniel F. Styer 1092