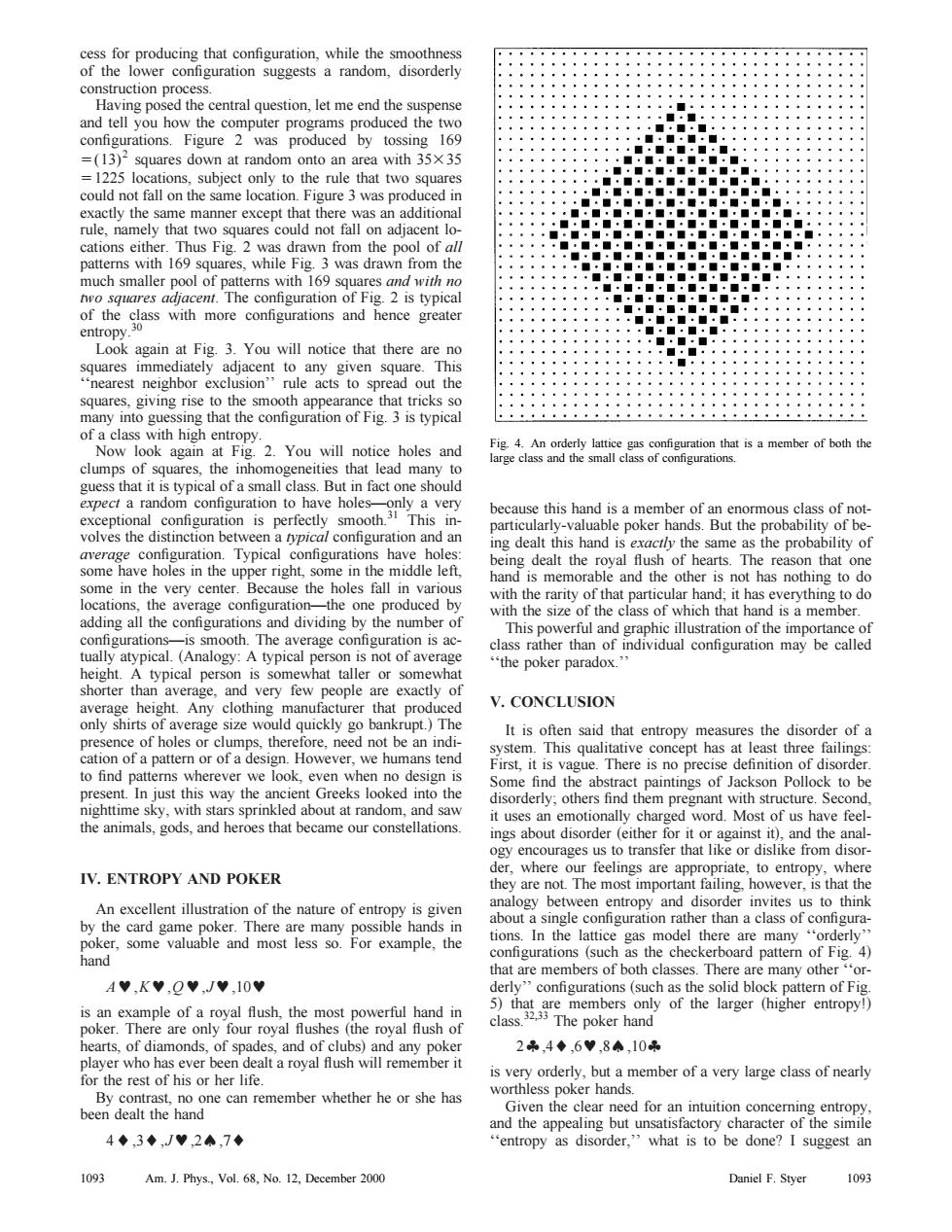
cess for producing that configuration,while the smoothness of the lower configuration suggests a random,disorderly construction process. Having posed the central question,let me end the suspense and tell you how the computer programs produced the two configurations.Figure 2 was produced by tossing 169 =(13)2squares down at random onto an area with 35x35 =1225 locations,subject only to the rule that two squares could not fall on the same location.Figure 3 was produced in exactly the same manner except that there was an additional ● rule,namely that two squares could not fall on adjacent lo- cations either.Thus Fig.2 was drawn from the pool of all patterns with 169 squares,while Fig.3 was drawn from the much smaller pool of patterns with 169 squares and with no two squares adjacent.The configuration of Fig.2 is typical of the class with more configurations and hence greater entropy.30 Look again at Fig.3.You will notice that there are no squares immediately adjacent to any given square.This "nearest neighbor exclusion''rule acts to spread out the squares,giving rise to the smooth appearance that tricks so many into guessing that the configuration of Fig.3 is typical of a class with high entropy. Now look again at Fig.2.You will notice holes and Fig.4.An orderly lattice gas configuration that is a member of both the large class and the small class of configurations. clumps of squares,the inhomogeneities that lead many to guess that it is typical of a small class.But in fact one should expect a random configuration to have holes-only a very exceptional configuration is perfectly smooth.31 This in- because this hand is a member of an enormous class of not- particularly-valuable poker hands.But the probability of be- volves the distinction between a typical configuration and an ing dealt this hand is exactly the same as the probability of average configuration.Typical configurations have holes: being dealt the roval flush of hearts.The reason that one some have holes in the upper right,some in the middle left, hand is memorable and the other is not has nothing to do some in the very center.Because the holes fall in various with the rarity of that particular hand;it has everything to do locations,the average configuration-the one produced by with the size of the class of which that hand is a member. adding all the configurations and dividing by the number of This powerful and graphic illustration of the importance of configurations-is smooth.The average configuration is ac- class rather than of individual configuration may be called tually atypical.(Analogy:A typical person is not of average s“the poker paradox.' height.A typical person is somewhat taller or somewhat shorter than average,and very few people are exactly of average height.Any clothing manufacturer that produced V.CONCLUSION only shirts of average size would quickly go bankrupt.)The It is often said that entropy measures the disorder of a presence of holes or clumps,therefore,need not be an indi- system.This qualitative concept has at least three failings: cation of a pattern or of a design.However,we humans tend First,it is vague.There is no precise definition of disorder. to find patterns wherever we look.even when no design is Some find the abstract paintings of Jackson Pollock to be present.In just this way the ancient Greeks looked into the disorderly;others find them pregnant with structure.Second, nighttime sky,with stars sprinkled about at random,and saw the animals,gods,and heroes that became our constellations. it uses an emotionally charged word.Most of us have feel- ings about disorder (either for it or against it),and the anal- ogy encourages us to transfer that like or dislike from disor- der,where our feelings are appropriate,to entropy,where IV.ENTROPY AND POKER they are not.The most important failing,however,is that the An excellent illustration of the nature of entropy is given analogy between entropy and disorder invites us to think about a single configuration rather than a class of configura- by the card game poker.There are many possible hands in poker,some valuable and most less so.For example,the tions.In the lattice gas model there are many "orderly' hand configurations (such as the checkerboard pattern of Fig.4) that are members of both classes.There are many other or- A,K9,Q9,J,10 derly'configurations (such as the solid block pattern of Fig is an example of a royal flush,the most powerful hand in 5)that are members only of the larger (higher entropy!) poker.There are only four royal flushes(the royal flush of class.32.33 The poker hand hearts,of diamonds,of spades,and of clubs)and any poker 2,4◆69,8◆，10* player who has ever been dealt a royal flush will remember it for the rest of his or her life. is very orderly,but a member of a very large class of nearly worthless poker hands. By contrast.no one can remember whether he or she has been dealt the hand Given the clear need for an intuition concerning entropy, and the appealing but unsatisfactory character of the simile 4◆，3◆，J,2◆.7◆ 'entropy as disorder,"what is to be done?I suggest an 1093 Am.J.Phys.,Vol.68,No.12,December 2000 Daniel F.Styer 1093cess for producing that configuration, while the smoothness of the lower configuration suggests a random, disorderly construction process. Having posed the central question, let me end the suspense and tell you how the computer programs produced the two configurations. Figure 2 was produced by tossing 169 5(13)2 squares down at random onto an area with 35335 51225 locations, subject only to the rule that two squares could not fall on the same location. Figure 3 was produced in exactly the same manner except that there was an additional rule, namely that two squares could not fall on adjacent lo￾cations either. Thus Fig. 2 was drawn from the pool of all patterns with 169 squares, while Fig. 3 was drawn from the much smaller pool of patterns with 169 squares and with no two squares adjacent. The configuration of Fig. 2 is typical of the class with more configurations and hence greater entropy.30 Look again at Fig. 3. You will notice that there are no squares immediately adjacent to any given square. This ‘‘nearest neighbor exclusion’’ rule acts to spread out the squares, giving rise to the smooth appearance that tricks so many into guessing that the configuration of Fig. 3 is typical of a class with high entropy. Now look again at Fig. 2. You will notice holes and clumps of squares, the inhomogeneities that lead many to guess that it is typical of a small class. But in fact one should expect a random configuration to have holes—only a very exceptional configuration is perfectly smooth.31 This in￾volves the distinction between a typical configuration and an average configuration. Typical configurations have holes: some have holes in the upper right, some in the middle left, some in the very center. Because the holes fall in various locations, the average configuration—the one produced by adding all the configurations and dividing by the number of configurations—is smooth. The average configuration is ac￾tually atypical. ~Analogy: A typical person is not of average height. A typical person is somewhat taller or somewhat shorter than average, and very few people are exactly of average height. Any clothing manufacturer that produced only shirts of average size would quickly go bankrupt.! The presence of holes or clumps, therefore, need not be an indi￾cation of a pattern or of a design. However, we humans tend to find patterns wherever we look, even when no design is present. In just this way the ancient Greeks looked into the nighttime sky, with stars sprinkled about at random, and saw the animals, gods, and heroes that became our constellations. IV. ENTROPY AND POKER An excellent illustration of the nature of entropy is given by the card game poker. There are many possible hands in poker, some valuable and most less so. For example, the hand Ak,Kk,Qk,Jk,10k is an example of a royal flush, the most powerful hand in poker. There are only four royal flushes ~the royal flush of hearts, of diamonds, of spades, and of clubs! and any poker player who has ever been dealt a royal flush will remember it for the rest of his or her life. By contrast, no one can remember whether he or she has been dealt the hand 4l,3l,Jk,2;,7l because this hand is a member of an enormous class of not￾particularly-valuable poker hands. But the probability of be￾ing dealt this hand is exactly the same as the probability of being dealt the royal flush of hearts. The reason that one hand is memorable and the other is not has nothing to do with the rarity of that particular hand; it has everything to do with the size of the class of which that hand is a member. This powerful and graphic illustration of the importance of class rather than of individual configuration may be called ‘‘the poker paradox.’’ V. CONCLUSION It is often said that entropy measures the disorder of a system. This qualitative concept has at least three failings: First, it is vague. There is no precise definition of disorder. Some find the abstract paintings of Jackson Pollock to be disorderly; others find them pregnant with structure. Second, it uses an emotionally charged word. Most of us have feel￾ings about disorder ~either for it or against it!, and the anal￾ogy encourages us to transfer that like or dislike from disor￾der, where our feelings are appropriate, to entropy, where they are not. The most important failing, however, is that the analogy between entropy and disorder invites us to think about a single configuration rather than a class of configura￾tions. In the lattice gas model there are many ‘‘orderly’’ configurations ~such as the checkerboard pattern of Fig. 4! that are members of both classes. There are many other ‘‘or￾derly’’ configurations ~such as the solid block pattern of Fig. 5! that are members only of the larger ~higher entropy!! class.32,33 The poker hand 2',4l,6k,8;,10' is very orderly, but a member of a very large class of nearly worthless poker hands. Given the clear need for an intuition concerning entropy, and the appealing but unsatisfactory character of the simile ‘‘entropy as disorder,’’ what is to be done? I suggest an Fig. 4. An orderly lattice gas configuration that is a member of both the large class and the small class of configurations. 1093 Am. J. Phys., Vol. 68, No. 12, December 2000 Daniel F. Styer 1093