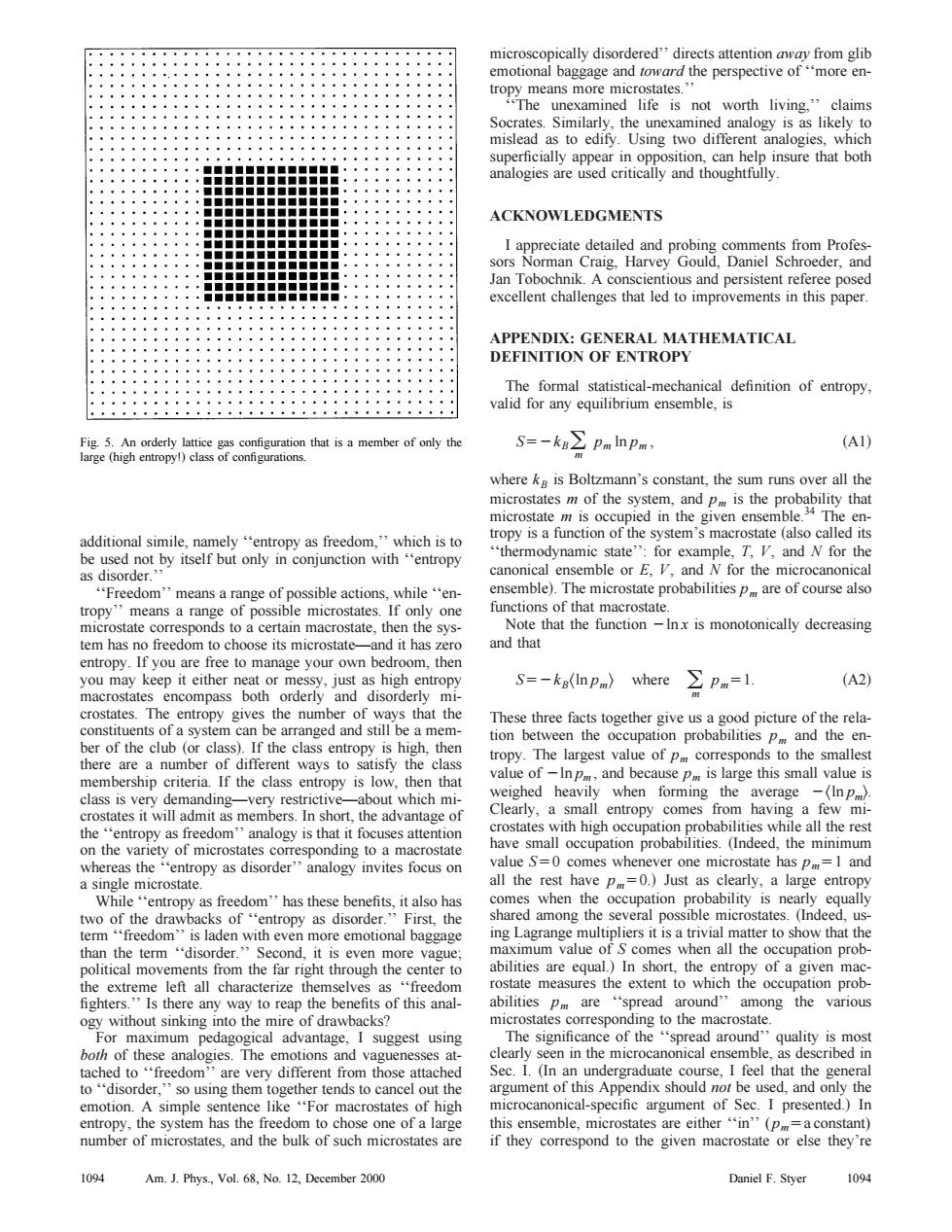
microscopically disordered''directs attention avay from glib emotional baggage and toward the perspective of"more en- tropy means more microstates. "The unexamined life is not worth living,"claims Socrates.Similarly,the unexamined analogy is as likely to mislead as to edify.Using two different analogies.which superficially appear in opposition,can help insure that both ■■■■■■■■■■■■ ■■■■■■■■■■■■■ analogies are used critically and thoughtfully. ■■■■■■■■■■■ ACKNOWLEDGMENTS I appreciate detailed and probing comments from Profes- ■■■ sors Norman Craig,Harvey Gould,Daniel Schroeder,and ■■■■■■■■■■■■ ■■■■■■■■■■■■ Jan Tobochnik.A conscientious and persistent referee posed ■■■ excellent challenges that led to improvements in this paper. APPENDIX:GENERAL MATHEMATICAL DEFINITION OF ENTROPY The formal statistical-mechanical definition of entropy, valid for any equilibrium ensemble,is Fig.5.An orderly lattice gas configuration that is a member of only the S=-kB∑PmInpm, (A1) large (high entropy!)class of configurations. where kg is Boltzmann's constant,the sum runs over all the microstates m of the system,and pm is the probability that microstate m is occupied in the given ensemble.34 The en- additional simile,namely"entropy as freedom,"which is to tropy is a function of the system's macrostate(also called its be used not by itself but only in conjunction with"entropy "thermodynamic state'':for example,T,V,and N for the as disorder.' canonical ensemble or E.V.and N for the microcanonical "Freedom"'means a range of possible actions,while "'en- ensemble).The microstate probabilities p are of course also tropy'means a range of possible microstates.If only one functions of that macrostate microstate corresponds to a certain macrostate,then the sys- Note that the function -Inx is monotonically decreasing tem has no freedom to choose its microstate-and it has zero and that entropy.If you are free to manage your own bedroom,then you may keep it either neat or messy,just as high entropy S=-kB(nPm）where∑Pm=l (A2) macrostates encompass both orderly and disorderly mi- crostates.The entropy gives the number of ways that the These three facts together give us a good picture of the rela- constituents of a system can be arranged and still be a mem- tion between the occupation probabilities pm and the en- ber of the club (or class).If the class entropy is high,then there are a number of different ways to satisfy the class tropy.The largest value of pm corresponds to the smallest membership criteria.If the class entropy is low,then that value of-Inpm,and because p is large this small value is class is very demanding-very restrictive-about which mi- weighed heavily when forming the average -(Inp) crostates it will admit as members.In short,the advantage of Clearly,a small entropy comes from having a few mi- the "'entropy as freedom''analogy is that it focuses attention crostates with high occupation probabilities while all the rest on the variety of microstates corresponding to a macrostate have small occupation probabilities.(Indeed,the minimum whereas the "entropy as disorder''analogy invites focus on value S=0 comes whenever one microstate has pm=1 and a single microstate. all the rest have pm=0.)Just as clearly,a large entropy While"entropy as freedom''has these benefits,it also has comes when the occupation probability is nearly equally two of the drawbacks of "entropy as disorder."First,the shared among the several possible microstates.(Indeed,us- term"freedom''is laden with even more emotional baggage ing Lagrange multipliers it is a trivial matter to show that the than the term "disorder."'Second,it is even more vague; maximum value of S comes when all the occupation prob- political movements from the far right through the center to abilities are equal.)In short,the entropy of a given mac- the extreme left all characterize themselves as "freedom rostate measures the extent to which the occupation prob- fighters."Is there any way to reap the benefits of this anal- abilities pm are "spread around'among the various ogy without sinking into the mire of drawbacks? microstates corresponding to the macrostate. For maximum pedagogical advantage,I suggest using The significance of the "spread around''quality is most both of these analogies.The emotions and vaguenesses at- clearly seen in the microcanonical ensemble,as described in tached to freedom''are very different from those attached Sec.I.(In an undergraduate course,I feel that the general to"'disorder,"so using them together tends to cancel out the argument of this Appendix should not be used,and only the emotion.A simple sentence like "For macrostates of high microcanonical-specific argument of Sec.I presented.)In entropy,the system has the freedom to chose one of a large this ensemble,microstates are either "in'(pm=aconstant) number of microstates,and the bulk of such microstates are if they correspond to the given macrostate or else they're 1094 Am.J.Phys.,Vol.68,No.12,December 2000 Daniel F.Styer 1094additional simile, namely ‘‘entropy as freedom,’’ which is to be used not by itself but only in conjunction with ‘‘entropy as disorder.’’ ‘‘Freedom’’ means a range of possible actions, while ‘‘en￾tropy’’ means a range of possible microstates. If only one microstate corresponds to a certain macrostate, then the sys￾tem has no freedom to choose its microstate—and it has zero entropy. If you are free to manage your own bedroom, then you may keep it either neat or messy, just as high entropy macrostates encompass both orderly and disorderly mi￾crostates. The entropy gives the number of ways that the constituents of a system can be arranged and still be a mem￾ber of the club ~or class!. If the class entropy is high, then there are a number of different ways to satisfy the class membership criteria. If the class entropy is low, then that class is very demanding—very restrictive—about which mi￾crostates it will admit as members. In short, the advantage of the ‘‘entropy as freedom’’ analogy is that it focuses attention on the variety of microstates corresponding to a macrostate whereas the ‘‘entropy as disorder’’ analogy invites focus on a single microstate. While ‘‘entropy as freedom’’ has these benefits, it also has two of the drawbacks of ‘‘entropy as disorder.’’ First, the term ‘‘freedom’’ is laden with even more emotional baggage than the term ‘‘disorder.’’ Second, it is even more vague; political movements from the far right through the center to the extreme left all characterize themselves as ‘‘freedom fighters.’’ Is there any way to reap the benefits of this anal￾ogy without sinking into the mire of drawbacks? For maximum pedagogical advantage, I suggest using both of these analogies. The emotions and vaguenesses at￾tached to ‘‘freedom’’ are very different from those attached to ‘‘disorder,’’ so using them together tends to cancel out the emotion. A simple sentence like ‘‘For macrostates of high entropy, the system has the freedom to chose one of a large number of microstates, and the bulk of such microstates are microscopically disordered’’ directs attention away from glib emotional baggage and toward the perspective of ‘‘more en￾tropy means more microstates.’’ ‘‘The unexamined life is not worth living,’’ claims Socrates. Similarly, the unexamined analogy is as likely to mislead as to edify. Using two different analogies, which superficially appear in opposition, can help insure that both analogies are used critically and thoughtfully. ACKNOWLEDGMENTS I appreciate detailed and probing comments from Profes￾sors Norman Craig, Harvey Gould, Daniel Schroeder, and Jan Tobochnik. A conscientious and persistent referee posed excellent challenges that led to improvements in this paper. APPENDIX: GENERAL MATHEMATICAL DEFINITION OF ENTROPY The formal statistical-mechanical definition of entropy, valid for any equilibrium ensemble, is S52kB( m pm ln pm , ~A1! where kB is Boltzmann’s constant, the sum runs over all the microstates m of the system, and pm is the probability that microstate m is occupied in the given ensemble.34 The en￾tropy is a function of the system’s macrostate ~also called its ‘‘thermodynamic state’’: for example, T, V, and N for the canonical ensemble or E, V, and N for the microcanonical ensemble!. The microstate probabilities pm are of course also functions of that macrostate. Note that the function 2ln x is monotonically decreasing and that S52kB^ln pm& where ( m pm51. ~A2! These three facts together give us a good picture of the rela￾tion between the occupation probabilities pm and the en￾tropy. The largest value of pm corresponds to the smallest value of 2ln pm , and because pm is large this small value is weighed heavily when forming the average 2^ln pm&. Clearly, a small entropy comes from having a few mi￾crostates with high occupation probabilities while all the rest have small occupation probabilities. ~Indeed, the minimum value S50 comes whenever one microstate has pm51 and all the rest have pm50.! Just as clearly, a large entropy comes when the occupation probability is nearly equally shared among the several possible microstates. ~Indeed, us￾ing Lagrange multipliers it is a trivial matter to show that the maximum value of S comes when all the occupation prob￾abilities are equal.! In short, the entropy of a given mac￾rostate measures the extent to which the occupation prob￾abilities pm are ‘‘spread around’’ among the various microstates corresponding to the macrostate. The significance of the ‘‘spread around’’ quality is most clearly seen in the microcanonical ensemble, as described in Sec. I. ~In an undergraduate course, I feel that the general argument of this Appendix should not be used, and only the microcanonical-specific argument of Sec. I presented.! In this ensemble, microstates are either ‘‘in’’ (pm5a constant) if they correspond to the given macrostate or else they’re Fig. 5. An orderly lattice gas configuration that is a member of only the large ~high entropy!! class of configurations. 1094 Am. J. Phys., Vol. 68, No. 12, December 2000 Daniel F. Styer 1094