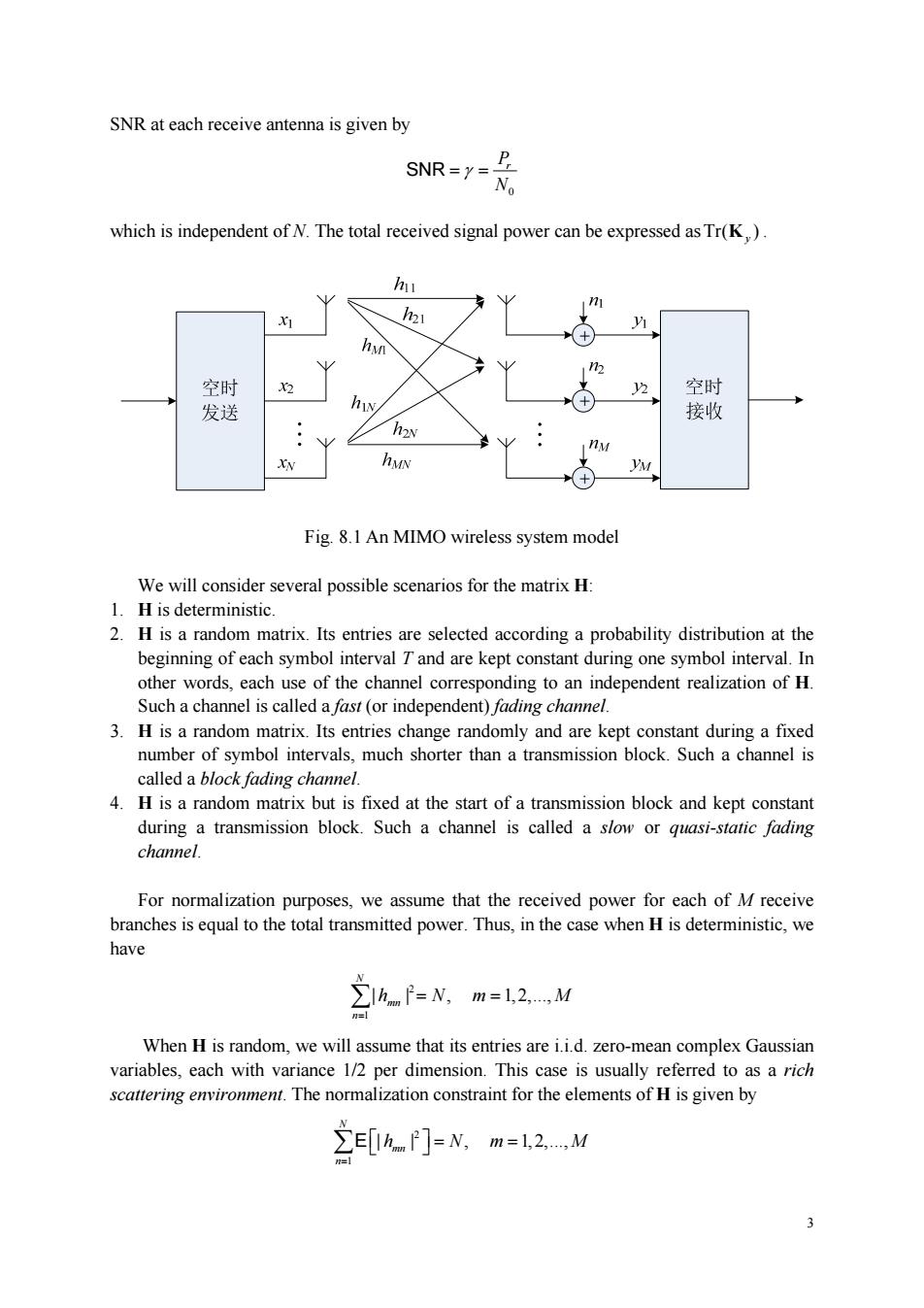
SNR at each receive antenna is given by SNR-7-N. which is independent of N.The total received signal power can be expressed asTr(K) h 7 h 2 空时 ④ 空时 发送 接收 ny Fig.8.1 An MIMO wireless system model We will consider several possible scenarios for the matrix H: 1.H is deterministic 2.H is a random matrix.Its entries are selected according a probability distribution at the beginning of each symbol interval T and are kept constant during one symbol interval.In each of the cha corresp ding to an inc dependent ealization of H. ing channel. 3.H is a random matrix.Its entries change randomly and are kept constant during a fixed number of symbol intervals,much shorter than a transmission block.Such a channel is called a block fading channel. 4.H is a random matrix but is fixed at the start of a transmission block and kept constant Such a channel is called a slow or quasi-static fading channel For normalization purposes,we assume that the received power for each of M receive branches is equal to the total transmitted power.Thus,in the case when H is deterministic,we have F-N.n.2. When H is random,we will assume that its entries are i.i.d.zero-mean complex Gaussian with variance per dimension.This scase is usually referred to as a rich scattering environmen.The normalization constraint for the elements of H is given by 2E[h门=N,m=l2M 3 SNR at each receive antenna is given by 0 Pr N SNR    which is independent of N. The total received signal power can be expressed asTr( ) K y .    Fig. 8.1 An MIMO wireless system model We will consider several possible scenarios for the matrix H: 1. H is deterministic. 2. H is a random matrix. Its entries are selected according a probability distribution at the beginning of each symbol interval T and are kept constant during one symbol interval. In other words, each use of the channel corresponding to an independent realization of H. Such a channel is called a fast (or independent) fading channel. 3. H is a random matrix. Its entries change randomly and are kept constant during a fixed number of symbol intervals, much shorter than a transmission block. Such a channel is called a block fading channel. 4. H is a random matrix but is fixed at the start of a transmission block and kept constant during a transmission block. Such a channel is called a slow or quasi-static fading channel. For normalization purposes, we assume that the received power for each of M receive branches is equal to the total transmitted power. Thus, in the case when H is deterministic, we have 2 1 | | , 1,2,., N mn n h Nm M     When H is random, we will assume that its entries are i.i.d. zero-mean complex Gaussian variables, each with variance 1/2 per dimension. This case is usually referred to as a rich scattering environment. The normalization constraint for the elements of H is given by 2 1 | | , 1,2,., N mn n h Nm M         E