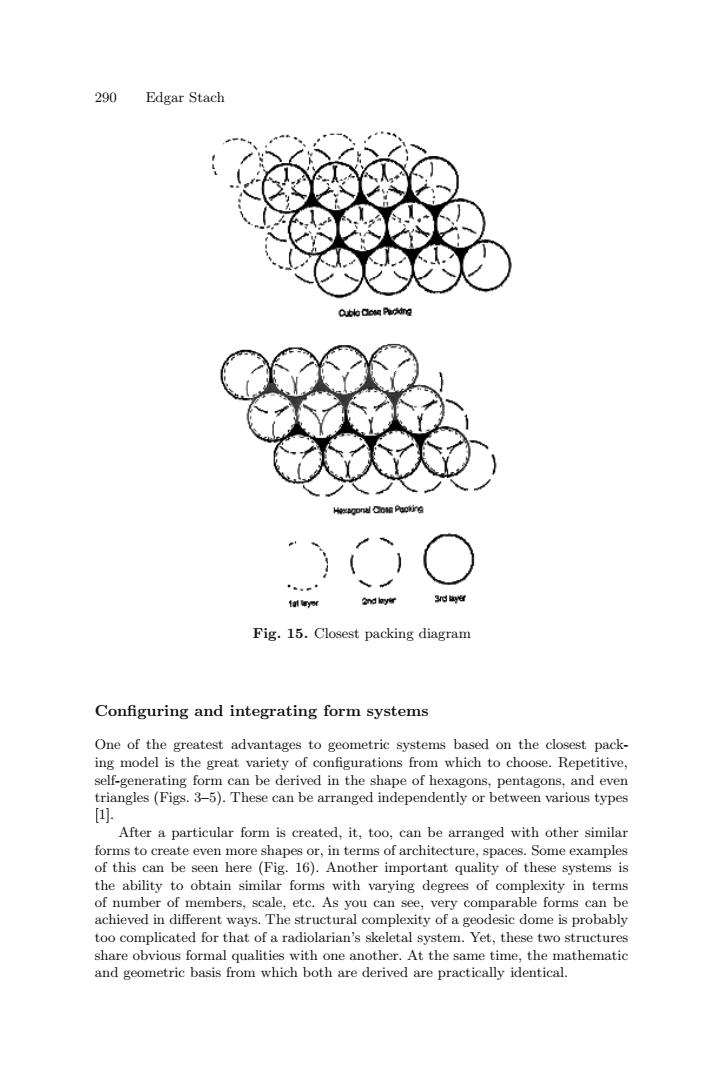
290 Edgar Stach Cublo Cose Puckng Hegonl Coe Paoking fal lyor 3日图 Fig.15.Closest packing diagram Configuring and integrating form systems One of the greatest advantages to geometric systems based on the closest pack- ing model is the great variety of configurations from which to choose.Repetitive, self-generating form can be derived in the shape of hexagons,pentagons,and even triangles (Figs.3-5).These can be arranged independently or between various types [. After a particular form is created,it,too,can be arranged with other similar forms to create even more shapes or,in terms of architecture,spaces.Some examples of this can be seen here (Fig.16).Another important quality of these systems is the ability to obtain similar forms with varying degrees of complexity in terms of number of members,scale,etc.As you can see,very comparable forms can be achieved in different ways.The structural complexity of a geodesic dome is probably too complicated for that of a radiolarian's skeletal system.Yet,these two structures share obvious formal qualities with one another.At the same time,the mathematic and geometric basis from which both are derived are practically identical.290 Edgar Stach Fig. 15. Closest packing diagram Configuring and integrating form systems One of the greatest advantages to geometric systems based on the closest pack￾ing model is the great variety of configurations from which to choose. Repetitive, self-generating form can be derived in the shape of hexagons, pentagons, and even triangles (Figs. 3–5). These can be arranged independently or between various types [1]. After a particular form is created, it, too, can be arranged with other similar forms to create even more shapes or, in terms of architecture, spaces. Some examples of this can be seen here (Fig. 16). Another important quality of these systems is the ability to obtain similar forms with varying degrees of complexity in terms of number of members, scale, etc. As you can see, very comparable forms can be achieved in different ways. The structural complexity of a geodesic dome is probably too complicated for that of a radiolarian’s skeletal system. Yet, these two structures share obvious formal qualities with one another. At the same time, the mathematic and geometric basis from which both are derived are practically identical