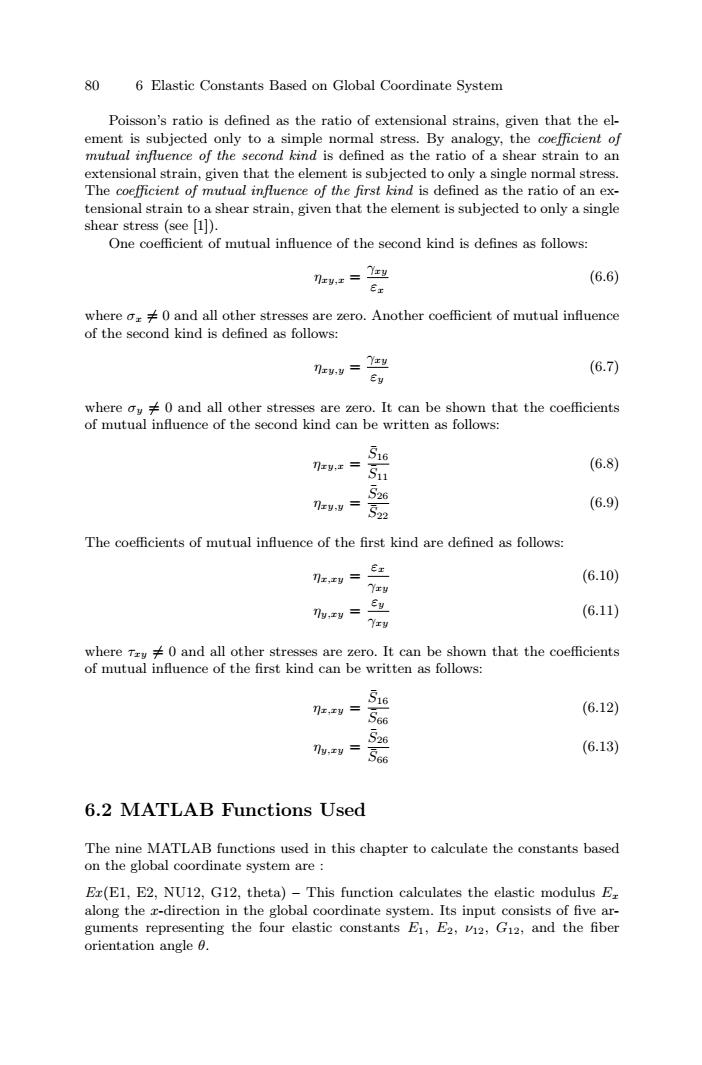
80 6 Elastic Constants Based on Global Coordinate System Poisson's ratio is defined as the ratio of extensional strains,given that the el- ement is subjected only to a simple normal stress.By analogy,the coefficient of mutual influence of the second kind is defined as the ratio of a shear strain to an extensional strain,given that the element is subjected to only a single normal stress. The coefficient of mutual influence of the first kind is defined as the ratio of an ex- tensional strain to a shear strain,given that the element is subjected to only a single shear stress (see 1). One coefficient of mutual influence of the second kind is defines as follows: Ty,Tty (6.6) Ex where o0 and all other stresses are zero.Another coefficient of mutual influence of the second kind is defined as follows: ney.v=Yty (6.7) Ey where oy0 and all other stresses are zero.It can be shown that the coefficients of mutual influence of the second kind can be written as follows: 江，x= 516 (6.8) 526 Tlzy.y= 522 (6.9) The coefficients of mutual influence of the first kind are defined as follows: nt,ty Ea (6.10) Yry Tyty Eu (6.11) Y红w whereT0 and all other stresses are zero.It can be shown that the coefficients of mutual influence of the first kind can be written as follows: nr,ty 516 S66 (6.12) 526 Thy.ty= 56 (6.13) 6.2 MATLAB Functions Used The nine MATLAB functions used in this chapter to calculate the constants based on the global coordinate system are Er(E1,E2,NU12,G12,theta)-This function calculates the elastic modulus E along the r-direction in the global coordinate system.Its input consists of five ar- guments representing the four elastic constants E,E2,v12,G12,and the fiber orientation angle 0.80 6 Elastic Constants Based on Global Coordinate System Poisson’s ratio is defined as the ratio of extensional strains, given that the el￾ement is subjected only to a simple normal stress. By analogy, the coefficient of mutual influence of the second kind is defined as the ratio of a shear strain to an extensional strain, given that the element is subjected to only a single normal stress. The coefficient of mutual influence of the first kind is defined as the ratio of an ex￾tensional strain to a shear strain, given that the element is subjected to only a single shear stress (see [1]). One coefficient of mutual influence of the second kind is defines as follows: ηxy,x = γxy εx (6.6) where σx = 0 and all other stresses are zero. Another coefficient of mutual influence of the second kind is defined as follows: ηxy,y = γxy εy (6.7) where σy = 0 and all other stresses are zero. It can be shown that the coefficients of mutual influence of the second kind can be written as follows: ηxy,x = S¯16 S¯11 (6.8) ηxy,y = S¯26 S¯22 (6.9) The coefficients of mutual influence of the first kind are defined as follows: ηx,xy = εx γxy (6.10) ηy,xy = εy γxy (6.11) where τxy = 0 and all other stresses are zero. It can be shown that the coefficients of mutual influence of the first kind can be written as follows: ηx,xy = S¯16 S¯66 (6.12) ηy,xy = S¯26 S¯66 (6.13) 6.2 MATLAB Functions Used The nine MATLAB functions used in this chapter to calculate the constants based on the global coordinate system are : Ex (E1, E2, NU12, G12, theta) – This function calculates the elastic modulus Ex along the x-direction in the global coordinate system. Its input consists of five ar￾guments representing the four elastic constants E1, E2, ν12, G12, and the fiber orientation angle θ.