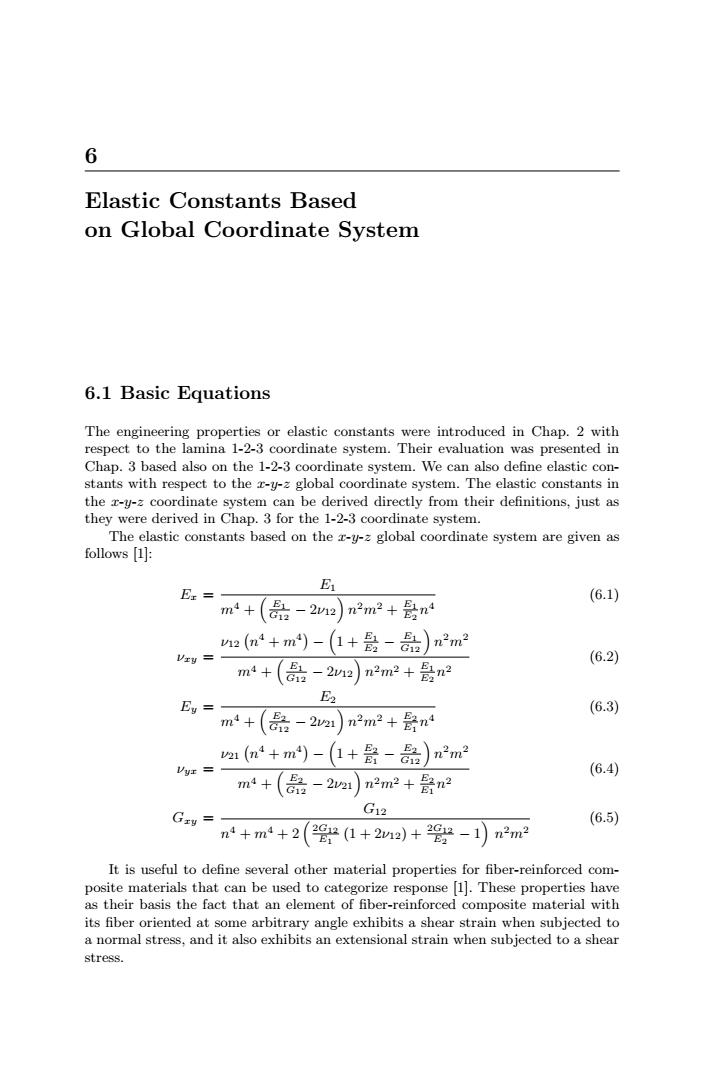
6 Elastic Constants Based on Global Coordinate System 6.1 Basic Equations The engineering properties or elastic constants were introduced in Chap.2 with respect to the lamina 1-2-3 coordinate system.Their evaluation was presented in Chap.3 based also on the 1-2-3 coordinate system.We can also define elastic con- stants with respect to the z-y-z global coordinate system.The elastic constants in the r-y-z coordinate system can be derived directly from their definitions,just as they were derived in Chap.3 for the 1-2-3 coordinate system. The elastic constants based on the r-y-z global coordinate system are given as follows [1]: E Er= (6.1) m+(品-2e）n2m2+n 2(m+m)-（1+悬-品）n2m2 (6.2) m4+(品-2e)n2m2+悬n2 E2 Ey= (6.3) m4+(品-22）n2m2+n4 2(m+m)-（1+景-品)n2m (6.4) m4+(品-22)n2m2+景n2 Gay= G12 (6.5) n4+m4+2(2g(1+212)+2-1)n2m2 B It is useful to define several other material properties for fiber-reinforced com- posite materials that can be used to categorize response [1].These properties have as their basis the fact that an element of fiber-reinforced composite material with its fiber oriented at some arbitrary angle exhibits a shear strain when subjected to a normal stress,and it also exhibits an extensional strain when subjected to a shear stress.6 Elastic Constants Based on Global Coordinate System 6.1 Basic Equations The engineering properties or elastic constants were introduced in Chap. 2 with respect to the lamina 1-2-3 coordinate system. Their evaluation was presented in Chap. 3 based also on the 1-2-3 coordinate system. We can also define elastic con￾stants with respect to the x-y-z global coordinate system. The elastic constants in the x-y-z coordinate system can be derived directly from their definitions, just as they were derived in Chap. 3 for the 1-2-3 coordinate system. The elastic constants based on the x-y-z global coordinate system are given as follows [1]: Ex = E1 m4 +  E1 G12 − 2ν12 n2m2 + E1 E2 n4 (6.1) νxy = ν12  n4 + m4 −  1 + E1 E2 − E1 G12  n2m2 m4 +  E1 G12 − 2ν12 n2m2 + E1 E2 n2 (6.2) Ey = E2 m4 +  E2 G12 − 2ν21 n2m2 + E2 E1 n4 (6.3) νyx = ν21  n4 + m4 −  1 + E2 E1 − E2 G12  n2m2 m4 +  E2 G12 − 2ν21 n2m2 + E2 E1 n2 (6.4) Gxy = G12 n4 + m4 + 2  2G12 E1 (1 + 2ν12) + 2G12 E2 − 1  n2m2 (6.5) It is useful to define several other material properties for fiber-reinforced com￾posite materials that can be used to categorize response [1]. These properties have as their basis the fact that an element of fiber-reinforced composite material with its fiber oriented at some arbitrary angle exhibits a shear strain when subjected to a normal stress, and it also exhibits an extensional strain when subjected to a shear stress