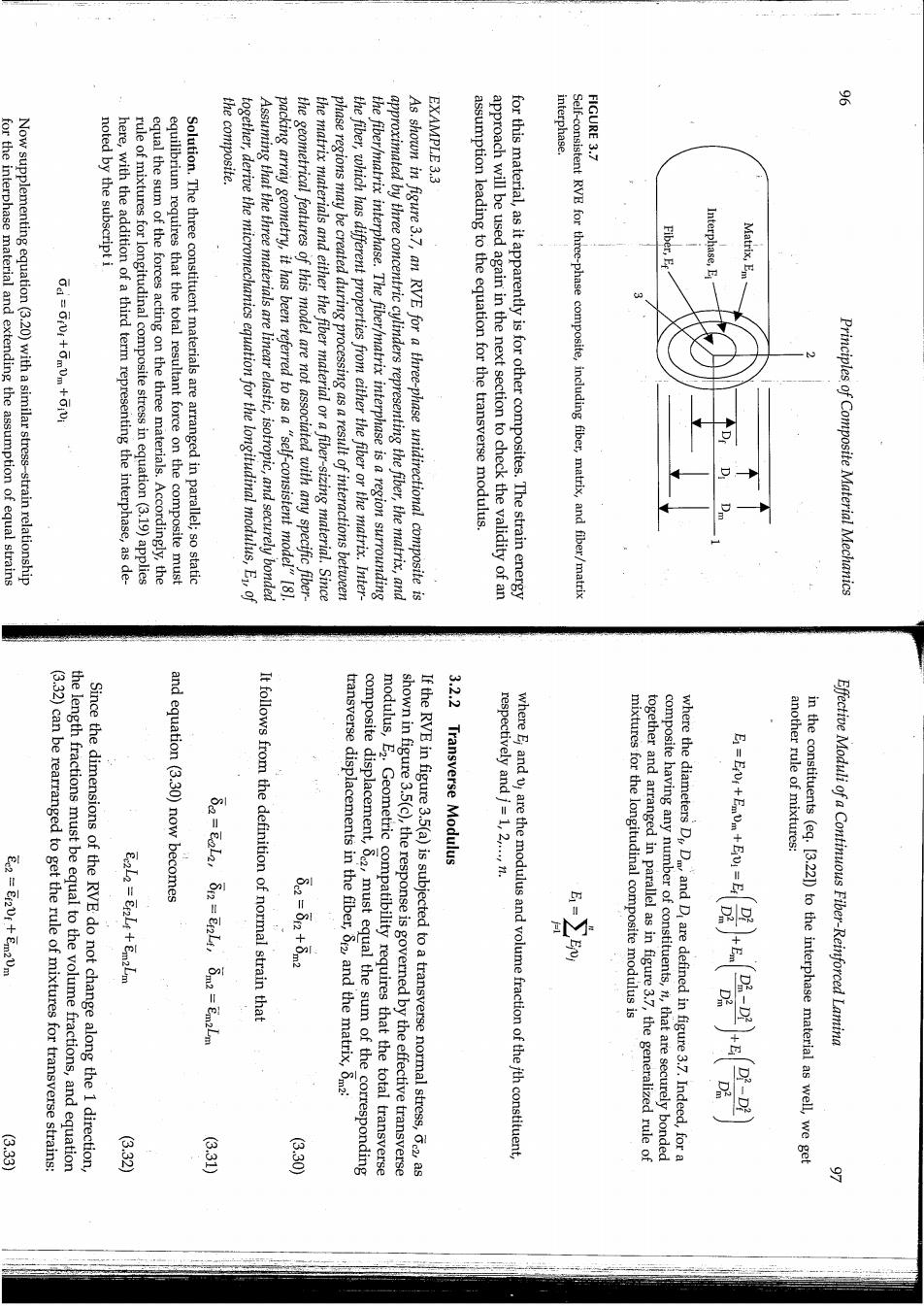
the composite. EXAMPLE 3.3 interphase. FIGURE 3.7 for the interphase material and extending the assumption of equal strains Now supplementing equation(3.20)with a similar stress-strain relationship noted by the subscript i here,with the addition of a third term representing the interphase,as de- rule of mixtures for longitudinal composite stress in equation(3.19)applies equal the sum of the forces acting on the three materials.Accordingly,the equilibrium requires that the total resultant force on the composite must Solution.The three constituent materials are arranged in parallel;so static together,derive the micromechanics equation for the longitudinal modulus,Eof Assuming that the three materials are linear elastic,isotropic,and securely bonded packing array geometry,it has been referred to as a "self-consistent model"[8] the geometrical features of this model are not associated with any specific fiber- the matrix materials and either the fiber material or a fiber-sizing material.Since phase regions may be created during processing as a result of interactions between the fiber,which has different properties from either the fiber or the matrix.Inter- the fiber/matrix interphase.The fiber/matrix interphase is a region surrounding approximated by three concentric cylinders representing the fiber,the matrix,and As shown in figure 3.7,an RVE for a three-phase unidirectional composite is assumption leading to the equation for the transverse modulus. approach will be used again in the next section to check the validity of an for this material,as it apparently is for other composites.The strain energy Self-consistent RVE for three-phase composite,including fiber,matrix,and fiber/matrix Fiber,Ee Interphase,E Matrix,Em Ga=GrVr+GmUm+Givi Principles,of Composite Material Mechanics 83-8217+2m2um (3.32)can be rearranged to get the rule of mixtures for transverse strains: the length fractions must be equal to the volume fractions,and equation Since the dimensions of the RVE do not change along the 1 direction, and equation(3.30)now becomes 83-01839+2mam It follows from the definition of normal strain that transverse displacements in the fiber,6r2,and the matrix,8m2: composite displacement,82,must equal the sum of the corresponding modulus,E2.Geometric compatibility requires that the total transverse shown in figure 3.5(c),the response is governed by the effective transverse If the RVE in figure 3.5(a)is subjected to a transverse normal stress,2,as 3.2.2 Transverse Modulus respectively and j=1,2,....n. where E and v;are the modulus and volume fraction of the jth constituent, mixtures for the longitudinal composite modulus is together and arranged in parallel as in figure 3.7,the generalized rule of where the diameters DDand D are defined in figure 3.7.Indeed,for a composite having any number of constituents,n,that are securely bonded in the constituents(eq.[3.221)to the interphase material as well,we get another rule of mixtures: Effective Moduli of a Continuous Fiber-Reinforced Lamina 3.33 8·32 830