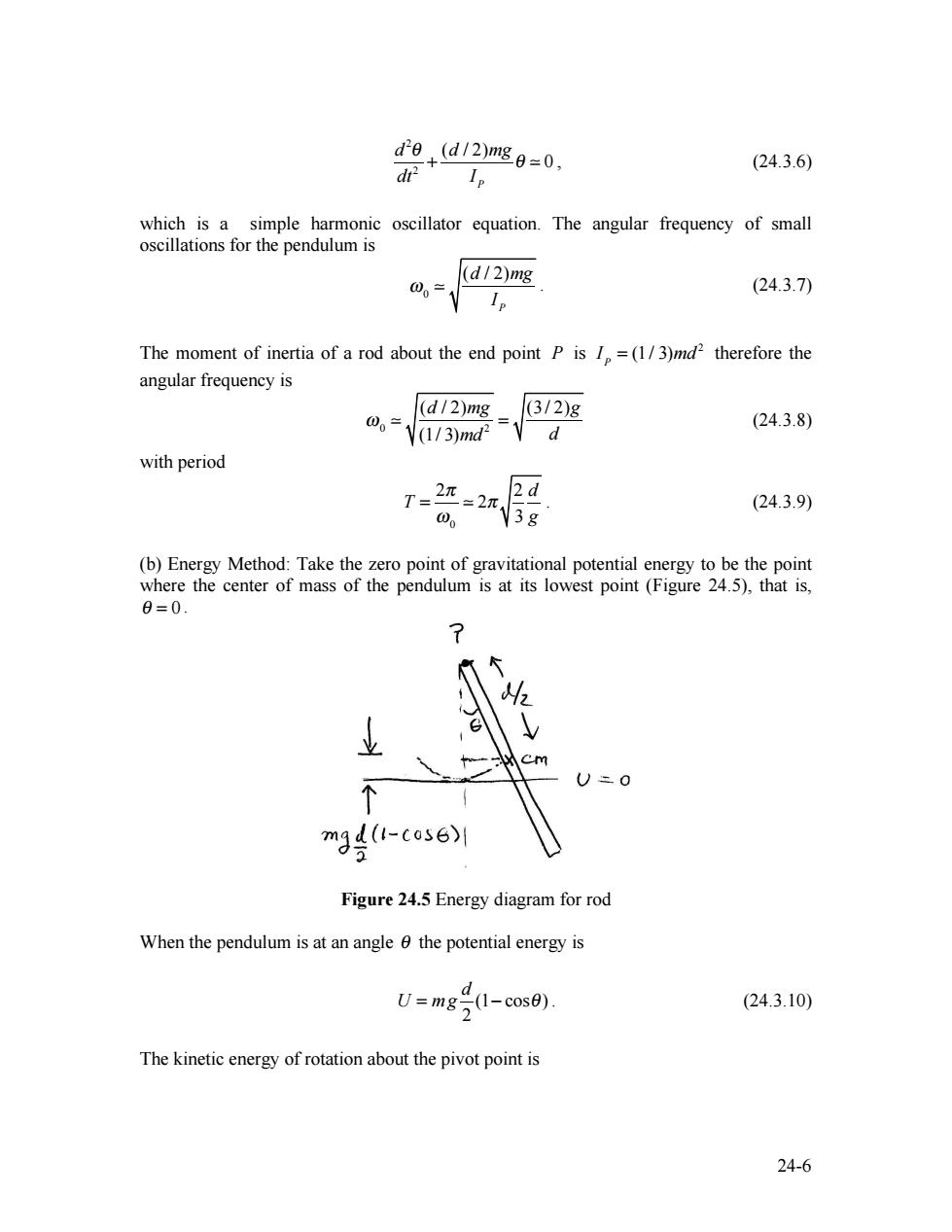
d9,(d/2)mg0=0, 3+ (24.3.6) Ip which is a simple harmonic oscillator equation.The angular frequency of small oscillations for the pendulum is (d/2)mg 00= (24.3.7) Ip The moment of inertia of a rod about the end point P is I=(1/3)md2 therefore the angular frequency is (d/2)mg (312)8 00 (24.3.8) V(1/3)md2 d with period 2d (24.3.9) 0。 (b)Energy Method:Take the zero point of gravitational potential energy to be the point where the center of mass of the pendulum is at its lowest point (Figure 24.5),that is, 0=0. 个 Cm 个 m9(4-co56)1 Figure 24.5 Energy diagram for rod When the pendulum is at an angle 0 the potential energy is U=mg(1-c0s0). (24.3.10) 2 The kinetic energy of rotation about the pivot point is 24-624-6 d2 θ dt 2 + (d / 2)mg IP θ  0 , (24.3.6) which is a simple harmonic oscillator equation. The angular frequency of small oscillations for the pendulum is ω0  (d / 2)mg IP . (24.3.7) The moment of inertia of a rod about the end point P is IP = (1/ 3)md2 therefore the angular frequency is ω0  (d / 2)mg (1/ 3)md2 = (3/ 2)g d (24.3.8) with period T = 2π ω0  2π 2 3 d g . (24.3.9) (b) Energy Method: Take the zero point of gravitational potential energy to be the point where the center of mass of the pendulum is at its lowest point (Figure 24.5), that is, θ = 0 . Figure 24.5 Energy diagram for rod When the pendulum is at an angle θ the potential energy is U = mg d 2 (1− cosθ). (24.3.10) The kinetic energy of rotation about the pivot point is