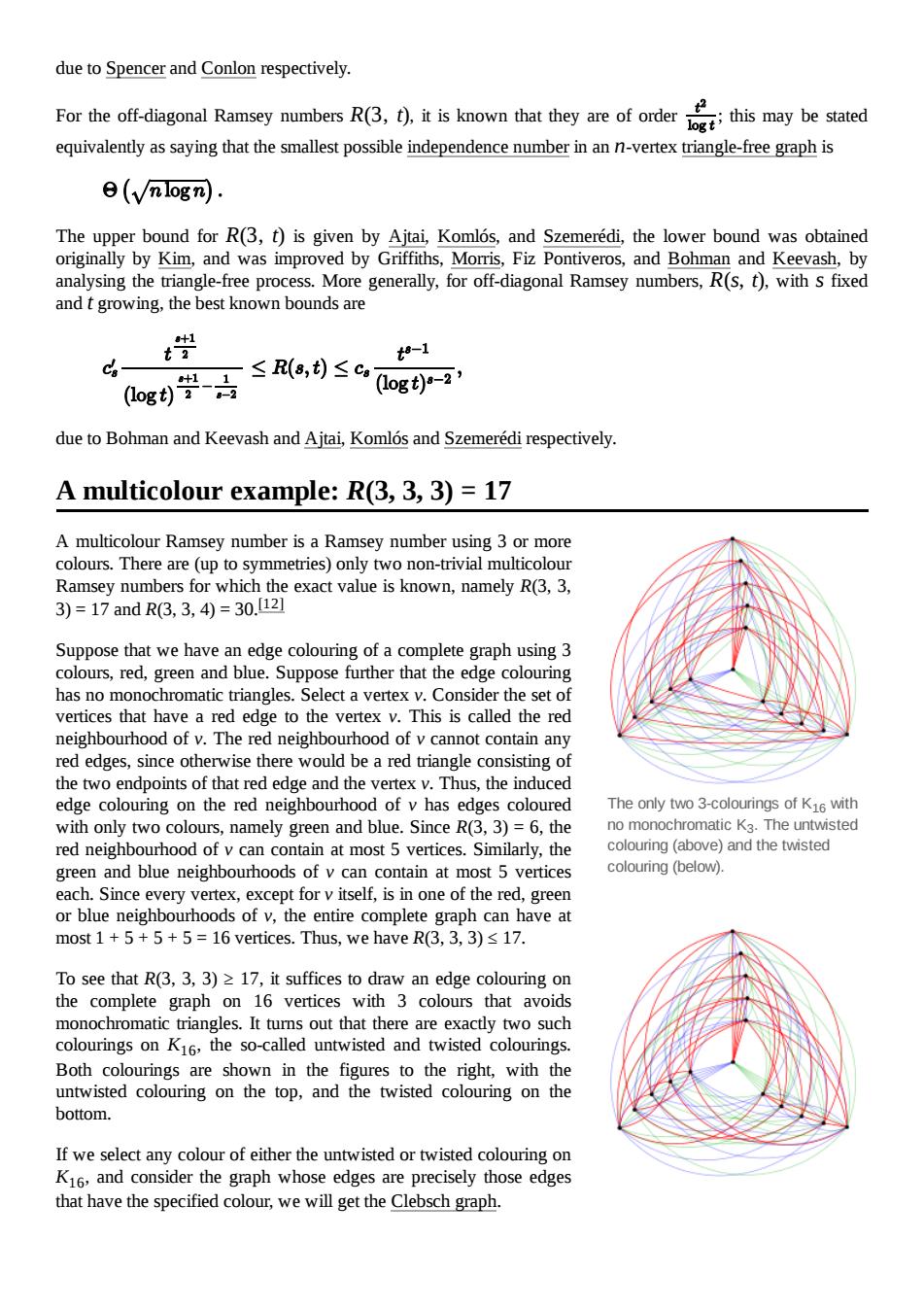
due to Spencer and Conlon respectively. For the off-diagonal Ramsey numbers R(3,t),it is known that they are of order this may be stated equivalently as saying that the smallest possible independence number in an n-vertex triangle-free graph is 日（√mogn The upper bound for R(3,t)is given by Ajtai,Komlos,and Szemeredi,the lower bound was obtained originally by Kim,and was improved by Griffiths,Morris,Fiz Pontiveros,and Bohman and Keevash,by analysing the triangle-free process.More generally,for off-diagonal Ramsey numbers,R(s,t),with s fixed and t growing,the best known bounds are og)尝-点 ≤(a,)≤c, (logt)*-2' due to Bohman and Keevash and Ajtai,Komlos and Szemeredi respectively. A multicolour example:R(3,3,3)=17 multicolour Ramsey number is a Ramsey number using 3 or more colours.There are (up to symmetries)only two non-trivial multicolou amsey numbers I whic exact value is known,namely R(3,3 3)=17andR3,3,9=30.2 0a reen d blu ect a This is ghbourhood of y.Ther od of v ld be a re ed tr al ints of tha a d of The only two 3-colourings of K,with nd bh no monochromatic K3.The untwisted red neighbo ost 5 v colouring(above)and the twisted nd blue ghbo. oods o colouring (below). h st fo t: ep most 1+5+5+5=16 vertices.Thus,we have R(3.3.3)17. To see that R(3,3,3)>17,it suffices to draw an edge colouring on the complete graph on 16 vertices with 3 colours that avoids monochromatic triangles.It turns out that there are exactly two such colourings on K16.the so-called untwisted and twisted colourings. Both colourings are shown in the figures to the right,with the untwisted colouring on the top,and the twisted colouring on the bottom. If we select any colour of either the untwisted or twisted colouring on K16.and consider the graph whose edges are precisely those edges that have the specified colour,we will get the Clebsch graph. The only two 3-colourings of K16 with no monochromatic K3 . The untwisted colouring (above) and the twisted colouring (below). due to Spencer and Conlon respectively. For the off-diagonal Ramsey numbers R(3, t), it is known that they are of order ; this may be stated equivalently as saying that the smallest possible independence number in an n-vertex triangle-free graph is The upper bound for R(3, t) is given by Ajtai, Komlós, and Szemerédi, the lower bound was obtained originally by Kim, and was improved by Griffiths, Morris, Fiz Pontiveros, and Bohman and Keevash, by analysing the triangle-free process. More generally, for off-diagonal Ramsey numbers, R(s, t), with s fixed and t growing, the best known bounds are due to Bohman and Keevash and Ajtai, Komlós and Szemerédi respectively. A multicolour Ramsey number is a Ramsey number using 3 or more colours. There are (up to symmetries) only two non-trivial multicolour Ramsey numbers for which the exact value is known, namely R(3, 3, 3) = 17 and R(3, 3, 4) = 30.[12] Suppose that we have an edge colouring of a complete graph using 3 colours, red, green and blue. Suppose further that the edge colouring has no monochromatic triangles. Select a vertex v. Consider the set of vertices that have a red edge to the vertex v. This is called the red neighbourhood of v. The red neighbourhood of v cannot contain any red edges, since otherwise there would be a red triangle consisting of the two endpoints of that red edge and the vertex v. Thus, the induced edge colouring on the red neighbourhood of v has edges coloured with only two colours, namely green and blue. Since R(3, 3) = 6, the red neighbourhood of v can contain at most 5 vertices. Similarly, the green and blue neighbourhoods of v can contain at most 5 vertices each. Since every vertex, except for v itself, is in one of the red, green or blue neighbourhoods of v, the entire complete graph can have at most 1 + 5 + 5 + 5 = 16 vertices. Thus, we have R(3, 3, 3) ≤ 17. To see that R(3, 3, 3) ≥ 17, it suffices to draw an edge colouring on the complete graph on 16 vertices with 3 colours that avoids monochromatic triangles. It turns out that there are exactly two such colourings on K16 , the so-called untwisted and twisted colourings. Both colourings are shown in the figures to the right, with the untwisted colouring on the top, and the twisted colouring on the bottom. If we select any colour of either the untwisted or twisted colouring on K16 , and consider the graph whose edges are precisely those edges that have the specified colour, we will get the Clebsch graph. A multicolour example: R(3, 3, 3) = 17