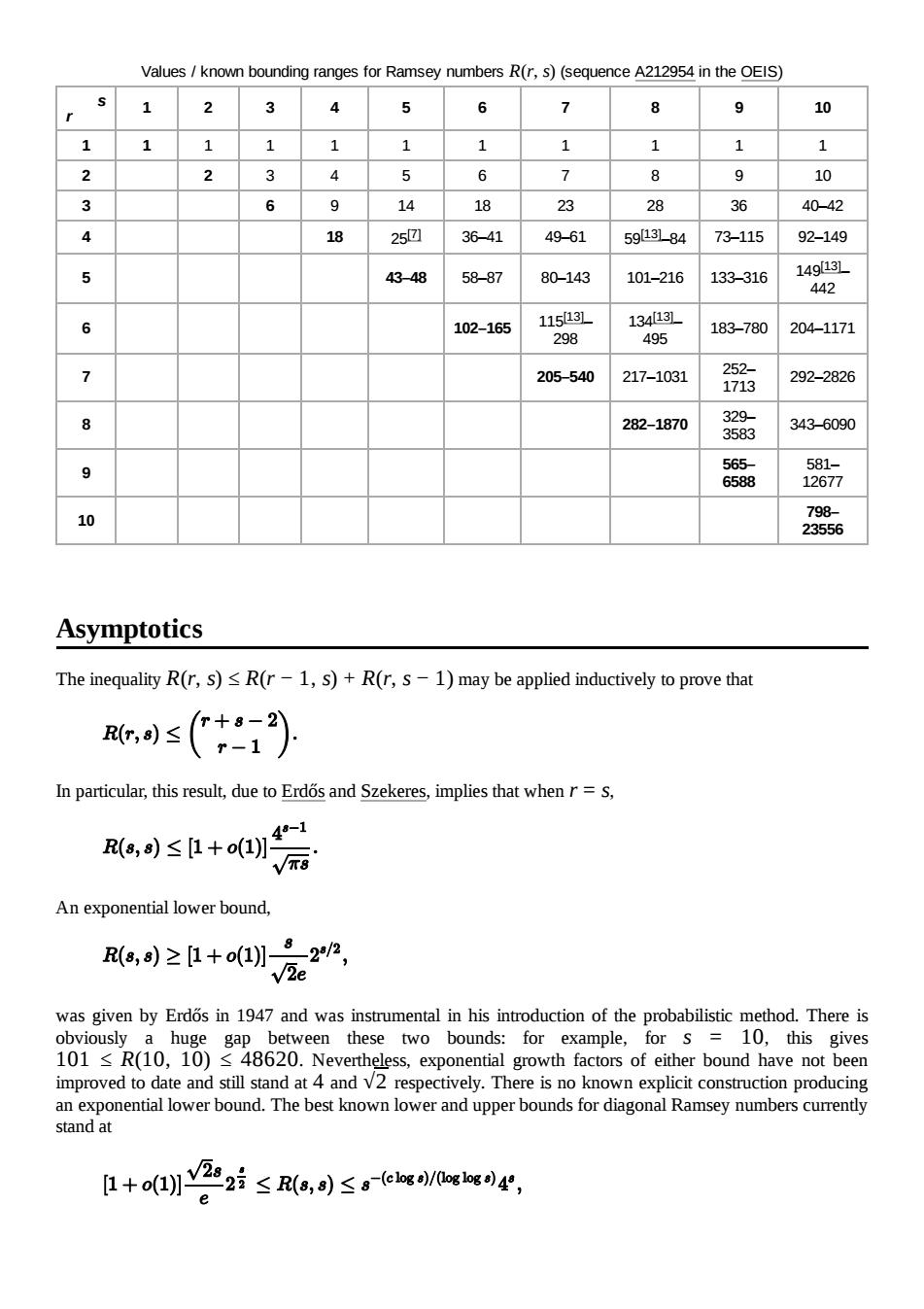
Values/known bounding ranges for Ramsey numbers R(r,s)(sequence A212954 in the OEIS) s 1 4 5 7 8 9 10 1 1 1 1 1 1 1 1 1 1 2 3 4 5 6 8 9 10 3 6 9 14 18 23 28 36 40-42 4 18 250 36-41 49-61 5913194 73-115 92-149 43-48 5887 80-143 101216 133-316 14g[13 442 6 102-165 183-780 204-1171 205-540 217-1031 252 7 292-2826 1713 282-1870 3583 3436090 10 Asymptotics The inequality R(r,s)sR(r-1,s)+R(r,s-1)may be applied inductively to prove that s(中2） In particular,this result,due to Erdos and Szekeres,implies that whenr=s, B)s卫+oa √⑧ An exponential lower bound, R(8,8)≥[1+o(1)月 method.There is given by rds in1947 d wasnsnimen热of he pob如 01Rd0,16248620 thes ample, everth ial either bound improved to e and still There is y icit constc weo的aa通bos的R网s 1+oYy22i≤R6,≤geg/ser, eValues / known bounding ranges for Ramsey numbers R(r, s) (sequence A212954 in the OEIS) s r 1 2 3 4 5 6 7 8 9 10 1 1 1 1 1 1 1 1 1 1 1 2 2 3 4 5 6 7 8 9 10 3 6 9 14 18 23 28 36 40–42 4 18 25 [7] 36–41 49–61 59 [13]–84 73–115 92–149 5 43–48 58–87 80–143 101–216 133–316 149 [13]– 442 6 102–165 115 [13]– 298 134 [13]– 495 183–780 204–1171 7 205–540 217–1031 252– 1713 292–2826 8 282–1870 329– 3583 343–6090 9 565– 6588 581– 12677 10 798– 23556 The inequality R(r, s) ≤ R(r − 1, s) + R(r, s − 1) may be applied inductively to prove that In particular, this result, due to Erdős and Szekeres, implies that when r = s, An exponential lower bound, was given by Erdős in 1947 and was instrumental in his introduction of the probabilistic method. There is obviously a huge gap between these two bounds: for example, for s = 10, this gives 101 ≤ R(10, 10) ≤ 48620. Nevertheless, exponential growth factors of either bound have not been improved to date and still stand at 4 and √2 respectively. There is no known explicit construction producing an exponential lower bound. The best known lower and upper bounds for diagonal Ramsey numbers currently stand at Asymptotics