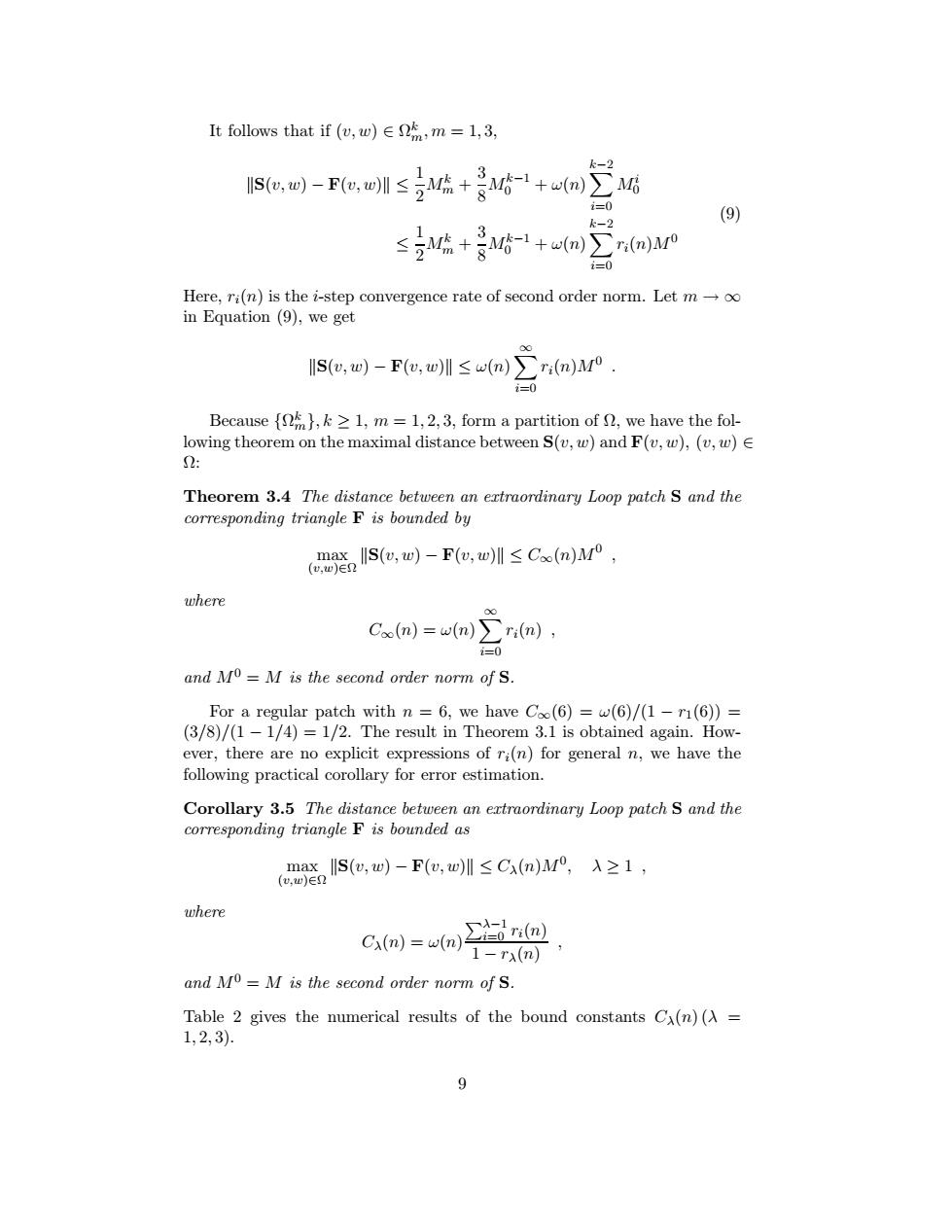
It follows that if (v.w)m=1.3. 5u-I≤+gg+fm2好 Ise,回)-Ft,ol≤e62nmla 时。 Se,)-F,l≤x(njA ahere ca=a2m。 yr Cm5rgonayepadsad IS(,叫)-F,w训≤C4,A≥1 o=M is the secored order zortn of s. It follows that if (v, w) ∈ Ω k m, m = 1, 3, kS(v, w) − F(v, w)k ≤ 1 2 Mk m + 3 8 Mk−1 0 + ω(n) X k−2 i=0 Mi 0 ≤ 1 2 Mk m + 3 8 Mk−1 0 + ω(n) X k−2 i=0 ri(n)M0 (9) Here, ri(n) is the i-step convergence rate of second order norm. Let m → ∞ in Equation (9), we get kS(v, w) − F(v, w)k ≤ ω(n) X∞ i=0 ri(n)M0 . Because {Ω k m}, k ≥ 1, m = 1, 2, 3, form a partition of Ω, we have the fol￾lowing theorem on the maximal distance between S(v, w) and F(v, w), (v, w) ∈ Ω: Theorem 3.4 The distance between an extraordinary Loop patch S and the corresponding triangle F is bounded by max (v,w)∈Ω kS(v, w) − F(v, w)k ≤ C∞(n)M0 , where C∞(n) = ω(n) X∞ i=0 ri(n) , and M0 = M is the second order norm of S. For a regular patch with n = 6, we have C∞(6) = ω(6)/(1 − r1(6)) = (3/8)/(1 − 1/4) = 1/2. The result in Theorem 3.1 is obtained again. How￾ever, there are no explicit expressions of ri(n) for general n, we have the following practical corollary for error estimation. Corollary 3.5 The distance between an extraordinary Loop patch S and the corresponding triangle F is bounded as max (v,w)∈Ω kS(v, w) − F(v, w)k ≤ Cλ(n)M0 , λ ≥ 1 , where Cλ(n) = ω(n) Pλ−1 i=0 ri(n) 1 − rλ(n) , and M0 = M is the second order norm of S. Table 2 gives the numerical results of the bound constants Cλ(n) (λ = 1, 2, 3). 9