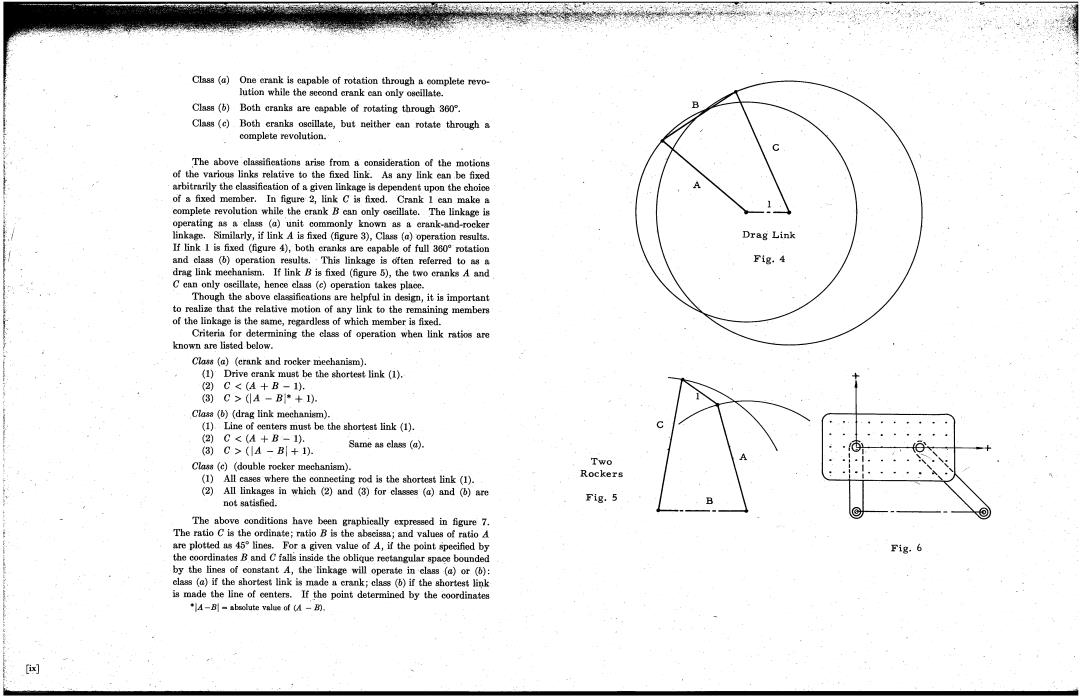
Class (a)One erank is eapable of rotation through a complete revo- lution while the second crank can only oseillate. Class (b)Both cranks are capable of rotating through 360. Class (e)Both cranks oscillate,but neither can rotate through a complete revolution. The above classifieations arise from a consideration of the motions of the various links relative to the fixed link.As any link ean be fixed arbitrarily the classification of a given linkage is dependent upon the choice of s fixed member.In figure 2,link C is fixed.Crank I ean make a complete revolution while the erank B can only oscillate.The linkage is operating as a class (a)unit commonly known as a erank-and-rocker linkage.Similarly,if link A is fixed (fgure 3),Class (a)operation results. Drag Link If link I is fixed (figure 4),both eranks are capable of full 360 rotation and class (b)operation results.This linkage is often referred to as Fig.4 drag link mechanism.If link B is fixed (figure 5),the two eranks A and C ean only oscillate,hence class (c)operation takes plaoe Though the above classifications are helpful in design,it is important to realize that the relative motion of any link to the remaining members of the linkage is the same,regardless of which member is fixed. Criteria for determining the class of operation when link ratios are known are listed below. Class (a)(crank and rocker mechanism). (1)Drive crank must be the shortest link (1). (2)C<(A+B-1). ()C>A-B1+1). Ciasa ()(drag link mechanism). (1)Line of eenters must be the shortest link (1). 2②)C<(A+B-1). (3)C>(A-B+1) Same as class (a). Ciass (e)(double rocker mechaniam). Two (1)All cases where the conneeting rod is the shortest link (1). Rockers (2)All linkages in which (2)and (3)for classes (a)and (b)are not satisfied. Fig.5 The above conditions have been graphically expressed in figure 7. The ratio C is the ordinate;ratio B is the abscissa;and values of ratio A are plotted as 45 lines.For a given value of A,if the point specifiod by Fig.6 the coordinates B and C ialls inside the oblique reetangular space bounded by the lines of constant A,the linkage will operate in class (a)or (b): class (a)if the shortest link is made a crank;class (b)if the shortest link is made the line of centers.If the point determined by the coordinates 4-B]sbsolute value of (A -B). [Class (a) One crank is capable of rotation through a complete revo￾lution while the second crank can only oscillate. Both cranks are capable of rotating through 3600 Both cranks oscillate, but neither can rotate through a complete revolution. ' Class (b) Class (c) 1: ' t c ~he above classifications arise from a consideration of the motions of the variolls links relative to the fixed link. As any link can be fixed , arbitrarily the classification of a given linkage is dependent upon the choice of a fixed member. In figure 2, link is fixed. Crank 1 can make a complete revolution while the crank can only oscillate. The linkage operating as a class (a) unit commonly known as a crank-and-rocker linkage, Similarly, if link A is fixed (figure 3), Class (a) operation results, If link 1 is fixed (figure 4), both cranks are capable of full 3600 rotation and class (b) operation results, ' This linkage is often referred to as a drag link mechanism, If link is fixed (figure 5), the two cranks A and can only oscillate, hence class (c) operation takes place. Though the above clas.sifications are helpful in design, it is important to realize that the relative. motion of any link to the remaining members of the linkage is the same, regardless of which member is fixed. Criteria for determining the class of operation when link ratios are known are listed below. Class (a) (crank and rocker mechanism), (1) Drive crank mustbe the shortest link (1). (2) C.c (A 1). (3) C;:. (IA BI* +1). Class (b) (drag link mechanism), (1), Line of centers must be the shortest link (1). (2) C.c (A B- 1). " (3) C;:. (IA BI + I), Same as class (a). Class (c) (double rocker mechanism). (1) All cases where the connecting rod is the shortest link (1). (2) All linkages in ~hich (2) and (3) for classes (a) and (b) are not satisfied; The above conditions have been graphically expressed in figure 7. The ratio is the ordinate; ratio is the abscissa; and values of ratio A are plotted as 450 lines, For a given value of A , if the point specified by the coordinates Band falls inside the oblique rectangular spa~e bounded by the lines of constant A, the ' linkage will operate in ' class (a) or (b): class (a) if the shortest link is made a crank; class (b) if the shortest liI~lk is made the line of centers, If Jhe point determined by the coordinates IA- BI = absolute value of (A B). Two Rockers Fig, / . --- (ixJ . . . ' . .,......... , " :.. . . , Fig. 6