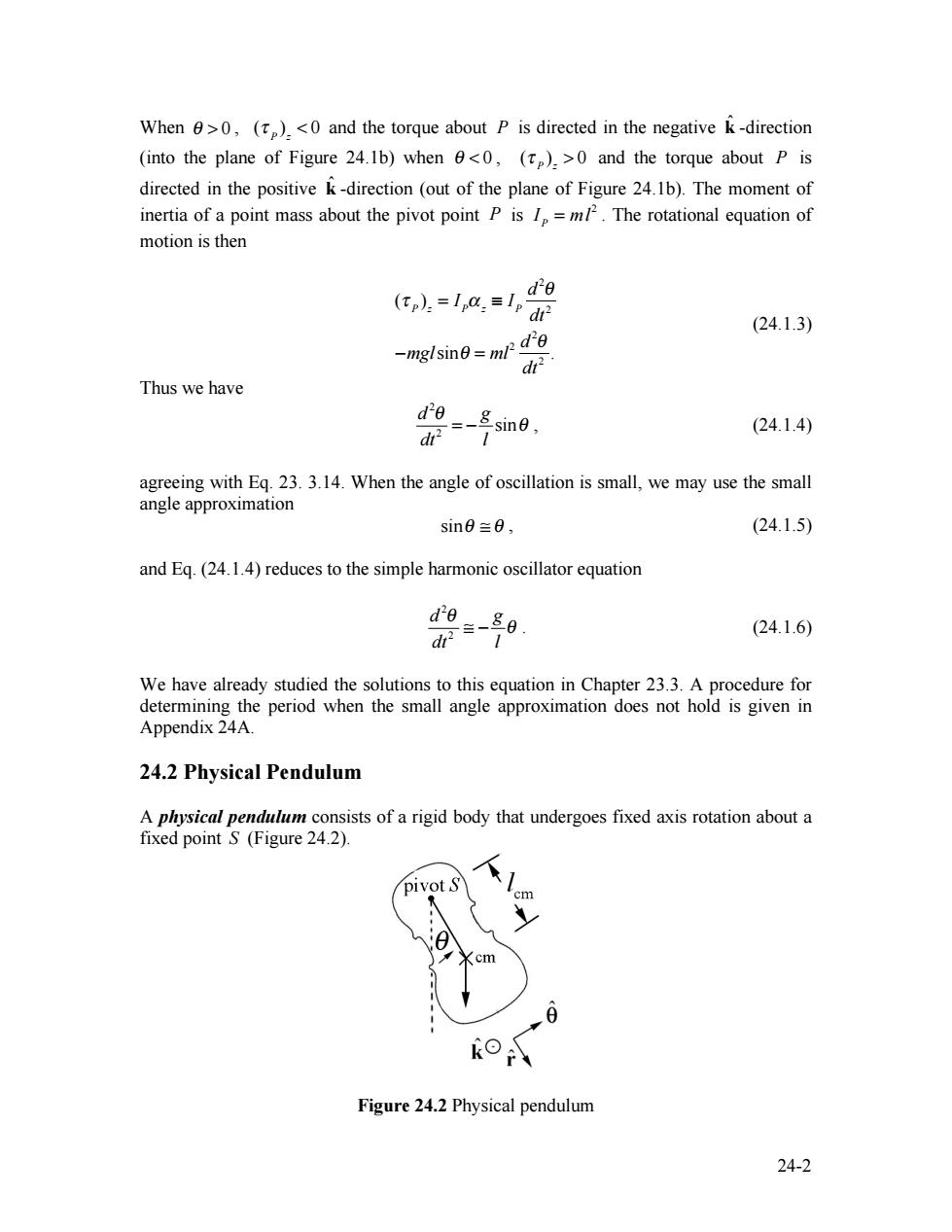
When >0,(and the torque about P is directed in the negative k-direction (into the plane of Figure 24.1b)when <0,()>0 and the torque about P is directed in the positive k-direction(out of the plane of Figure 24.1b).The moment of inertia of a point mass about the pivot point P is I=m12.The rotational equation of motion is then ,l.=1a,=l, do (24.1.3) -mglsine ml2 de dr Thus we have do=_Esine, (24.1.4) agreeing with Eq.23.3.14.When the angle of oscillation is small,we may use the small angle approximation sin0三0， (24.1.5) and Eq.(24.1.4)reduces to the simple harmonic oscillator equation d' dt2 -86 1 (24.1.6) We have already studied the solutions to this equation in Chapter 23.3.A procedure for determining the period when the small angle approximation does not hold is given in Appendix 24A. 24.2 Physical Pendulum A physical pendulum consists of a rigid body that undergoes fixed axis rotation about a fixed point S (Figure 24.2). pivot S cm <cm Figure 24.2 Physical pendulum 24-224-2 When θ > 0 , (τ P )z < 0 and the torque about P is directed in the negative kˆ -direction (into the plane of Figure 24.1b) when θ < 0 , (τ P )z > 0 and the torque about P is directed in the positive kˆ -direction (out of the plane of Figure 24.1b). The moment of inertia of a point mass about the pivot point P is IP = ml 2 . The rotational equation of motion is then (τ P )z = IPα z ≡ IP d2 θ dt 2 −mglsinθ = ml 2 d2 θ dt 2 . (24.1.3) Thus we have 2 2 sin d g dt l θ = − θ , (24.1.4) agreeing with Eq. 23. 3.14. When the angle of oscillation is small, we may use the small angle approximation sinθ ≅ θ , (24.1.5) and Eq. (24.1.4) reduces to the simple harmonic oscillator equation 2 2 d g dt l θ ≅ − θ . (24.1.6) We have already studied the solutions to this equation in Chapter 23.3. A procedure for determining the period when the small angle approximation does not hold is given in Appendix 24A. 24.2 Physical Pendulum A physical pendulum consists of a rigid body that undergoes fixed axis rotation about a fixed point S (Figure 24.2). Figure 24.2 Physical pendulum