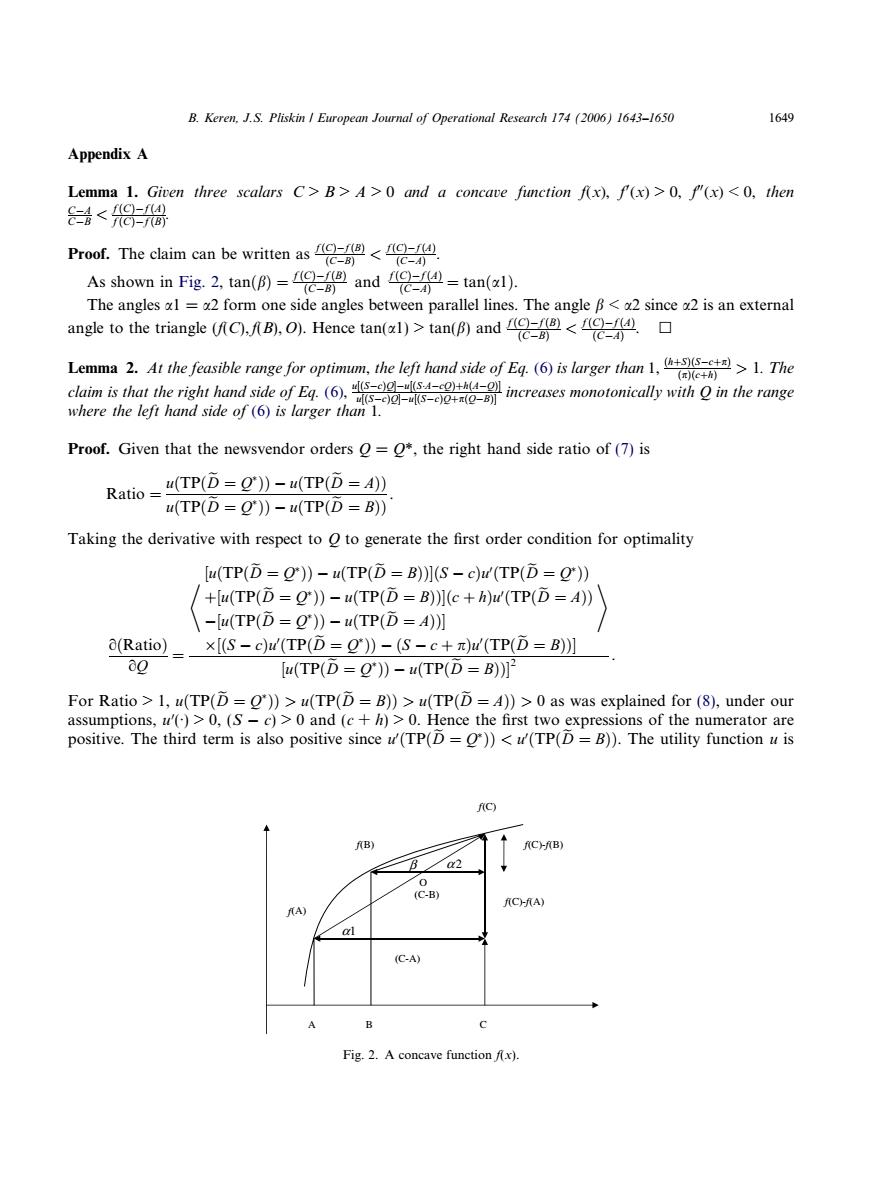
B.Keren.J.S.Pliskin European Journal of Operational Research 174 (2006)1643-1650 1649 Appendix A Lemma 1.Given three scalars C>B>A>0 and a concave function fx),f(x)>0,f"(x)<0,then 特<得 Proof.The claim can be written as (C-B) (C-A) As shown in Fig.2.tan(B)=tc)and tc()=tan(a). (C-B) (C-A) The angles al=a2 form one side angles between parallel lines.The angle B<a2 since a2 is an external angle to the triangle (C).B),O).Hence tan(al)>tan(B)and (c<(4) (C-B) (C-A) Lemma 2.At the feasible range for optimum,the left hand side of Eq.(6)is larger than1.1.The ()(c+h) claim is that the right hand side of Eq.(6).increases monotonically with Q in the range S-cg-S-c0+0-B町 where the left hand side of(6)is larger than 1. Proof.Given that the newsvendor orders o=O*,the right hand side ratio of(7)is Ratio= u(TP(D=))-u(TP(D =A)) u(TP(D=O))-u(TP(D B)) Taking the derivative with respect to O to generate the first order condition for optimality [u(TP(D=))-u(TP(D B))](S-c)ul(TP(D=2)) +[u(TP(D=O))-u(TP(D B))](c+h)u'(TP(D=A)) -u(TP(D=))-u(TP(D=A))] (Ratio) ×[(S-c)d(TP(D=g)-(S-c+π)(TP(D=B)] 3o [u(TP(D=))-u(TP(D=B))] For Ratio>1,u(TP(D=))>u(TP(D=B))>u(TP(D=4))>0 as was explained for(8),under our assumptions,u'()>0,(S-c)>0 and (c+h)>0.Hence the first two expressions of the numerator are positive.The third term is also positive since (TP(D=))<(TP(D=B)).The utility function uis fC) fB) f(C)fB) B 0 (C-B) C)A) fA) l (C-A) B C Fig.2.A concave function fx).Appendix A Lemma 1. Given three scalars C > B > A > 0 and a concave function f(x), f0 (x) > 0, f00(x) < 0, then CA CB < f ðCÞf ðAÞ f ðCÞf ðBÞ . Proof. The claim can be written as f ðCÞf ðBÞ ðCBÞ < f ðCÞf ðAÞ ðCAÞ . As shown in Fig. 2, tanðbÞ ¼ f ðCÞf ðBÞ ðCBÞ and f ðCÞf ðAÞ ðCAÞ ¼ tanða1Þ. The angles a1 = a2 form one side angles between parallel lines. The angle b < a2 since a2 is an external angle to the triangle (f(C),f(B),O). Hence tan(a1) > tan(b) and f ðCÞf ðBÞ ðCBÞ < f ðCÞf ðAÞ ðCAÞ . h Lemma 2. At the feasible range for optimum, the left hand side of Eq. (6) is larger than 1, ðhþSÞðScþpÞ ðpÞðcþhÞ > 1. The claim is that the right hand side of Eq. (6), u½ðScÞQu½ðSAcQÞþhðAQÞ u½ðScÞQu½ðScÞQþpðQBÞ increases monotonically with Q in the range where the left hand side of (6) is larger than 1. Proof. Given that the newsvendor orders Q = Q*, the right hand side ratio of (7) is Ratio ¼ uðTPðDe ¼ Q ÞÞ uðTPðDe ¼ AÞÞ uðTPðDe ¼ Q ÞÞ uðTPðDe ¼ BÞÞ. Taking the derivative with respect to Q to generate the first order condition for optimality oðRatioÞ oQ ¼ ½uðTPðDe ¼ Q ÞÞ uðTPðDe ¼ BÞÞðS cÞu0 ðTPðDe ¼ Q ÞÞ þ½uðTPðDe ¼ Q ÞÞ uðTPðDe ¼ BÞÞðc þ hÞu0 ðTPðDe ¼ AÞÞ ½uðTPðDe ¼ Q ÞÞ uðTPðDe ¼ AÞÞ ½ðS cÞu0 ðTPðDe ¼ Q ÞÞ ðS c þ pÞu0 ðTPðDe ¼ BÞÞ * + ½uðTPðDe ¼ Q ÞÞ uðTPðDe ¼ BÞÞ2 . For Ratio > 1, uðTPðDe ¼ Q ÞÞ > uðTPðDe ¼ BÞÞ > uðTPðDe ¼ AÞÞ > 0 as was explained for (8), under our assumptions, u0 (Æ) > 0, (S c) > 0 and (c + h) > 0. Hence the first two expressions of the numerator are positive. The third term is also positive since u0 ðTPðDe ¼ Q ÞÞ < u0 ðTPðDe ¼ BÞÞ. The utility function u is AB C (C-A) O (C-B) f(C)-f(B) f(C) f(B) f(A) f(C)-f(A) α1 β α2 Fig. 2. A concave function f(x). B. Keren, J.S. Pliskin / European Journal of Operational Research 174 (2006) 1643–1650 1649