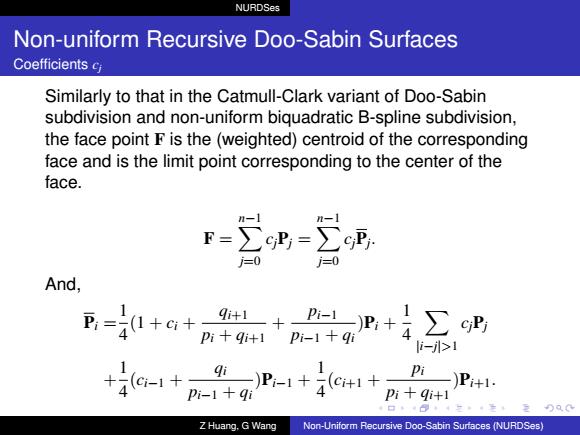
NURDSes Non-uniform Recursive Doo-Sabin Surfaces Coefficients c Similarly to that in the Catmull-Clark variant of Doo-Sabin subdivision and non-uniform biquadratic B-spline subdivision, the face point F is the(weighted)centroid of the corresponding face and is the limit point corresponding to the center of the face. 月一1 F=∑=∑p 0 0 And, R-+a++P+ ∑cP Pi+gi+l Pi-1+gi i->1 1 a(c-1+一)Pi-1+(c+1 pi一)Pi+1 Pi-1+gi pi+gi+1 。生 ZHuang.G Wang Non-Uniform Recursive Doo-Sabin Surtaces (NURDSes) NURDSes Non-uniform Recursive Doo-Sabin Surfaces Coefficients cj Similarly to that in the Catmull-Clark variant of Doo-Sabin subdivision and non-uniform biquadratic B-spline subdivision, the face point F is the (weighted) centroid of the corresponding face and is the limit point corresponding to the center of the face. F = Xn−1 j=0 cjPj = Xn−1 j=0 cjPj . And, Pi = 1 4 (1 + ci + qi+1 pi + qi+1 + pi−1 pi−1 + qi )Pi + 1 4 X |i−j|>1 cjPj + 1 4 (ci−1 + qi pi−1 + qi )Pi−1 + 1 4 (ci+1 + pi pi + qi+1 )Pi+1. Z Huang, G Wang Non-Uniform Recursive Doo-Sabin Surfaces (NURDSes)