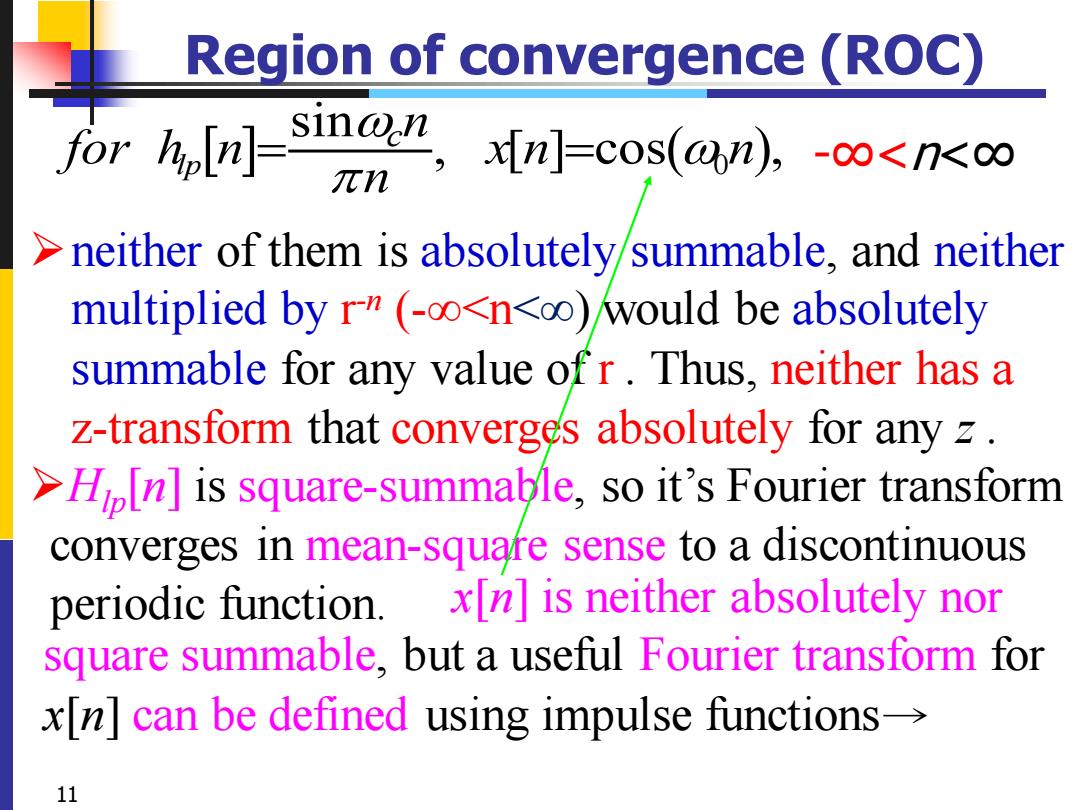
Region of convergence (ROC) drk,[]-i,a小-co@wnho<n∞ >neither of them is absolutely summable,and neither multiplied by r"(-oo<n<oo)would be absolutely summable for any value ofr.Thus,neither has a z-transform that converges absolutely for any z. >HIp[n]is square-summable,so it's Fourier transform converges in mean-square sense to a discontinuous periodic function. x[n]is neither absolutely nor square summable,but a useful Fourier transform for x[n]can be defined using impulse functions-> 1111 Region of convergence (ROC)   ( 0 ) sin , [ ] cos , lp c n for h n x n n n    = = ➢neither of them is absolutely summable, and neither multiplied by r -n (-∞<n<∞) would be absolutely summable for any value of r . Thus, neither has a z-transform that converges absolutely for any z . ➢Hlp[n] is square-summable, so it’s Fourier transform converges in mean-square sense to a discontinuous periodic function. -∞<n<∞ x[n] is neither absolutely nor square summable, but a useful Fourier transform for x[n] can be defined using impulse functions→