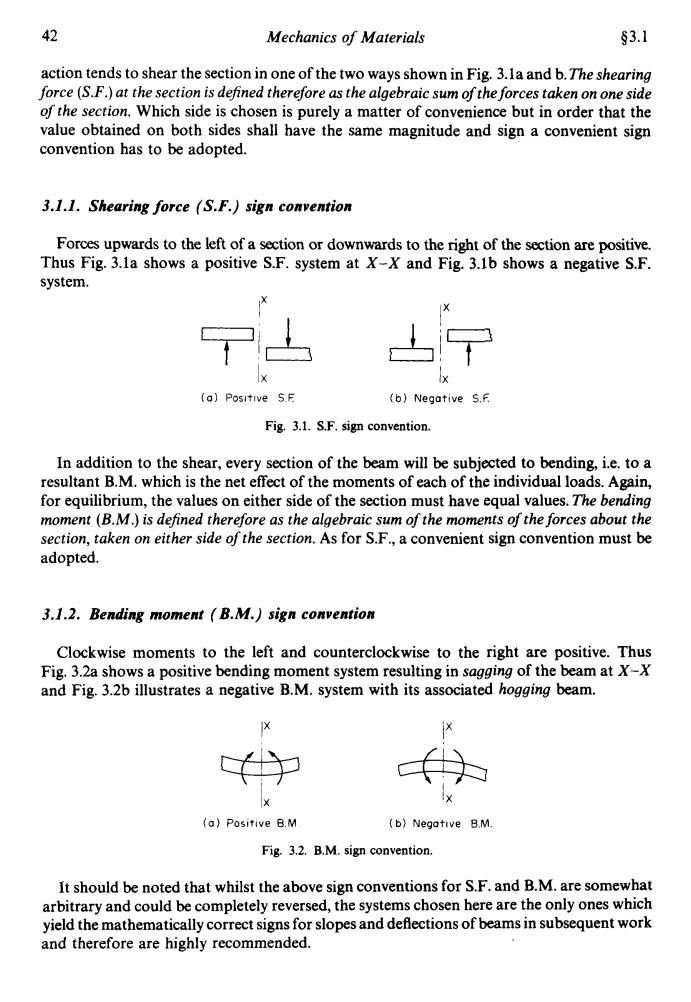
42 Mechanics of Materials §3.1 action tends to shear the section in one of the two ways shown in Fig.3.la and b.The shearing force (S.F.)at the section is defined therefore as the algebraic sum of the forces taken on one side of the section.Which side is chosen is purely a matter of convenience but in order that the value obtained on both sides shall have the same magnitude and sign a convenient sign convention has to be adopted. 3.1.1.Shearing force (S.F.)sign convention Forces upwards to the left of a section or downwards to the right of the section are positive. Thus Fig.3.1a shows a positive S.F.system at X-X and Fig.3.1b shows a negative S.F. system. (o】Positive S.E (b)Negative S.F Fig.3.1.S.F.sign convention. In addition to the shear,every section of the beam will be subjected to bending,i.e.to a resultant B.M.which is the net effect of the moments of each of the individual loads.Again, for equilibrium,the values on either side of the section must have equal values.The bending moment(B.M.)is defined therefore as the algebraic sum of the moments of the forces about the section,taken on either side of the section.As for S.F.,a convenient sign convention must be adopted. 3.1.2.Bending moment B.M.)sign convention Clockwise moments to the left and counterclockwise to the right are positive.Thus Fig.3.2a shows a positive bending moment system resulting in sagging of the beam at X-X and Fig.3.2b illustrates a negative B.M.system with its associated hogging beam. (a)Positive B.M (b)Negative B.M. Fig.3.2.B.M.sign convention. It should be noted that whilst the above sign conventions for S.F.and B.M.are somewhat arbitrary and could be completely reversed,the systems chosen here are the only ones which yield the mathematically correct signs for slopes and deflections of beams in subsequent work and therefore are highly recommended.42 Mechanics of Materials $3.1 action tends to shear the section in one of the two ways shown in Fig. 3.la and b. The shearing force (S.F.) at the section is defined therefore as the algebraic sum of the forces taken on one side of the section. Which side is chosen is purely a matter of convenience but in order that the value obtained on both sides shall have the same magnitude and sign a convenient sign convention has to be adopted. 3.1.1. Shearing force (S.F.) sign convention Forces upwards to the left of a section or downwards to the right of the section are positive. Thus Fig. 3.la shows a positive S.F. system at X-X and Fig. 3.lb shows a negative S.F. system. tX A!'? 723 (b) Negative Ix 5.E IX (a) Positive 5 F: Fig. 3.1. S.F. sign convention. In addition to the shear, every section of the beam will be subjected to bending, i.e. to a resultant B.M. which is the net effect of the moments of each of the individual loads. Again, for equilibrium, the values on either side of the section must have equal values. The bending moment (B.M.) is defined therefore as the algebraic sum of the moments of the forces about the section, taken on either side of the section. As for S.F., a convenient sign convention must be adopted. 3.1.2. Bending moment (B.M.) sign convention Clockwise moments to the left and counterclockwise to the right are positive. Thus Fig. 3.h shows a positive bending moment system resulting in sagging of the beam at X-X and Fig. 3.2b illustrates a negative B.M. system with its associated hogging beam. IX IX Wb IX e IX (a) Positive B M (b) Negative B.M Fig. 3.2. B.M. sign convention. It should be noted that whilst the above sign conventions for S.F. and B.M. are somewhat arbitrary and could be completely reversed, the systems chosen here are the only ones which yield the mathematically correct signs for slopes and deflections of beams in subsequent work and therefore are highly recommended