正在加载图片...
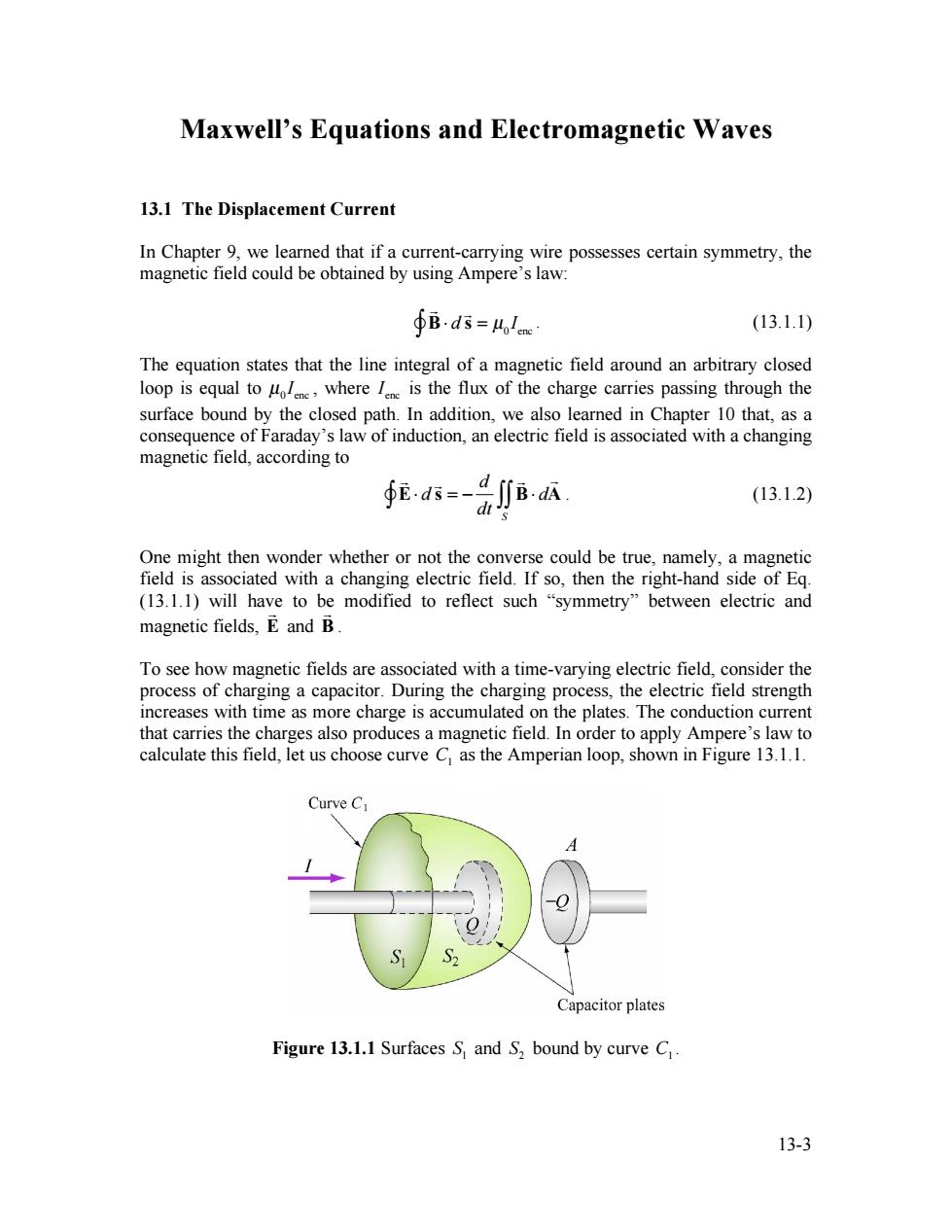
Maxwell's Equations and Electromagnetic Waves 13.1 The Displacement Current In Chapter 9,we learned that if a current-carrying wire possesses certain symmetry,the magnetic field could be obtained by using Ampere's law: Bds=Molcne (13.1.1) The equation states that the line integral of a magnetic field around an arbitrary closed loop is equal to ol where I is the flux of the charge carries passing through the surface bound by the closed path.In addition,we also learned in Chapter 10 that,as a consequence of Faraday's law of induction,an electric field is associated with a changing magnetic field,according to (13.1.2) One might then wonder whether or not the converse could be true,namely,a magnetic field is associated with a changing electric field.If so,then the right-hand side of Eq. (13.1.1)will have to be modified to reflect such "symmetry"between electric and magnetic fields,E and B To see how magnetic fields are associated with a time-varying electric field,consider the process of charging a capacitor.During the charging process,the electric field strength increases with time as more charge is accumulated on the plates.The conduction current that carries the charges also produces a magnetic field.In order to apply Ampere's law to calculate this field,let us choose curve C as the Amperian loop,shown in Figure 13.1.1. Curve C Capacitor plates Figure 13.1.1 Surfaces S and S,bound by curve C 13-313-3 Maxwell’s Equations and Electromagnetic Waves 13.1 The Displacement Current In Chapter 9, we learned that if a current-carrying wire possesses certain symmetry, the magnetic field could be obtained by using Ampere’s law: ! B! d ! s "" = µ0 Ienc . (13.1.1) The equation states that the line integral of a magnetic field around an arbitrary closed loop is equal to 0 enc µ I , where enc I is the flux of the charge carries passing through the surface bound by the closed path. In addition, we also learned in Chapter 10 that, as a consequence of Faraday’s law of induction, an electric field is associated with a changing magnetic field, according to ! E! d ! s "" = # d dt ! B! d ! A S "" . (13.1.2) One might then wonder whether or not the converse could be true, namely, a magnetic field is associated with a changing electric field. If so, then the right-hand side of Eq. (13.1.1) will have to be modified to reflect such “symmetry” between electric and magnetic fields, E ! and B ! . To see how magnetic fields are associated with a time-varying electric field, consider the process of charging a capacitor. During the charging process, the electric field strength increases with time as more charge is accumulated on the plates. The conduction current that carries the charges also produces a magnetic field. In order to apply Ampere’s law to calculate this field, let us choose curve C1 as the Amperian loop, shown in Figure 13.1.1. Figure 13.1.1 Surfaces 1 S and 2 S bound by curve C1