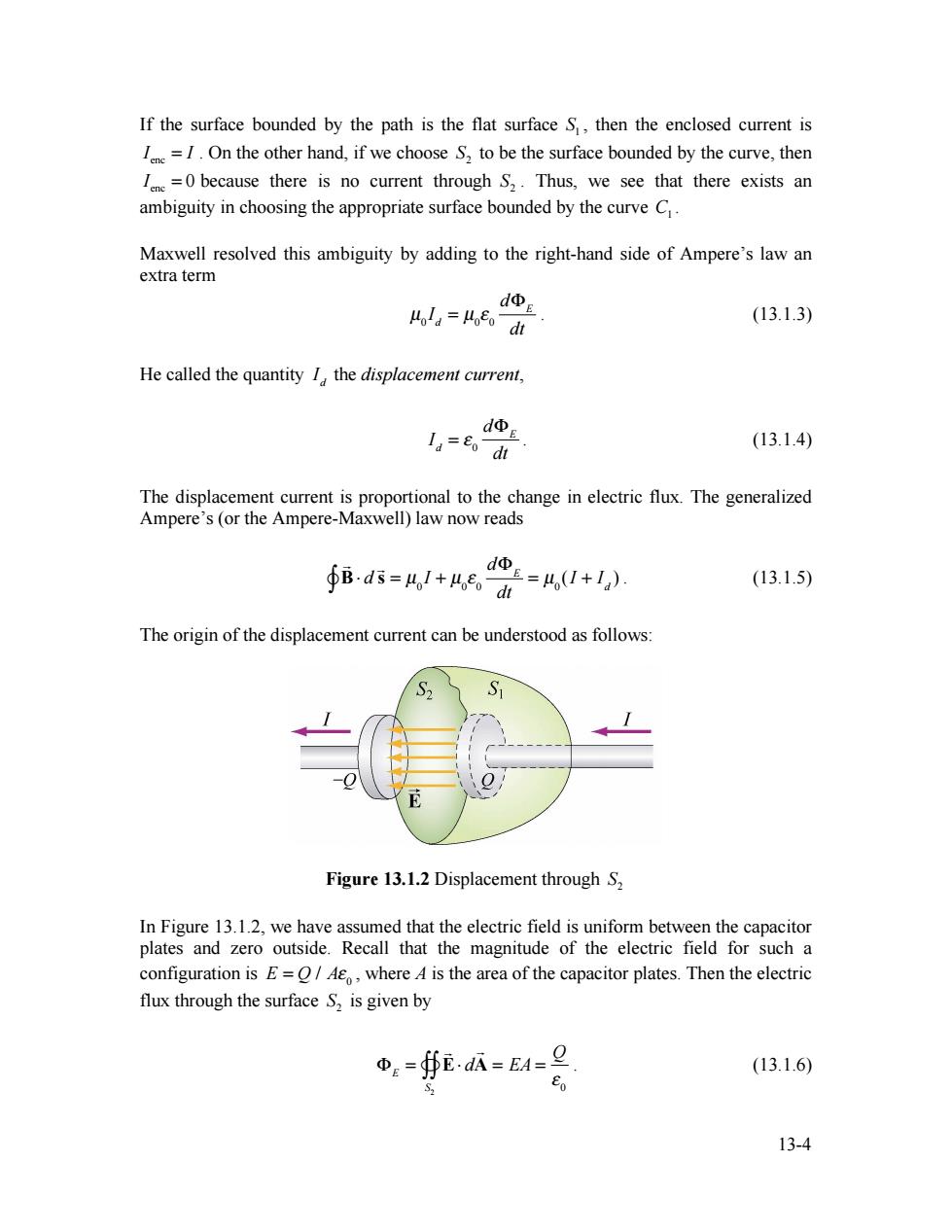
If the surface bounded by the path is the flat surface S,then the enclosed current is I=1.On the other hand,if we choose 2 to be the surface bounded by the curve,then =0 because there is no current through S2.Thus,we see that there exists an ambiguity in choosing the appropriate surface bounded by the curve C. Maxwell resolved this ambiguity by adding to the right-hand side of Ampere's law an extra term dΦE ola=odi (13.1.3) He called the quantity I the displacement current, d Ia=Eo dt (13.1.4) The displacement current is proportional to the change in electric flux.The generalized Ampere's (or the Ampere-Maxwell)law now reads ∮Bd5=/+4,e。 d=4,I+l） (13.1.5) The origin of the displacement current can be understood as follows: S2 Figure 13.1.2 Displacement through S2 In Figure 13.1.2,we have assumed that the electric field is uniform between the capacitor plates and zero outside.Recall that the magnitude of the electric field for such a configuration is E=/A,where A is the area of the capacitor plates.Then the electric flux through the surface S,is given by ΦE=∯EaA=EA=旦 (13.1.6) S2 13-413-4 If the surface bounded by the path is the flat surface 1 S , then the enclosed current is enc I = I . On the other hand, if we choose 2 S to be the surface bounded by the curve, then enc I = 0 because there is no current through 2 S . Thus, we see that there exists an ambiguity in choosing the appropriate surface bounded by the curve C1 . Maxwell resolved this ambiguity by adding to the right-hand side of Ampere’s law an extra term µ0 Id = µ0 ! 0 d"E dt . (13.1.3) He called the quantity Id the displacement current, Id = ! 0 d"E dt . (13.1.4) The displacement current is proportional to the change in electric flux. The generalized Ampere’s (or the Ampere-Maxwell) law now reads ! B! d ! s "" = µ0 I + µ0 # 0 d$E dt = µ0 (I + Id ) . (13.1.5) The origin of the displacement current can be understood as follows: Figure 13.1.2 Displacement through 2 S In Figure 13.1.2, we have assumed that the electric field is uniform between the capacitor plates and zero outside. Recall that the magnitude of the electric field for such a configuration is E = Q / A! 0 , where A is the area of the capacitor plates. Then the electric flux through the surface 2 S is given by !E = ! E" d ! A S2 "## = EA = Q $ 0 . (13.1.6)