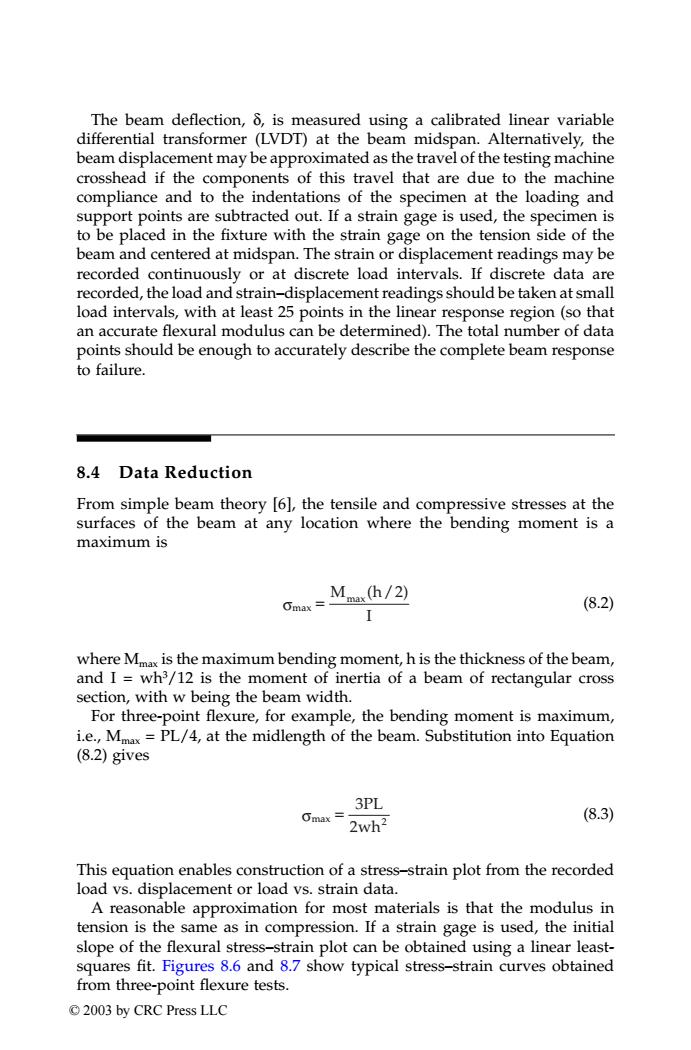
The beam deflection,8,is measured using a calibrated linear variable differential transformer (LVDT)at the beam midspan.Alternatively,the beam displacement may be approximated as the travel of the testing machine crosshead if the components of this travel that are due to the machine compliance and to the indentations of the specimen at the loading and support points are subtracted out.If a strain gage is used,the specimen is to be placed in the fixture with the strain gage on the tension side of the beam and centered at midspan.The strain or displacement readings may be recorded continuously or at discrete load intervals.If discrete data are recorded,the load and strain-displacement readings should be taken at small load intervals,with at least 25 points in the linear response region (so that an accurate flexural modulus can be determined).The total number of data points should be enough to accurately describe the complete beam response to failure. 8.4 Data Reduction From simple beam theory [61,the tensile and compressive stresses at the surfaces of the beam at any location where the bending moment is a maximum is Omax= M(h/2） (8.2) where Mmax is the maximum bending moment,h is the thickness of the beam, and I wh3/12 is the moment of inertia of a beam of rectangular cross section,with w being the beam width. For three-point flexure,for example,the bending moment is maximum, i.e.,Mmax =PL/4,at the midlength of the beam.Substitution into Equation (8.2)gives 3PL Omax= 2wh2 (8.3) This equation enables construction of a stress-strain plot from the recorded load vs.displacement or load vs.strain data. A reasonable approximation for most materials is that the modulus in tension is the same as in compression.If a strain gage is used,the initial slope of the flexural stress-strain plot can be obtained using a linear least- squares fit.Figures 8.6 and 8.7 show typical stress-strain curves obtained from three-point flexure tests. ©2003 by CRC Press LLCThe beam deflection, δ, is measured using a calibrated linear variable differential transformer (LVDT) at the beam midspan. Alternatively, the beam displacement may be approximated as the travel of the testing machine crosshead if the components of this travel that are due to the machine compliance and to the indentations of the specimen at the loading and support points are subtracted out. If a strain gage is used, the specimen is to be placed in the fixture with the strain gage on the tension side of the beam and centered at midspan. The strain or displacement readings may be recorded continuously or at discrete load intervals. If discrete data are recorded, the load and strain–displacement readings should be taken at small load intervals, with at least 25 points in the linear response region (so that an accurate flexural modulus can be determined). The total number of data points should be enough to accurately describe the complete beam response to failure. 8.4 Data Reduction From simple beam theory [6], the tensile and compressive stresses at the surfaces of the beam at any location where the bending moment is a maximum is (8.2) where Mmax is the maximum bending moment, h is the thickness of the beam, and I = wh3/12 is the moment of inertia of a beam of rectangular cross section, with w being the beam width. For three-point flexure, for example, the bending moment is maximum, i.e., Mmax = PL/4, at the midlength of the beam. Substitution into Equation (8.2) gives (8.3) This equation enables construction of a stress–strain plot from the recorded load vs. displacement or load vs. strain data. A reasonable approximation for most materials is that the modulus in tension is the same as in compression. If a strain gage is used, the initial slope of the flexural stress–strain plot can be obtained using a linear least￾squares fit. Figures 8.6 and 8.7 show typical stress–strain curves obtained from three-point flexure tests. max max (/) σ = M h I 2 σmax = 3PL 2wh2 TX001_ch08_Frame Page 127 Saturday, September 21, 2002 4:59 AM © 2003 by CRC Press LLC