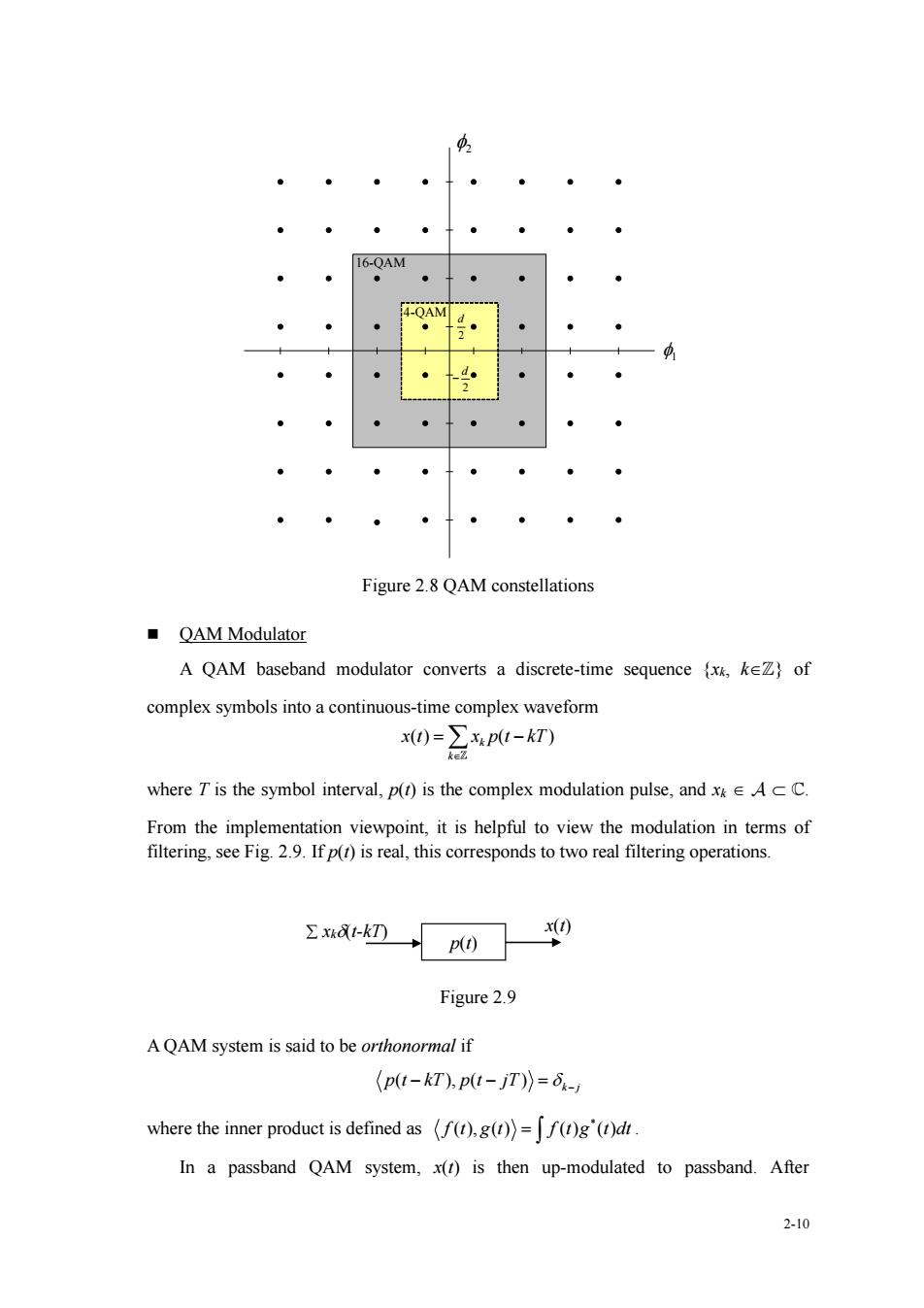
4-QAM Figure 2.8 QAM constellations ■QAM Modulator A QAM baseband modulator converts a discrete-time sequence kZof complex symbols into a continuous-time complex waveform x()=∑P-kT) where T is the symbol interval,p(t)is the complex modulation pulse,and xAcC. From the impementa tion viewpoint,it is helpful to view the modulation in terms of filtering,see Fig.2.9.If p()is real,this corresponds to two real filtering operations. znak009 Figure 2.9 AQAM system is said to be orthonormal if (pu-kT),p1-jT)）=6- where the inner product is defined as (().g()=f()g'(d In a passband QAM system,x(r)is then up-modulated to passband.After 2-10 2-10 1 2 16-QAM 4-QAM 2 d 2 d − Figure 2.8 QAM constellations ◼ QAM Modulator A QAM baseband modulator converts a discrete-time sequence {xk, k} of complex symbols into a continuous-time complex waveform ( ) ( ) k k x t x p t kT  = −  where T is the symbol interval, p(t) is the complex modulation pulse, and xk    . From the implementation viewpoint, it is helpful to view the modulation in terms of filtering, see Fig. 2.9. If p(t) is real, this corresponds to two real filtering operations. Figure 2.9 A QAM system is said to be orthonormal if ( ), ( ) k j p t kT p t jT  − − = − where the inner product is defined as * f t g t f t g t dt ( ), ( ) ( ) ( ) =  . In a passband QAM system, x(t) is then up-modulated to passband. After p(t)  x(t) xk(t-kT)