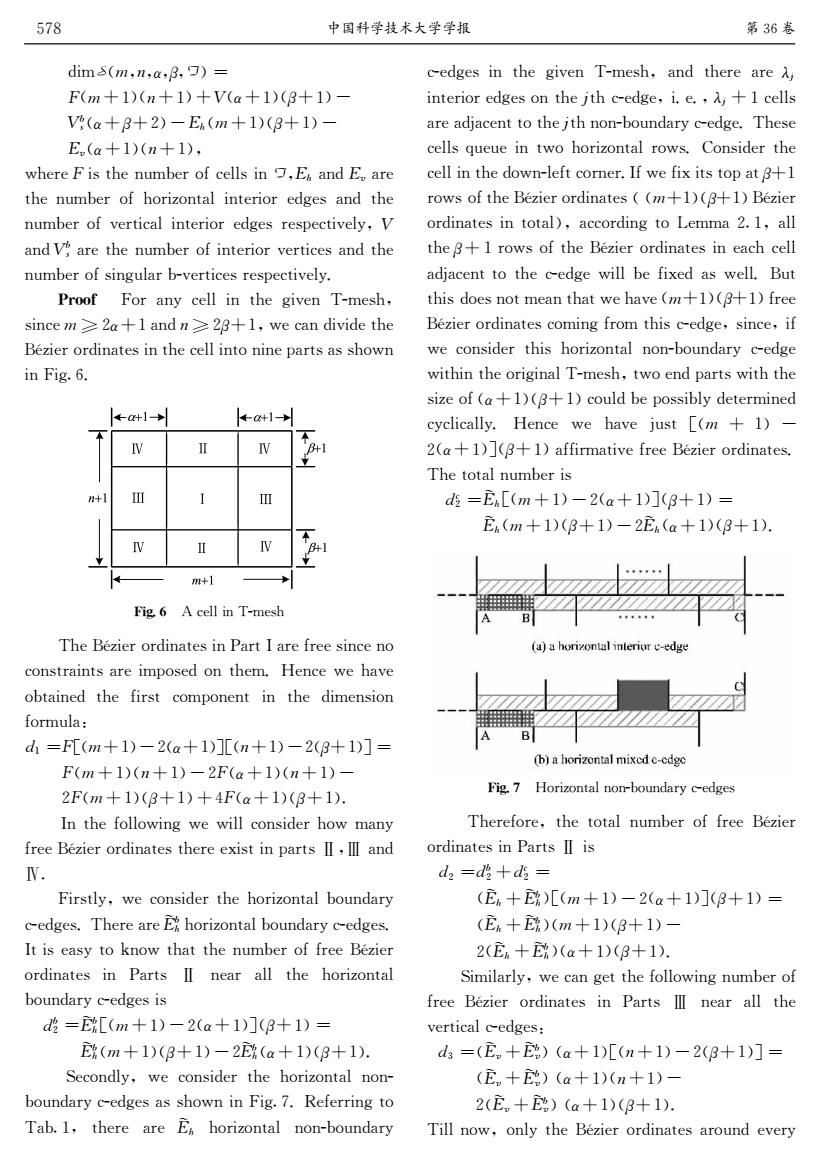
578 中回科学技术大季学摄 第36卷 im5(m,n,a,3,)= c-edges in the given T-mesh.and there are A F(m+1)(n十1)+V(a+1)(3+1)一 interior edges on the jth r-edge,i.e..A+1 rells V(a+B+2)-E(m十1)(3+1)- are adjacent to the jth norrboundary e-edge.These E(a+1)(w+1), cells queue in two horizontal rows.Consider the where F is the numher of cells in Es and E.are cell in the down-left comer.If we fix its top at 8+1 the number of horizontal interior edges and the rows of the Bezier ordinates ((+1)(1)Bezier number of vertical interior edges respectively,V ordinates in total),according to Lemma 2.1.all and V are the number of interior vertices and the the +1 tows of the Bezier ordinates in each cell numher of singular h-vertices respectively. adjacent to the eedge will be fixed as well.But Proof For any cell in the given T-mesh, this does not mean that we have (m+1)(+1)free since m≥2u十1andn≥23+1，we can divide the Bezier ordinates coming from this e-edge.since.if Bezier ordinates in the cell into nine parts as shown we consider this horizontal nomboundary cedge in Fig.6. within the original T-mesh.two end parts with the size of (a+1)(+1)could be possibly determined 女+l+州 +at1→ cyelically,Hence we have just [(m 1) W 2(a+1)(8+1)affirmative free Bezier ordinates. The total number is n+】 1加 d-E[(m+1)-2(m+1)](3+1)= E(m+1)(9+1)-2E.(a+1)(3+1). Ⅱ W 41 优+I Fig 6 A coll in T-mish The Bezier ordinates in Part I are free sinre no la)2 horxumal c-edpe constraints are imposed on them.Hence we have obtained the first component in the dimension formula: d4=(m+1)-2(a+1)][(n+1)-29+1)]= hia3i市mal mxol e-ee F(m+1)(n+1)-2F(a+1)(n+1)- 2F(m+1)(3+1》+4Fa+1)(3+1). Fi 7 Harizontal nan-boumndary cedges In the following we will consider how many Therefore,the total number of free Bezier free Bezier ordinates there exist in parts II,II and ordinates in Parts II is N. d,=十诸= Firstly,we consider the horizontal boundary (E+)[(m+1)-2(a+1)](0+1)= c-edges.There are Et horizontal boundary c-edges. (E+含)(m+1)(3+1) It is easy to know that the number of free Bezier 2(E+)(a+1)(3+1). ordinates in Parts II near all the horizontal Similarly,we can get the following number of houndary c-edges is free Bezier ordinates in Parts near all the d-[(m+1)-2(a+1)](3+1) vertical cedges: (m+1)(3+1)-2(a+1)(9+1). d&=(E.+)(a+1)[(m+1)-2(3+1)]= Secondly.we consider the horizontal nou- (E.+)(a+1)(a+1) houndary c-edges as shown in Fig.7.Referring to 2(E+E)(a+1)(3+1). Tah.1,there are E horizntal non-boundary Till now.only the Bezier ordinates amund every J0I#!’"("""#"!#; G!’DS#!(DS#DF!"DS#!#DS#C F: /!"D#D(#CE,!’DS#!#DS#C E1!"DS#!(DS#" K>2.2G0C/>2,+IM2.$O12%%C0,!"E,?,JE1?.2 />2,+IM2.$O>$.0Q$,/?%0,/2.0$.2J@2C?,J/>2 ,+IM2.$OH2./01?%0,/2.0$.2J@2C.2CG21/0H2%U"F ?,JF: /?.2/>2,+IM2.$O0,/2.0$.H2./012C?,J/>2 ,+IM2.$OC0,@+%?.M6H2./012C.2CG21/0H2%U& L.’’( \$.?,U12%%0,/>2@0H2, F6I2C>" C0,12’ (("DS?,J(((#DS"K21?,J0H0J2/>2 NPQ02.$.J0,?/2C0,/>212%%0,/$,0,2G?./C?CC>$K, 0,\0@Y"& D"/GM -12%%0,F6I2C> F>2NPQ02.$.J0,?/2C0,_?./3?.2O.22C0,12,$ 1$,C/.?0,/C?.20IG$C2J$,/>2I&:2,12K2>?H2 $M/?0,2J/>2O0.C/1$IG$,2,/0, />2 J0I2,C0$, O$.I+%?$ 4S ;G%!’DS#C(!"DS#&%!(DS#C(!#DS#&; G!’DS#!(DS#C(G!"DS#!(DS#C (G!’DS#!#DS#DTG!"DS#!#DS#< 3,/>2O$%%$K0,@K2K0%%1$,C0J2.>$K I?,U O.22NPQ02.$.J0,?/2C/>2.22Z0C/0,G?./C#"$?,J %& \0.C/%U"K21$,C0J2./>2>$.0Q$,/?%M$+,J?.U 162J@2C&F>2.2?.2E): ,>$.0Q$,/?%M$+,J?.U162J@2C& 3/0C2?CU/$[,$K/>?//>2,+IM2.$OO.22NPQ02. $.J0,?/2C0, _?./C # ,2?. ?%%/>2 >$.0Q$,/?% M$+,J?.U162J@2C0C 4: ( ;E): ,%!’DS#C(!"DS#&!#DS#; E): ,!’DS#!#DS#C(E): ,!"DS#!#DS#< W21$,J%U"K21$,C0J2./>2>$.0Q$,/?%,$,6 M$+,J?.U162J@2C?CC>$K,0,\0@Y7&X2O2..0,@/$ F?MYS"/>2.2 ?.2 E), >$.0Q$,/?% ,$,6M$+,J?.U 162J@2C0,/>2@0H2, F6I2C>"?,J/>2.2?.2’@ 0,/2.0$.2J@2C$,/>2@/>162J@2"0&2&"’@ DS12%%C ?.2?JA?12,//$/>2@/>,$,6M$+,J?.U162J@2&F>2C2 12%%Cb+2+20,/K$>$.0Q$,/?%.$KC&V$,C0J2./>2 12%%0,/>2J$K,6%2O/1$.,2.&3OK2O0Z0/C/$G?/#DS .$KC$O/>2NPQ02.$.J0,?/2C!!’DS#!#DS#NPQ02. $.J0,?/2C0,/$/?%#"?11$.J0,@/$D2II?(YS"?%% />2#DS.$KC$O/>2NPQ02.$.J0,?/2C0,2?1>12%% ?JA?12,//$/>2162J@2K0%%M2O0Z2J?CK2%%&N+/ />0CJ$2C,$/I2?,/>?/K2>?H2!’DS#!#DS#O.22 NPQ02.$.J0,?/2C1$I0,@O.$I/>0C162J@2"C0,12"0O K21$,C0J2./>0C>$.0Q$,/?%,$,6M$+,J?.U162J@2 K0/>0,/>2$.0@0,?%F6I2C>"/K$2,JG?./CK0/>/>2 C0Q2$O!"DS#!#DS#1$+%JM2G$CC0M%UJ2/2.I0,2J 1U1%01?%%U& :2,12 K2 >?H2 A+C/ %!’ D S#C (!"DS#&!#DS#?OO0.I?/0H2O.22NPQ02.$.J0,?/2C& F>2/$/?%,+IM2.0C 4. ( ;E),%!’DS#C(!"DS#&!#DS#; E),!’DS#!#DS#C(E),!"DS#!#DS#< D"/GN :$.0Q$,/?%,$,6M$+,J?.U162J@2C F>2.2O$.2"/>2/$/?%,+IM2.$OO.22NPQ02. $.J0,?/2C0,_?./C#0C 4( ;4: ( D4. ( ; !E), DE): ,#%!’DS#C(!"DS#&!#DS#; !E), DE): ,#!’DS#!#DS#C (!E), DE): ,#!"DS#!#DS#< W0I0%?.%U"K21?,@2//>2O$%%$K0,@,+IM2.$O O.22 NPQ02. $.J0,?/2C0, _?./C $ ,2?.?%%/>2 H2./01?%162J@2C$ 4! ;!E)1 DE): 1#!"DS#%!(DS#C(!#DS#&; !E)1 DE): 1#!"DS#!(DS#C (!E)1 DE): 1#!"DS#!#DS#< F0%%,$K"$,%U/>2NPQ02.$.J0,?/2C?.$+,J2H2.U 578 CDEFGHIFFJ K!"L