中国科学技术大学：JOURNAL OF UNIVERSITY OF SCIENCE AND TECHNOLOGY OF CHINA.pdf_大学文库

第36卷第6期中国神学技术大学学报 Vol.36,No.6 2006年6月 JOURNAL OF UNIVERSITY OF SCIENCE AND TECHNOLOGY OF CHINA Jun.2006 Article ID:0253-2778(2006)06-0573-09 Dimensions of spline spaces over general T-meshes HUANG Zhang-jin, DENG Jian-song, LI Xin (De partment of Matheratics,Umiversity of Science and Technology of China.Hefei 230026,China) Abstract: The spline spaces over general T-meshes and the periodic spline spaces over regular T- meshes were studied. With the method based on B-nets the dimension formulae were proposed as well. Key words: T-mesh; spline; Bezier ordinate; dimension CLC number: O174. 4; O241. 5 Document code: A AMS Subject Classification(2000): 65D07 一般T网格上样条空间的维数黄章进，邓建松，李新 (中国科学技术大学数学系，安徽合肥230026) 摘要：研究了一般T网格上的样条空间和规则T网格上的周期样条空间，并用基于B网的方法给出了它们的维数公式. 关键词：T网格；样条；Bézier纵标；维数 spline over T-meshes, i. e., for every vertex, a 0 Introduction blending function is defined. Each of the blending T-meshes are formed by a set of horizontal functions comes from some tensor-product spline line segments and a set of vertical line segments, space. Though this type of spline supports many where T-junctions are allowed. It could be thought valuable operations within a consistent framework, of as a transition between the simple tensor- some of them, such as, evaluation and local product meshes and the complicated triangular refinement, are not simple. In the T-spline meshes. Over the T-meshes, we can define many theory, the basis functions are rational which lead types of spline spaces. Currently, there are basically two types of spline spaces defined over T- to evaluations and are not simple; on the other meshes: one is from Sederberg et al, and the hand, the local refinement is dependent on the other is from Deng et al2]. structure of the mesh, and its complexity is Ref. [1] invented T-spline. It is a point-based uncertain. Furthermore, it is an ongoing problem Received: 2005-11-10; Revised: 2006-05-17 Foundation item: Supported by the Outstanding Youth Grant of NSF of China (60225002), National Key Basic Research Project of China (2004CB318000), NSF of China (60473132), the TRAPOYT in Higher Education Institute of MOE of China, and SRF for ROCS, SEM. Biography: HUANG Zhang-jin, male, born in 1980, PhD. Research field:CAGD and CG. E-mail: zjh@mail. ustc. edu. cn Corresponding author: DENG Jian-song, Prof. E-mail: dengjs@ustc. edu. cn

574 中四种学被术大李学极第36卷 whether T-spline blending functions are always over T-meshes. linearly independentta.The reason for these 1.I T-meshes problems is mainly that the spline over every cell of T-meshes are formed by a set of horicontal the mesh is not a polynomial.hut a piecewise line segments and a set of vertical line segments. polynomial. where T-junctions are allowed.We can also think Forcing functions to be a tensor-proxduxct that the T-mesh is a simply connected domain polynomial on every cell and to satisfy the specified formed by the union of a set of rectangles whose smoothness across common edges,Deng er al sides are horizontal or vertical.Fig.I illustrates introduced the spline spaces over T-meshes4. two examples of T-meshes.while in Fig.2 two They derived the dimension formulae for the spline examples of non-T-meshes are shown. spaces over regular T-meshes via a method based on B-nets.Here "regular"means that the whole domains are rectangles without holes inside. Comparing with Sederberg's T-spline,the surface moxdeling based on the spline spaces over T-meshes allows local refinement to he really local.And the evaluation is quick sinee this type of spline is Fig.1 Two examples of T-meshes polynomial,nat rational. However,all these works about spline spaces over T-meshes only take into account the regular T-meshes,and the spline surfaces defined over such T-meshes will have topological equivalent to the disks.In the practice of surface modeling.the b boundary of the domain of a complex surfare may Fig.2 Two examples of ron-T-meshes be an arbitrary polygon.and the domain may also Fig.3 gives a more complicated T-mesh, have one ar several holes.For example,if we fit a where three parts with gray fill-in are excluded spline surface over T-meshes according to the from the T-mesh.Hence there are three holes in range data of some mask,then we need the domain the T-mesh.Here each small rectangle in the T- T-mesh to have at least three holes inside,one for mesh is called a cell ar a facet.The corners of the mouth,and the other two for two eyes.On the these rectangles are called vertices of the T-mesh. other hand,if we want to model a simple close If a vertex is on the boundary,then it is called a surface or a cylindrical surface with a close section. boundary vertex.Otherwise,it is called an interior then we need periodic splines.Hence in this paper, we consider the spline function spares over general T-meshes.For the regular T-meshes.periodic splines are discussed as well.With the method hs 6 based on B-nets proposed by Deng er al.we ，4 derive the dimension formulae of these spline spaces. Spline spaces over T-meshes In this section,we first review some concepts related to T-meshes and the spline function spaces Fig.3 A T-mesh with notatians

K>2/>2. F6CG%0,2M%2,J0,@O+,1/0$,C?.2?%K?UC %0,2?.%U 0,J2G2,J2,/!!" & F>2 .2?C$, O$. />2C2 G.$M%2IC0CI?0,%U/>?//>2CG%0,2$H2.2H2.U12%%$O />2 I2C>0C,$/?G$%U,$I0?%#M+/?G0212K0C2 G$%U,$I0?%& \$.10,@ O+,1/0$,C /$ M2 ? /2,C$.6G.$J+1/ G$%U,$I0?%$,2H2.U12%%?,J/$C?/0COU/>2CG210O02J CI$$/>,2CC?1.$CC1$II$, 2J@2C#42,@"&$6 0,/.$J+12J/>2CG%0,2CG?12C $H2. F6I2C>2C!(" & F>2UJ2.0H2J/>2J0I2,C0$,O$.I+%?2O$./>2CG%0,2 CG?12C$H2..2@+%?.F6I2C>2CH0??I2/>$JM?C2J $,N6,2/C&:2.2$.2@+%?.%I2?,C/>?//>2K>$%2 J$I?0,C ?.2 .21/?,@%2C K0/>$+/ >$%2C 0,C0J2& V$IG?.0,@K0/>W2J2.M2.@aCF6CG%0,2#/>2C+.O?12 I$J2%0,@M?C2J$,/>2CG%0,2CG?12C$H2.F6I2C>2C ?%%$KC%$1?%.2O0,2I2,//$M2.2?%%U%$1?%&-,J/>2 2H?%+?/0$,0Cb+01[C0,12/>0C/UG2$OCG%0,20C G$%U,$I0?%#,$/.?/0$,?%& :$K2H2.#?%%/>2C2K$.[C?M$+/CG%0,2CG?12C $H2.F6I2C>2C$,%U/?[20,/$?11$+,//>2.2@+%?. F6I2C>2C#?,J/>2CG%0,2C+.O?12CJ2O0,2J$H2. C+1>F6I2C>2CK0%%>?H2/$G$%$@01?%2b+0H?%2,//$ />2J0C[C&3,/>2G.?1/012$OC+.O?12I$J2%0,@#/>2 M$+,J?.U$O/>2J$I?0,$O?1$IG%2ZC+.O?12I?U M2?,?.M0/.?.UG$%U@$,#?,J/>2J$I?0,I?U?%C$ >?H2$,2$.C2H2.?%>$%2C&\$.2Z?IG%2#0OK2O0/? CG%0,2C+.O?12 $H2. F6I2C>2C ?11$.J0,@ /$ />2 .?,@2J?/?$OC$I2I?C[#/>2,K2,22J/>2J$I?0, F6I2C>/$>?H2?/%2?C//>.22>$%2C0,C0J2#$,2O$. />2I$+/>#?,J/>2$/>2./K$O$./K$2U2C&R,/>2 $/>2.>?,J#0OK2K?,//$ I$J2%?C0IG%21%$C2 C+.O?12$.?1U%0,J.01?%C+.O?12K0/>?1%$C2C21/0$,# />2,K2,22JG2.0$J01CG%0,2C&:2,120,/>0CG?G2.# K21$,C0J2./>2CG%0,2O+,1/0$,CG?12C$H2.@2,2.?% F6I2C>2C&\$./>2.2@+%?. F6I2C>2C#G2.0$J01 CG%0,2C?.2J0C1+CC2J?C K2%%&L0/>/>2 I2/>$J M?C2J$,N6,2/CG.$G$C2JMU 42,@"&$6!("#K2 J2.0H2/>2 J0I2,C0$, O$.I+%?2 $O/>2C2 CG%0,2 CG?12C& F >)*"%$&)+,$&’-$.01#$&2$& 3,/>0CC21/0$,#K2O0.C/.2H02KC$I21$,12G/C .2%?/2J/$F6I2C>2C?,J/>2CG%0,2O+,1/0$,CG?12C $H2.F6I2C>2C& FGF 01#$&2$& F6I2C>2C?.2O$.I2JMU?C2/$O>$.0Q$,/?% %0,2C2@I2,/C?,J?C2/$OH2./01?%%0,2C2@I2,/C# K>2.2F6A+,1/0$,C?.2?%%$K2J&L21?,?%C$/>0,[ />?//>2 F6I2C>0C?C0IG%U1$,,21/2J J$I?0, O$.I2JMU/>2+,0$,$O?C2/$O.21/?,@%2CK>$C2 C0J2C?.2>$.0Q$,/?%$.H2./01?%&\0@YS0%%+C/.?/2C /K$2Z?IG%2C$OF6I2C>2C#K>0%20,\0@Y(/K$ 2Z?IG%2C$O,$,6F6I2C>2C?.2C>$K,& D"/GF FK$2Z?IG%2C$OF6I2C>2C D"/G@ FK$2Z?IG%2C$O,$,6F6I2C>2C D"/GH -F6I2C>K0/>,$/?/0$,C \0@Y! @0H2C ? I$.2 1$IG%01?/2J F6I2C># K>2.2/>.22G?./CK0/>@.?UO0%%60,?.22Z1%+J2J O.$I/>2F6I2C>&:2,12/>2.2?.2/>.22>$%2C0, />2F6I2C>&:2.22?1>CI?%%.21/?,@%20,/>2F6 I2C>0C1?%%2J?12%%$.?O?12/&F>21$.,2.C$O />2C2.21/?,@%2C?.21?%%2JH2./012C$O/>2F6I2C>& 3O?H2./2Z0C$,/>2M$+,J?.U#/>2,0/0C1?%%2J? M$+,J?.UH2./2Z&R/>2.K0C2#0/0C1?%%2J?,0,/2.0$. 57T CDEFGHIFFJ K!"L

第B潮 Dimensions of spline spaces over general T-meshes 575 vertex.For example,6,(i 1....32)in Fig.3 is called a houndary c-edge.for example,b in are boundary vertices,and all the other vertices Fig,3.An interior cedge is the eedge which (i =1...8)are interior vertices. consists of only interior edges,for example,vv Interior vertices have two types.One is and nshs in Fig.3.A mixed c-edge is the c-edge crossing.for example,v:in Fig.3:and the other is which consists of both interior cdges and boundary T-junctional,for example,i in Fig.3.We call edges.for example,vzbic and bs in Fig.3.Mixed them crossing vertices and T-vertices respectively. c-edges and interior cedges are also called non- Boundary vertices also have two types.If a boundary eedges. boundary vertex is on the boundary of some cell. The regular T-mesh considered by Deng et but not any corner of the cell,then it is called an alf)is such a T-mesh that the union of its cells inner b-vertex:otherwise,it is called a free b- forms a reetangle,see Fig.1(b).In a regular T- vertex.For example,by in Fig.3 is an inner b mesh,a cedge is either a boundary cedge or an vertex.and bie a free b-vertex.Especially.if a free interior c-edge. vertex is the common corner and the only 1.2 The spline space interxection point of two cells.then it is ralled a Given a T-mesh 7.we use 9 to dennte all the singular h-vertex,such as b and hu in Fig.3. cells in andn to denote the region occupied by all The line segment connecting two adjacent the cells in The spline space over the given T. vertices is called an edge of the T-mesh.If an edge mesh is defined as is on the boundary of the T-mesh.then it is called s(m,a,3,了)= a boundary edge;otherwise it is called an interior {sx,y)∈C)ls(ry)ls∈Pm,V∈9} edge.For example,in Fig.3.and m are where is the space of all polynomials with bi interior edges whileb and babe are boundary degree (m.n).and C(n)is the space consisting edges. of all bivariate functions which are continuous in f Two cells are called adjacent if they share a with order a along the r direction and with order common cdge as part of their boundaries.If one along the y direetion. cell is above or below the other,then they are It is obvious that the spline spaces are lincar called adjacent vertically.If one cell is on the left spaces and the study as to its dimensions property or right of the other,then they are called adjacent is an interesting problem.The results are useful for horizontally.A cell is called adjacent to a grid line surface modeling with the spline spaces over T-meshes (an edge ar composition of several edges)if some 2 boundary line of the cell is part of the grid line.For Review of B-net method example,in Fig.3.vvbv and ibububis are B-net method is used in the proof of the adjacent vertically.and vh6 are dimension formulae proposed by Deng era.We adjacent horizontally,ande is adjacent to will also use B-net method to discuss the 劝. dimensions of the spline spaces over general T. A romposite edge (shortly,c-edge)is the meshes. longest possible line segment in the T-mesh.The Let (c.y)and (r,y)be two polynomials inner vertices (vertices except the end points of the with bi-degree (m,),defined over two adjacent line scgment)are all cither interior T-vertices or domains inner bvertices.For example,in Fig.3,tbie, [西]×[b] hbz,bs,nnd:sare-rges,while的， and and hby are not.C-edges have three [m,]×[h], types.If a e-edge is just a boundary edge,then it respectively.They can be expressed in Bernsteir

H2./2Z&\$.2Z?IG%2!:-"-;S!#!!($0,\0@Y! ?.2M$+,J?.UH2./012C!?,J?%%/>2$/>2.H2./012C1- "-;S!#!8$?.20,/2.0$.H2./012C& 3,/2.0$. H2./012C >?H2 /K$ /UG2C& R,20C 1.$CC0,@!O$.2Z?IG%2!1(0,\0@Y!%?,J/>2$/>2.0C F6A+,1/0$,?%!O$.2Z?IG%2!1S0,\0@Y!&L21?%% />2I1.$CC0,@H2./012C?,JF6H2./012C.2CG21/0H2%U& N$+,J?.U H2./012C ?%C$ >?H2 /K$ /UG2C&3O ? M$+,J?.UH2./2Z0C$,/>2M$+,J?.U$OC$I212%%! M+/,$/?,U1$.,2.$O/>212%%!/>2,0/0C1?%%2J?, 0,,2.M6H2./2Z%$/>2.K0C2!0/0C1?%%2J?O.22M6 H2./2Z&\$.2Z?IG%2!:S70,\0@Y!0C?,0,,2.M6 H2./2Z!?,J:S"?O.22M6H2./2Z&BCG210?%%U!0O?O.22 M6H2./2Z0C />2 1$II$, 1$.,2. ?,J />2 $,%U 0,/2.C21/0$,G$0,/$O/K$12%%C!/>2,0/0C1?%%2J? C0,@+%?.M6H2./2Z!C+1>?C:9?,J:(90,\0@Y!& F>2%0,2 C2@I2,/1$,,21/0,@ /K$ ?JA?12,/ H2./012C0C1?%%2J?,2J@2$O/>2F6I2C>&3O?,2J@2 0C$,/>2M$+,J?.U$O/>2F6I2C>!/>2,0/0C1?%%2J ?M$+,J?.U2J@2%$/>2.K0C20/0C1?%%2J?,0,/2.0$. 2J@2&\$.2Z?IG%2!0,\0@Y!!1S:() ?,J1S1( ?.2 0,/2.0$.2J@2CK>0%2:(:! ?,J:(S:(( ?.2M$+,J?.U 2J@2C& FK$12%%C?.21?%%2J?JA?12,/0O/>2UC>?.2? 1$II$,2J@2?CG?./$O/>20.M$+,J?.02C&3O$,2 12%%0C?M$H2$.M2%$K/>2$/>2.!/>2,/>2U?.2 1?%%2J?JA?12,/H2./01?%%U&3O$,212%%0C$,/>2%2O/ $..0@>/$O/>2$/>2.!/>2,/>2U?.21?%%2J?JA?12,/ >$.0Q$,/?%%U&-12%%0C1?%%2J?JA?12,//$?@.0J%0,2 "?,2J@2$.1$IG$C0/0$,$OC2H2.?%2J@2C$0OC$I2 M$+,J?.U%0,2$O/>212%%0CG?./$O/>2@.0J%0,2&\$. 2Z?IG%2!0, \0@Y!!1!1T15:S" ?,J1":ST:S5:S" ?.2 ?JA?12,/H2./01?%%U!1!1T15:S" ?,J1(:S7:S8:S9 ?.2 ?JA?12,/>$.0Q$,/?%%U!?,J1!1T15:S"0C?JA?12,//$ 1S:S5$./%U!162J@2$0C/>2 %$,@2C/G$CC0M%2%0,2C2@I2,/0,/>2F6I2C>&F>2 0,,2.H2./012C"H2./012C2Z12G//>22,JG$0,/C$O/>2 %0,2C2@I2,/$?.2?%%20/>2.0,/2.0$.F6H2./012C$. 0,,2.M6H2./012C&\$.2Z?IG%2!0, \0@Y!!1":S"! 15:(5!1(:S"!?,J:S5:S" ?.2162J@2C!K>0%21S1!! 1(:S7!1(:S5 ?,J:S":S7 ?.2,$/&V62J@2C>?H2/>.22 /UG2C&3O?162J@20CA+C/?M$+,J?.U2J@2!/>2,0/ 0C1?%%2J?M$+,J?.U162J@2!O$.2Z?IG%2!:S:(0, \0@Y!&-,0,/2.0$.162J@20C/>2162J@2 K>01> 1$,C0C/C$O$,%U0,/2.0$.2J@2C!O$.2Z?IG%2!1S1( ?,J1":S"0,\0@Y!&- I0Z2J162J@20C/>2162J@2 K>01>1$,C0C/C$OM$/>0,/2.0$.2J@2C?,JM$+,J?.U 2J@2C!O$.2Z?IG%2!1(:S"?,J15:(50,\0@Y!&‘0Z2J 162J@2C?,J0,/2.0$.162J@2C?.2?%C$1?%%2J,$,6 M$+,J?.U162J@2C& F>2.2@+%?. F6I2C>1$,C0J2.2JMU 42,@"& $6&(’ 0CC+1>?F6I2C>/>?//>2+,0$,$O0/C12%%C O$.IC?.21/?,@%2!C22\0@YS"M$&3,?.2@+%?.F6 I2C>!?162J@20C20/>2.?M$+,J?.U162J@2$.?, 0,/2.0$.162J@2& FG@ 02$&)*"%$&)+,$ !!K2+C2"/$J2,$/2?%%/>2 12%%C0,!?,J!/$J2,$/2/>2.2@0$,$11+G02JMU?%% />212%%C0,!2CG%0,2CG?12$H2./>2@0H2,F6 I2C>!0CJ2O0,2J?C #"’!(!"!#!!$=; (/">!2$#8"!#"!$/">!2$$ # $’(!$$# ")! K>2.2$’(0C/>2CG?12$O?%%G$%U,$I0?%CK0/>M06 J2@.22"’!($!?,J8"!#"!$0C/>2CG?121$,C0C/0,@ $O?%%M0H?.0?/2O+,1/0$,CK>01>?.21$,/0,+$+C0,! K0/>$.J2."?%$,@/>2>J0.21/0$,?,JK0/>$.J2.# ?%$,@/>22J0.21/0$,& 3/0C$MH0$+C/>?//>2CG%0,2CG?12C?.2%0,2?. CG?12C?,J/>2C/+JU?C/$0/CJ0I2,C0$,CG.$G2./U 0C?,0,/2.2C/0,@G.$M%2I&F>2.2C+%/C?.2+C2O+%O$. C+.O?12I$J2%0,@K0/>/>2CG%0,2CG?12C$H2.F6I2C>2C& @ C$-"$8’(E1%$5#$52’9 N6,2/ I2/>$J0C +C2J0,/>2 G.$$O$O/>2 J0I2,C0$,O$.I+%?2G.$G$C2JMU42,@"&$6&(’ &L2 K0%% ?%C$ +C2 N6,2/ I2/>$J /$ J0C1+CC />2 J0I2,C0$,C$O/>2CG%0,2CG?12C$H2.@2,2.?%F6 I2C>2C& D2/%S">!2$?,J%(">!2$M2/K$G$%U,$I0?%C K0/>M06J2@.22"’!($!J2O0,2J$H2./K$?JA?12,/ J$I?0,C &>)!>S’?&2)!2S’ ?,J &>S!>(’?&2)!2S’! .2CG21/0H2%U&F>2U1?,M22ZG.2CC2J0,N2.,C/20,6 K"2 40I2,C0$,C$OCG%0,2CG?12C$H2.@2,2.?%F6I2C>2C 575

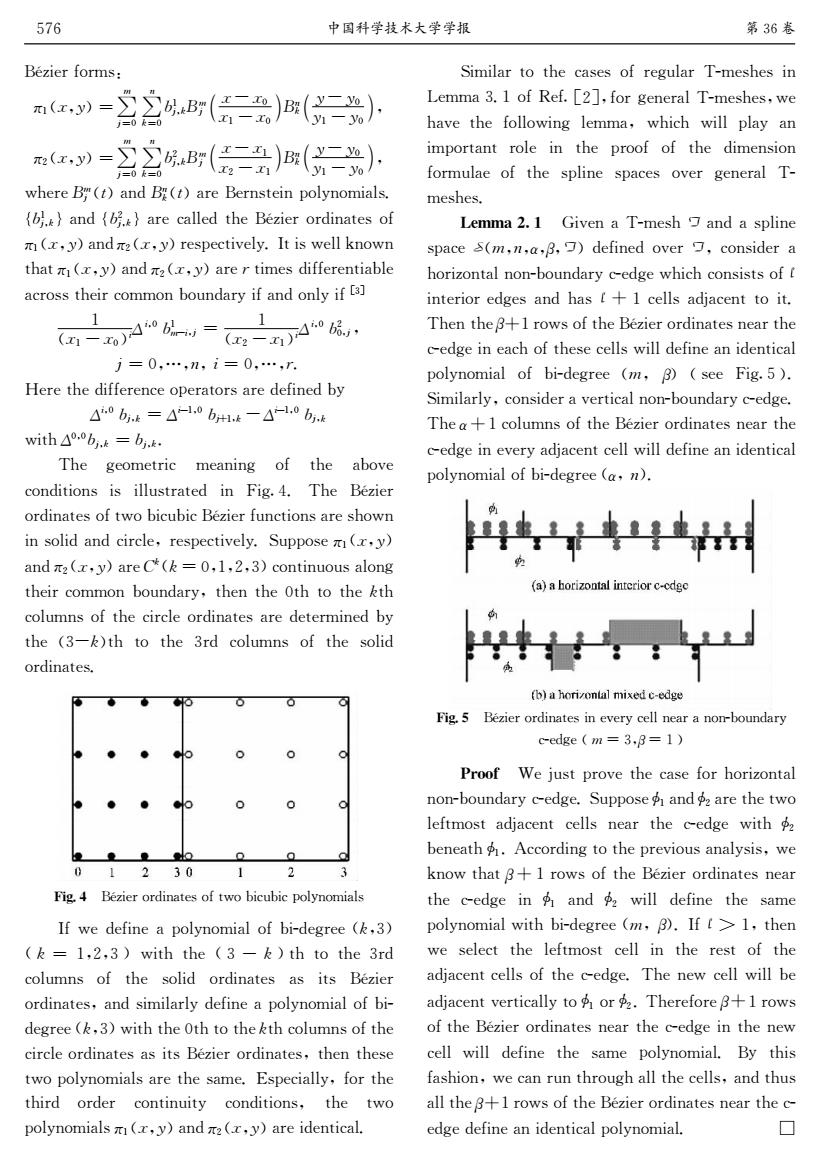
576 中回科学徒术大零学摄第36表 Bezier forms: Similar to the caxes of regular T-meshes in Lemma 3.1 of Ref.[2],for general T-meshes,we have the following lemma.which will play an -含2r信（货 important role in the proof of the dimension formulae of the spline spaces over general T- where B(t)and B()are Bernstein polynomials. meshes. {以，.】and{.}are called the Bezier ordinates of Lemma 2.I Given a T-mesh and a spline m(r.y)and(r.y)respertively.It is well known space s(m,i,a,3,)defined over了，consider a that(.c,y)and (c,y)are r times differentiable horizontal non-boundary cedge which consists of I across their commo boundary if and ony if interior edges and has !+I cells adjacent to it. -n20n4成· Then the +1 rows of the Bezier ordinates near the edge in each of these cells will define an identical j=0,…,,了=0，…， polynomial of bi-degree (m.see Fig.5). Here the difference operators are defined by Similarly,consider a vertical notrboundary cedge. 4b4=△-10644-△-10bc The a+1 columns of the Bezier ordinates near the with△0.h.t=hk cedge in every adjacent cell will define an identical The geometric meaning of the above polynomial of bi-degree (a,n). conditions is illustrated in Fig.4.The Bezier ordinates of two birubic Bezier functions are shown in solid and circle,respectively.Suppose m.y) and (c.y)are C(-0.1.2,3)continuous along 件m their common boundary,then the Oth to the kth columns of the circle ordinates are determined by the (3-)th to the 3rd columns of the solid ordinates. b)a hrmyuntal mived c-edpe Fig.5 Bezier ordntes in every cvll meur s nourboundary c-edge (-3.8-1) Proof We just prove the case for horizontal non-boundary c-edge.Suppose$andf:are the two leftmost adjacent cells near the eedge with beneath According to the previous analysis,we 01 1301 know that a+1 rows of the Bezier ordinates near Fig 4 Birier ordirates of two bicuhic polynommials the c-edge in and will define the same If we define a polynomial of hi-degree (,3) polynomial with bi-degree (m.B).If 1,then (1,2.3 with the (3-)th to the 3rd we select the leftmost cell in the rest of the columns of the solid ordinates as its Bezier adjacent cells of the cedge.The new cell will be ordinates,and similarly define a polynomial of bi- adjacent vertically to or.Therefore 8+1 rows degree (.3)with the Oth to the tth columns of the of the Bezier ordinates near the cedge in the new circle ordinates as its Bezier ordinates,then these cell will define the same polynomial.By this two polynomials are the same.Especially.for the fashion,we can nm through all the cells,and thus third order continuity conditions,the two all the +1 rows of the Bezier ordinates near the e polynomials(r.y)and ga(r,y)are identical edge define an identical polypomial. ▣

NPQ02.O$.IC! %S">#2$;% ’ @;)% ( A;) :S @#AB’ @ >C>) ">S C>)$B( A 2C2) "2S C2)$# %(">#2$;% ’ @;)% ( A;) :( @#AB’ @ >C>S ">( C>S$B( A 2C2) "2S C2)$# K>2.2B’ @ "&$?,JB( A"&$?.2N2.,C/20,G$%U,$I0?%C& %:S @#A&?,J%:( @#A&?.21?%%2J/>2NPQ02.$.J0,?/2C$O %S">#2$?,J%(">#2$.2CG21/0H2%U&3/0CK2%%[,$K, />?/%S">#2$?,J%(">#2$?.2%/0I2CJ0OO2.2,/0?M%2 ?1.$CC/>20.1$II$,M$+,J?.U0O?,J$,%U0O’!( S ">S C>)$-&-#):S ’C-#@ ; S ">( C>S$-&-#):( )#@# @;)#)#(#-;)#)#%2J0OO2.2,12$G2.?/$.C?.2J2O0,2JMU &-#):@#A ;&-CS#):@DS#A C&-CS#):@#A K0/>&)#) :@#A ;:@#A2 @2$I2/.01 I2?,0,@ $O />2 ?M$H2 1$,J0/0$,C0C0%%+C/.?/2J 0, \0@YT& F>2 NPQ02. $.J0,?/2C$O/K$M01+M01NPQ02.O+,1/0$,C?.2C>$K, 0,C$%0J?,J10.1%2#.2CG21/0H2%U&W+GG$C2%S">#2$ ?,J%(">#2$?.28A"A;)#S#(#!$1$,/0,+$+C?%$,@ />20.1$II$,M$+,J?.U#/>2,/>2)/>/$/>2A/> 1$%+I,C$O/>210.1%2$.J0,?/2C?.2J2/2.I0,2JMU />2 "!cA$/>/$/>2!.J 1$%+I,C $O/>2C$%0J $.J0,?/2C& D"/GI NPQ02.$.J0,?/2C$O/K$M01+M01G$%U,$I0?%C 3OK2J2O0,2?G$%U,$I0?%$OM06J2@.22"A#!$ "A ; S#(#!$K0/>/>2 "!CA$/>/$/>2!.J 1$%+I,C $O />2 C$%0J $.J0,?/2C ?C 0/C NPQ02. $.J0,?/2C#?,JC0I0%?.%UJ2O0,2?G$%U,$I0?%$OM06 J2@.22"A#!$K0/>/>2)/>/$/>2A/>1$%+I,C$O/>2 10.1%2$.J0,?/2C?C0/CNPQ02.$.J0,?/2C#/>2,/>2C2 /K$G$%U,$I0?%C?.2/>2C?I2&BCG210?%%U#O$./>2 />0.J $.J2. 1$,/0,+0/U 1$,J0/0$,C# />2 /K$ G$%U,$I0?%C%S">#2$?,J%(">#2$?.20J2,/01?%& W0I0%?./$/>21?C2C$O.2@+%?.F6I2C>2C0, D2II?!&S$OX2OY’((#O$.@2,2.?%F6I2C>2C#K2 >?H2/>2O$%%$K0,@%2II?#K>01> K0%%G%?U?, 0IG$./?,/.$%20, />2 G.$$O $O/>2 J0I2,C0$, O$.I+%?2$O/>2CG%0,2CG?12C $H2. @2,2.?% F6 I2C>2C& ;$##+@JF !?,J?CG%0,2 CG?12#"’#(#"###!$J2O0,2J$H2.!#1$,C0J2.? >$.0Q$,/?%,$,6M$+,J?.U162J@2K>01>1$,C0C/C$O% 0,/2.0$.2J@2C?,J>?C%DS12%%C?JA?12,//$0/& F>2,/>2#DS.$KC$O/>2NPQ02.$.J0,?/2C,2?./>2 162J@20,2?1>$O/>2C212%%CK0%%J2O0,2?,0J2,/01?% G$%U,$I0?%$O M06J2@.22 "’##$ "C22 \0@Y5$& W0I0%?.%U#1$,C0J2.?H2./01?%,$,6M$+,J?.U162J@2& F>2"DS1$%+I,C$O/>2NPQ02.$.J0,?/2C,2?./>2 162J@20,2H2.U?JA?12,/12%%K0%%J2O0,2?,0J2,/01?% G$%U,$I0?%$OM06J2@.22""#($21?C2O$.>$.0Q$,/?% ,$,6M$+,J?.U162J@2&W+GG$C2$S?,J$(?.2/>2/K$ %2O/I$C/?JA?12,/12%%C,2?./>2162J@2 K0/>$( M2,2?/>$S2G.2H0$+C?,?%UC0C#K2 [,$K/>?/#DS.$KC$O/>2NPQ02.$.J0,?/2C,2?. />2162J@20,$S ?,J$( K0%% J2O0,2 />2 C?I2 G$%U,$I0?%K0/>M06J2@.22"’##$2, K2C2%21//>2%2O/I$C/12%%0,/>2.2C/$O/>2 ?JA?12,/12%%C$O/>2162J@2&F>2,2K12%%K0%%M2 ?JA?12,/H2./01?%%U/$$S$.$(2.2O$.2#DS.$KC $O/>2NPQ02.$.J0,?/2C,2?./>2162J@20,/>2,2K 12%% K0%%J2O0,2/>2 C?I2 G$%U,$I0?%&NU />0C O?C>0$,#K21?,.+,/>.$+@>?%%/>212%%C#?,J/>+C ?%%/>2#DS.$KC$O/>2NPQ02.$.J0,?/2C,2?./>216 2J@2J2O0,2?,0J2,/01?%G$%U,$I0?%& ’ 57" CDEFGHIFFJ K!"L

第6潮 Dimensions of spline spaces over generl T-mishes 577 3 Dimension formula of spline spaces E-E-E.=V. (3) (N) over general T-meshes +=+.+座=+德(4) In this sertion,we will derive a dimensian Proof formula for the spline space s(m.)over a I)Eg.(1)holds obviously according to the general T-mesh whenm≥2a+1andn≥2p+l. definition of A:and a.. 3.1 Some notations for a T-mesh (I)Since every cell has two horizontal Before we derive the dimension formula.we boundary lines,each of which is part of some introduce some notations for a T-mesh as shown in horizontal cedge,and every horizontal nom Tab.1. boundary c-edge has A+1 adjacent cells,by the Tabs.1 Notations for a T-mesh contribution of boundary eedges,it follows that motations description E H mher of horiatal houndary edges 2F=A+ (+1). D mber of verticel boundary edges By Eq.(1).we have mhr f horiannia interinr le 2F-座-E=E. E er of wrtiral inierint age Similarly, A her of horiza boundary dges 2F-的-E.=E. B mamber of werticel boundary c-edues )Observe that A mdr of hotiamll worleudhry cules E-E-E,=(E-E)+(E-E,). B madr of werinl wrloundry®s Since E.-Ex and E.-E.represent the numbers of nalr of intetior ocs an the ith botioontal non- all interior T-vertices on all horizontal and vertical unly,j■L,a。Da cedges,respectively,it follows that maber of interor odges on the ith verticul not- 西 E-E一E=Vh. cundiry.i-1,…s (N)If there are no singular b-vertires in the E maeber of interior edges I-mesh.according to the simple connectivity,it 件 mber of interior coss vertes follows that I ms ol interinr T-wertices “=成+ mf interint wrtins Note that we can give a little perturbation to each mber of free b-vertices singular h-vertex to isolate the two cells W her of sirolar b-vertices intersected at it.Then we have m of boundary vertics V+V作=+ maber of ells in the mesh It is easy to know that the number of inner b Similar to Lemma 4.1 for a regular T-mesh in vertices is equal to the number of boundary edges Ref.[2].for a general T-mesh,we have the but not boundary cedges.Hence we have following topological cquations. 四+？=+ Lemma 3.1 Given a T-mesh with the notations in Tah.1.then 3.2 The dimension formula (I) Now we are ready to prove the dimension E formula for the spline space s(m.n,.)when 4=E (1) m之2m+1dn之23+1. (Π) Theorem 3.2 Given a general T-mesh and a 2F-E-E=E,2F-E-E=E.(2) corresponding spline space s(m.nB), (Ⅲ) suppose m2+1 andn28+1.then

H !"#$%&"’%(’.#0CC21/0$,!K2 K0%%J2.0H2?J0I2,C0$, O$.I+%?O$./>2CG%0,2CG?12#"’!(!"!#!!#$H2.? @2,2.?%F6I2C>!K>2,’ (("DS?,J(((#dS’#$%’5+5"’%&(’.+01#$&2 N2O$.2K2J2.0H2/>2J0I2,C0$,O$.I+%?!K2 0,/.$J+12C$I2,$/?/0$,CO$.?F6I2C>?CC>$K,0, F?MYS& 0+4GF ’$/?/0$,CO$.?F6I2C> ,$/?/0$,C J2C1.0G/0$, E:, ,+IM2.$O>$.0Q$,/?%M$+,J?.U2J@2C E:1 ,+IM2.$OH2./01?%M$+,J?.U2J@2C E, ,+IM2.$O>$.0Q$,/?%0,/2.0$.2J@2C E1 ,+IM2.$OH2./01?%0,/2.0$.2J@2C E):, ,+IM2.$O>$.0Q$,/?%M$+,J?.U162J@2C E):1 ,+IM2.$OH2./01?%M$+,J?.U162J@2C E), ,+IM2.$O>$.0Q$,/?%,$,6M$+,J?.U162J@2C E)1 ,+IM2.$OH2./01?%,$,6M$+,J?.U162J@2C ’@ ,+IM2.$O0,/2.0$.2J@2C$,/>2@/>>$.0Q$,/?%,$,6 M$+,J?.U162J@2!@;S!$!E), (@ ,+IM2.$O0,/2.0$.2J@2C$,/>2@/> H2./01?%,$,6 M$+,J?.U162J@2!@;S!$!E)1 E ,+IM2.$O0,/2.0$.2J@2C FD ,+IM2.$O0,/2.0$.1.$CC0,@H2./012C F* ,+IM2.$O0,/2.0$.F6H2./012C F ,+IM2.$O0,/2.0$.H2./012C F:* ,+IM2.$OO.22M6H2./012C F:/ ,+IM2.$OC0,@+%?.M6H2./012C F: ,+IM2.$OM$+,J?.UH2./012C G ,+IM2.$O12%%C0,/>2I2C> W0I0%?./$D2II?T&SO$.?.2@+%?.F6I2C>0, X2OY%(&!O$.?@2,2.?% F6I2C>!K2>?H2/>2 O$%%$K0,@/$G$%$@01?%2b+?/0$,C& ;$##+ HJF K0/> />2 ,$/?/0$,C0,F?MYS!/>2, ""# % E), @;S ’@ ;E,!% E)1 @;S (@ ;E1$%JC$MH0$+C%U?11$.J0,@/$/>2 J2O0,0/0$,$O’-?,J(-?C /K$ >$.0Q$,/?% M$+,J?.U%0,2C!2?1>$O K>01>0CG?./$OC$I2 >$.0Q$,/?% 162J@2! ?,J 2H2.U >$.0Q$,/?% ,$,6 M$+,J?.U162J@2>?C’- DS?JA?12,/12%%C!MU/>2 1$,/.0M+/0$,$OM$+,J?.U162J@2C!0/O$%%$KC/>?/ (G ;E): , D% E), @;S "’@DS#?H2 (GCE): , CE), ;E,?/ ECE), CE)1 ; "E, CE),#D"E1 CE)1#2,+IM2.C$O ?%%0,/2.0$.F6H2./012C$,?%%>$.0Q$,/?%?,JH2./01?% 162J@2C!.2CG21/0H2%U!0/O$%%$KC/>?/ ECE), CE)1 ;F* 2.2?.2,$C0,@+%?.M6H2./012C0,/>2 F6I2C>!?11$.J0,@/$/>2C0IG%21$,,21/0H0/U!0/ O$%%$KC/>?/ F: ;E: , DE: 1?/K21?,@0H2?%0//%2G2./+.M?/0$,/$2?1> C0,@+%?. M6H2./2Z /$ 0C$%?/2 />2 /K$ 12%%C 0,/2.C21/2J?/0/&F>2,K2>?H2 F: DF: / ;E: , DE: 1?//>2,+IM2.$O0,,2.M6 H2./012C0C2b+?%/$/>2,+IM2.$OM$+,J?.U2J@2C M+/,$/M$+,J?.U162J@2C&:2,12K2>?H2 F: * DF: / ;E): , DE): 12J0I2,C0$, O$.I+%?O$./>2CG%0,2CG?12#"’!(!"!#!!#K>2, ’ (("DS?,J(((#DS?,J? 1$..2CG$,J0,@ CG%0,2 CG?12 #"’!(!"!#!!#! C+GG$C2’ (("DS?,J(((#DS!/>2, K"2 40I2,C0$,C$OCG%0,2CG?12C$H2.@2,2.?%F6I2C>2C 577

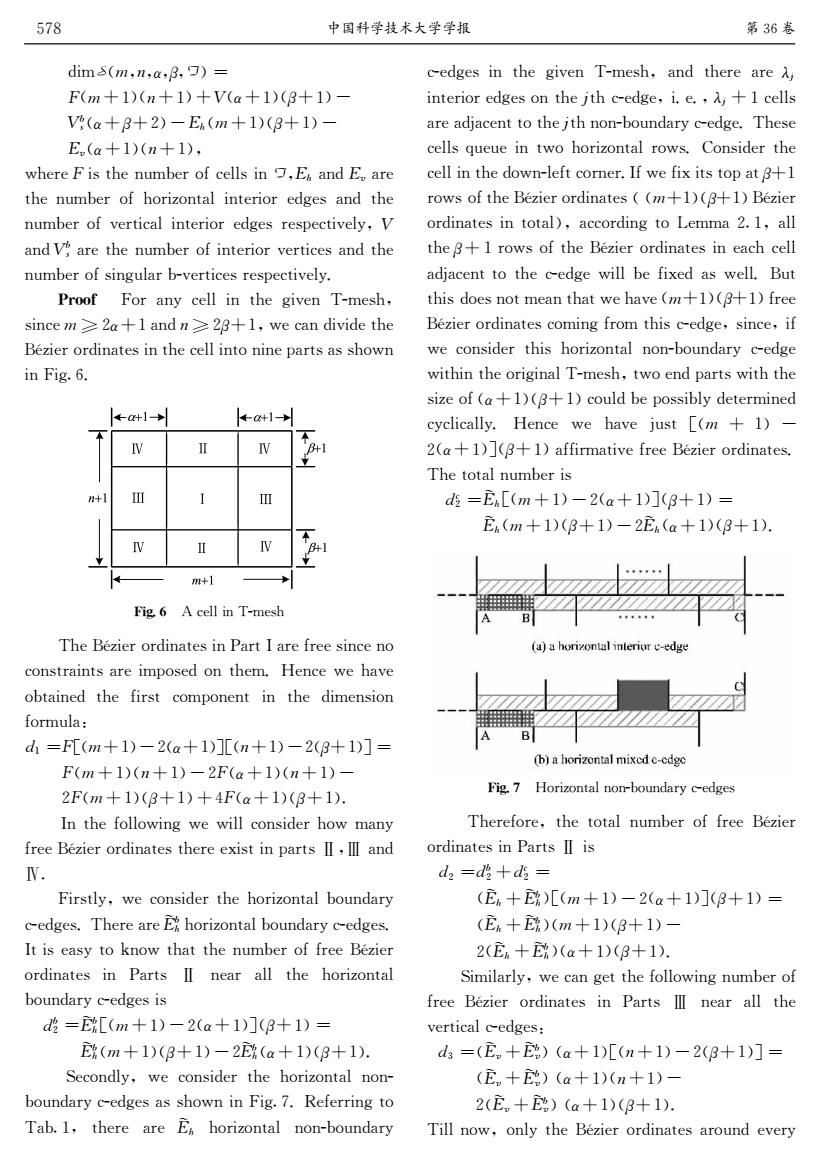
578 中回科学技术大季学摄第36卷 im5(m,n,a,3,)= c-edges in the given T-mesh.and there are A F(m+1)(n十1)+V(a+1)(3+1)一 interior edges on the jth r-edge,i.e..A+1 rells V(a+B+2)-E(m十1)(3+1)- are adjacent to the jth norrboundary e-edge.These E(a+1)(w+1), cells queue in two horizontal rows.Consider the where F is the numher of cells in Es and E.are cell in the down-left comer.If we fix its top at 8+1 the number of horizontal interior edges and the rows of the Bezier ordinates ((+1)(1)Bezier number of vertical interior edges respectively,V ordinates in total),according to Lemma 2.1.all and V are the number of interior vertices and the the +1 tows of the Bezier ordinates in each cell numher of singular h-vertices respectively. adjacent to the eedge will be fixed as well.But Proof For any cell in the given T-mesh, this does not mean that we have (m+1)(+1)free since m≥2u十1andn≥23+1，we can divide the Bezier ordinates coming from this e-edge.since.if Bezier ordinates in the cell into nine parts as shown we consider this horizontal nomboundary cedge in Fig.6. within the original T-mesh.two end parts with the size of (a+1)(+1)could be possibly determined 女+l+州 +at1→ cyelically,Hence we have just [(m 1) W 2(a+1)(8+1)affirmative free Bezier ordinates. The total number is n+】 1加 d-E[(m+1)-2(m+1)](3+1)= E(m+1)(9+1)-2E.(a+1)(3+1). Ⅱ W 41 优+I Fig 6 A coll in T-mish The Bezier ordinates in Part I are free sinre no la)2 horxumal c-edpe constraints are imposed on them.Hence we have obtained the first component in the dimension formula: d4=(m+1)-2(a+1)][(n+1)-29+1)]= hia3i市mal mxol e-ee F(m+1)(n+1)-2F(a+1)(n+1)- 2F(m+1)(3+1》+4Fa+1)(3+1). Fi 7 Harizontal nan-boumndary cedges In the following we will consider how many Therefore,the total number of free Bezier free Bezier ordinates there exist in parts II,II and ordinates in Parts II is N. d,=十诸= Firstly,we consider the horizontal boundary (E+)[(m+1)-2(a+1)](0+1)= c-edges.There are Et horizontal boundary c-edges. (E+含)(m+1)(3+1) It is easy to know that the number of free Bezier 2(E+)(a+1)(3+1). ordinates in Parts II near all the horizontal Similarly,we can get the following number of houndary c-edges is free Bezier ordinates in Parts near all the d-[(m+1)-2(a+1)](3+1) vertical cedges: (m+1)(3+1)-2(a+1)(9+1). d&=(E.+)(a+1)[(m+1)-2(3+1)]= Secondly.we consider the horizontal nou- (E.+)(a+1)(a+1) houndary c-edges as shown in Fig.7.Referring to 2(E+E)(a+1)(3+1). Tah.1,there are E horizntal non-boundary Till now.only the Bezier ordinates amund every

J0I#!’"("""#"!#; G!’DS#!(DS#DF!"DS#!#DS#C F: /!"D#D(#CE,!’DS#!#DS#C E1!"DS#!(DS#" K>2.2G0C/>2,+IM2.$O12%%C0,!"E,?,JE1?.2 />2,+IM2.$O>$.0Q$,/?%0,/2.0$.2J@2C?,J/>2 ,+IM2.$OH2./01?%0,/2.0$.2J@2C.2CG21/0H2%U"F ?,JF: /?.2/>2,+IM2.$O0,/2.0$.H2./012C?,J/>2 ,+IM2.$OC0,@+%?.M6H2./012C.2CG21/0H2%U& L.’’( \$.?,U12%%0,/>2@0H2, F6I2C>" C0,12’ (("DS?,J(((#DS"K21?,J0H0J2/>2 NPQ02.$.J0,?/2C0,/>212%%0,/$,0,2G?./C?CC>$K, 0,\0@Y"& D"/GM -12%%0,F6I2C> F>2NPQ02.$.J0,?/2C0,_?./3?.2O.22C0,12,$ 1$,C/.?0,/C?.20IG$C2J$,/>2I&:2,12K2>?H2 $M/?0,2J/>2O0.C/1$IG$,2,/0, />2 J0I2,C0$, O$.I+%?$ 4S ;G%!’DS#C(!"DS#&%!(DS#C(!#DS#&; G!’DS#!(DS#C(G!"DS#!(DS#C (G!’DS#!#DS#DTG!"DS#!#DS#2O$%%$K0,@K2K0%%1$,C0J2.>$K I?,U O.22NPQ02.$.J0,?/2C/>2.22Z0C/0,G?./C#"$?,J %& \0.C/%U"K21$,C0J2./>2>$.0Q$,/?%M$+,J?.U 162J@2C&F>2.2?.2E): ,>$.0Q$,/?%M$+,J?.U162J@2C& 3/0C2?CU/$[,$K/>?//>2,+IM2.$OO.22NPQ02. $.J0,?/2C0, _?./C # ,2?. ?%%/>2 >$.0Q$,/?% M$+,J?.U162J@2C0C 4: ( ;E): ,%!’DS#C(!"DS#&!#DS#; E): ,!’DS#!#DS#C(E): ,!"DS#!#DS#2>$.0Q$,/?%,$,6 M$+,J?.U162J@2C?CC>$K,0,\0@Y7&X2O2..0,@/$ F?MYS"/>2.2 ?.2 E), >$.0Q$,/?% ,$,6M$+,J?.U 162J@2C0,/>2@0H2, F6I2C>"?,J/>2.2?.2’@ 0,/2.0$.2J@2C$,/>2@/>162J@2"0&2&"’@ DS12%%C ?.2?JA?12,//$/>2@/>,$,6M$+,J?.U162J@2&F>2C2 12%%Cb+2+20,/K$>$.0Q$,/?%.$KC&V$,C0J2./>2 12%%0,/>2J$K,6%2O/1$.,2.&3OK2O0Z0/C/$G?/#DS .$KC$O/>2NPQ02.$.J0,?/2C!!’DS#!#DS#NPQ02. $.J0,?/2C0,/$/?%#"?11$.J0,@/$D2II?(YS"?%% />2#DS.$KC$O/>2NPQ02.$.J0,?/2C0,2?1>12%% ?JA?12,//$/>2162J@2K0%%M2O0Z2J?CK2%%&N+/ />0CJ$2C,$/I2?,/>?/K2>?H2!’DS#!#DS#O.22 NPQ02.$.J0,?/2C1$I0,@O.$I/>0C162J@2"C0,12"0O K21$,C0J2./>0C>$.0Q$,/?%,$,6M$+,J?.U162J@2 K0/>0,/>2$.0@0,?%F6I2C>"/K$2,JG?./CK0/>/>2 C0Q2$O!"DS#!#DS#1$+%JM2G$CC0M%UJ2/2.I0,2J 1U1%01?%%U& :2,12 K2 >?H2 A+C/ %!’ D S#C (!"DS#&!#DS#?OO0.I?/0H2O.22NPQ02.$.J0,?/2C& F>2/$/?%,+IM2.0C 4. ( ;E),%!’DS#C(!"DS#&!#DS#; E),!’DS#!#DS#C(E),!"DS#!#DS#2.2O$.2"/>2/$/?%,+IM2.$OO.22NPQ02. $.J0,?/2C0,_?./C#0C 4( ;4: ( D4. ( ; !E), DE): ,#%!’DS#C(!"DS#&!#DS#; !E), DE): ,#!’DS#!#DS#C (!E), DE): ,#!"DS#!#DS#2O$%%$K0,@,+IM2.$O O.22 NPQ02. $.J0,?/2C0, _?./C $ ,2?.?%%/>2 H2./01?%162J@2C$ 4! ;!E)1 DE): 1#!"DS#%!(DS#C(!#DS#&; !E)1 DE): 1#!"DS#!(DS#C (!E)1 DE): 1#!"DS#!#DS#2NPQ02.$.J0,?/2C?.$+,J2H2.U 578 CDEFGHIFFJ K!"L

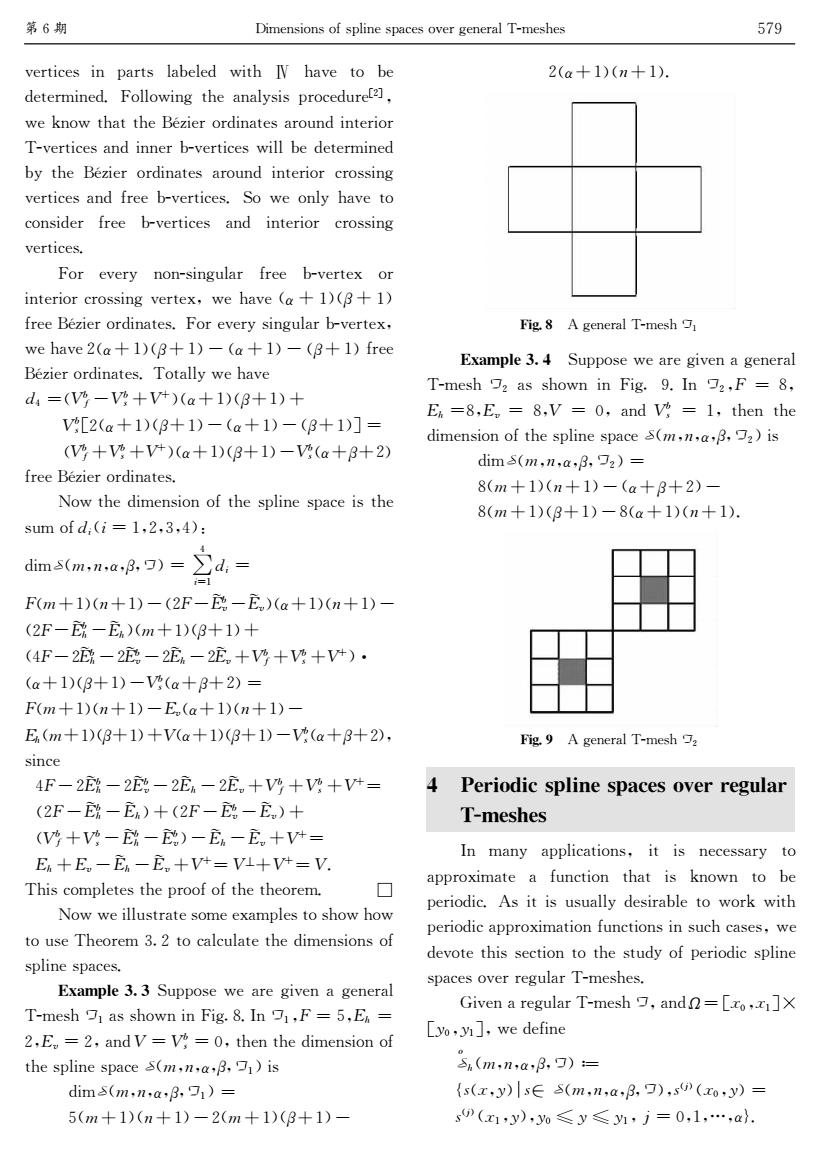
第B湘 Dimensions of spline spaces over general T-meshes 579 vertices in parts labeled with N have to he 2(a+12(n+1). determinod.Following the analysis procedure, we know that the Bezier ordinates around interior T-vertices and inner b-vertices will be determined by the Bezier ordinates around interior crossing vertices and free b-vertices.So we only have to consider free h-vertices and interinr croxsing vertices For every non-singular free b-vertex or interior crossing vertex.we have (g+1)(2+1) free Bezier ordinates.For every singular b-vertex. 下ig8 A general T-mesh we have2(a+1)(9+1》-(a+1)-(3+1》free Example 3.4 Suppoxse we are given a general Bezier ordinates.Totally we have T-mesh as shown in Fig,9.In :.8, d=(-g++)(a+1)(8+1)+ E=8.E=8.V =0,and V=1,then the [2(a十1)3+1)-(a+1)-(3+1)]= dimension of the spline space &(m.n)is (9+8+)(a+1)(3+1)-(a++2) dims(mn:)= free Bezier ordinates. 8(m+1)(n+1)-(a+3+2)- Now the dimension of the spline spare is the 8(m十1)(3+1》-8(a+1)(n+1). sum of d,i=1,2,3,4): dims(m)=d.= =1 F(m+1)(m+1)一(2F--E.)(a+1)(m+1)- (2F--E)(m十1)(3+1)十 (4F-2E-2E-2E-2E+9++V)· (a+1)(g+1)-(a+3+2)= F(m+1)(n+1)-E(a+1)(#+1)- E(m+1)(0+1)+a+10+1)-(a+3+2). Fig.9 A general T-mesh since 4F-2-2E-2E-2E.+%++V- 4 Periodic spline spaces over regular (2F--E)+(2F-E-E)+ T-meshes (V+V-m-E)-E-E++ Es+E-E-E+V+=V+V+=V. In many applications.it is nerexsary to ▣ approximate a function that is known to he This completes the proof of the theoremn. periodic.As it is usually desirable to work with Now we illustrate some examples to show how periodic approximation functions in such cases,we to use Theorem 3.2 to calculate the dimensions of devote this section to the study of periodic spline spline spaces. spaces over regular T'-meshes. Example 3.3 Suppose we are given a general Given a regular T-mesh and-[e.]x T-mesh as shown in Fig.8.In 1.F=5.E= 2.E -2,and V-V-0,then the dimension of [yo,y ]we define the spline space s(m.n8.1)is ,(m,na,月，)= dim(m,,c3,1)= {s(r,y)s∈(mn,a,3,),sp(x,y》= 5(m+1)(n+1)-2(m+1)(0+1)一 (1y)为≤y≤y…i=01u小

L21?%%# ) , />2>$.0Q$,/?%%UG2.0$J01CG%0,2CG?12 $H2./>2.2@+%?. F6I2C> !2 H2./01?%%U G2.0$J01 CG%0,2 CG?12 # ) 1"’!(!"!#!!#?,J/>2 M06J0.21/0$,?%G2.0$J01 CG%0,2CG?12# ) "’!(!"!#!!#$H2./>2.2@+%?.F6 I2C>!$.0Q$,/?%%U G2.0$J01CG%0,2!/>2NPQ02.$.J0,?/2C0,_?./$?,J _?./ % ".2O2..0,@/$ \0@Y"#,2?./>2H2./01?% M$+,J?.U2J@2C"162J@2C#?.20,/2..2%?/2J&L>2, 1$+,/0,@/>2,+IM2.$OO.22NPQ02.$.J0,?/2C!K2 1?,0I?@0,2M2,J0,@/>2F6I2C>?,J@%+0,@/>2 /K$H2./01?%M$+,J?.U@.0J%0,2C/$@2/>2.&F>2, />2M$+,J?.UH2./012C$,/>2H2./01?%M$+,J?.U@.0J %0,2C "2Z12G//>2O$+.1$.,2.H2./012C#1?, M2 1$,C0J2.2J?C0,/2.0$.H2./012C!/>2O$+.1$.,2. H2./012C 1?, M2 1$,C0J2.2J ?C /K$ M$+,J?.U H2./012C!H2./01?%M$+,J?.U2J@2CM21$I2H2./01?% 0,/2.0$.2J@2C!?,J,2K $1.$CC0,@H2./012C%?,J ,2K $H2./01?%162J@2C%?%$,@/>2H2./01?%M$+,J?.U %0,2C1?,M20,/.$J+12J&:2,12K2>?H2!K0/>/>2 ,$/?/0$,0,/>2G.$$O$OF>2$.2I!&(! 4S ;G&"’DS#C(""DS#’&"(DS#C("#DS#’( 4( ; "E), DE: ,#&"’DS#C(""DS#’"#DS#( 4! ; "E)1 D&E)1#""DS#&"(DS#C("#DS#’( 4T ; "FD DF: D&FD C&F:#""DS#"#DS#2,+IM2.$O0,1.2?C2JH2./01?%0,/2.0$.16 2J@2C K>01> 1$I2O.$I />2 H2./01?% M$+,J?.U 2J@2C!&FD 0C/>2,+IM2.$O0,1.2?C2J1.$CC0,@ H2./012C!?,J &F: 0C />2 ,+IM2. $O .2J+12J M$+,J?.UH2./012C&3/0C2?CU/$[,$K/>?/&E)1 ; &FD DS!&F: ;E: 1!?,J&FD0C2b+?%/$/>2,+IM2. $OG?0.C$O/>2M$+,J?.UH2./012CK0/>/>2C?I2 261$$.J0,?/2C$,/>2H2./01?%M$+,J?.U@.0J%0,2C "2Z12G//>2O$+.1$.,2.H2./012C#& F>+C />2 J0I2,C0$, $O />2 CG%0,2 CG?12 # ) ,"’!(!"!#!!#0C J0I# ) ,"’!(!"!#!!#; % T -;S 4- ; G"’DS#"(DS#C "(GCE: , CE),#"’DS#"#DS#C "(GC&E)1 CE)1#""DS#"(DS#D "TGC(E: , C(E), C(&E)1 C(E)1 DFD D F: D&FD C&F:#""DS#"#DS#; G"’DS#"(DS#CE,"’DS#"#DS#C "E1 D&E1#""DS#"(DS#D "FD&F#""DS#"#DS#; J0I#"’!(!"!#!!#C&E1""DS#"(DS#D &F""DS#"#DS#! K>2.2&E1 ; E: 1 C &FDC S0C/>2,+IM2.$O 0,1.2?C2JH2./01?%0,/2.0$.2J@2C!?,J&F ; E: 1 C &FDC( ; &E1 CS0C/>2,+IM2.$O0,1.2?C2J 0,/2.0$.H2./012C&\$.2Z?IG%2!0,\0@YS)!G ;7! E, ;7!E1 ;5!E: 1 ;"!F ;"!?,J&FD;S!/>2,! &E1 ;"CSCS;T!&F ;TCS;!2>$.0Q$,/?%%UG2.0$J01 CG%0,2 CG?12 # ) ,"’!(!"!#!!#! K2 >?H2 />2 O$%%$K0,@/>2$.2I& 02$’.$#IJF ?,J? 1$..2CG$,J0,@ >$.0Q$,/?%%U G2.0$J01CG%0,2 CG?12 # ) ,"’!(!"!#!!#!C+GG$C2’(("dS?,J(((#dS! />2, J0I# ) ,"’!(!"!#!!#; J0I#"’!(!"!#!!#C&E1""DS#"(DS#D &F""DS#"#DS#! K>2.2&E1 ;E: 1C&FD 1 CS!&F ;&E1CS(E: 10C/>2 ,+IM2.$OH2./01?%M$+,J?.U2J@2C!?,J&FD 10C/>2 ,+IM2.$OG?0.C$O/>2M$+,J?.UH2./012CK0/>/>2 C?I2261$$.J0,?/2C$,/>2H2./01?%M$+,J?.U@.0J %0,2C"2Z12G//>2O$+.1$.,2.H2./012C#& W0I0%?.%U!O$./>2H2./01?%%U G2.0$J01CG%0,2 CG?12# ) 1"’!(!"!#!!#!K2>?H2 02$’.$#IJ@ ?,J? 1$..2CG$,J0,@ H2./01?%%U G2.0$J01 CG%0,2 CG?12 58) CDEFGHIFFJ K!"L

# ) 1!’"("""#"!#"C+GG$C2’(("dS?,J(((#dS" />2, J0I# ) 1!’"("""#"!#; J0I#!’"("""#"!#C&E,!’DS#!#DS#D &F!"DS#!#DS#" K>2.2&E, ;E: ,C&FD , CS"&F ;&E,CS$E: ,0C/>2 ,+IM2.$O>$.0Q$,/?%M$+,J?.U2J@2C"?,J&FD , 0C />2,+IM2.$OG?0.C$O/>2M$+,J?.UH2./012CK0/> />2C?I2>61$$.J0,?/2C$,/>2>$.0Q$,/?%M$+,J?.U @.0J%0,2C!2Z12G//>2O$+.1$.,2.H2./012C#& \$./>2 M06J0.21/0$,?%G2.0$J01CG%0,2CG?12 # ) !’"("""#"!#"K2>?H2/>2O$%%$K0,@/>2$.2I& 02$’.$#IJH ?,J? 1$..2CG$,J0,@M06J0.21/0$,?%G2.0$J01CG%0,2CG?12 # ) !’"("""#"!#"C+GG$C2’(("dS?,J(((#dS" />2, J0I# ) !’"("""#"!#; J0I#!’"("""#"!#D&F!"DS#!#DS#C &E,!’DS#!#DS#C&E1!"DS#!(DS#" K>2.2&E, ;E: , C&FD , CS"&E1 ;E: 1 C&FD 1 CS" &F ; &E, D&E1 CS2 ,+IM2.$O >$.0Q$,/?% M$+,J?.U 2J@2C ?,J />2 ,+IM2.$OH2./01?%M$+,J?.U2J@2C.2CG21/0H2%U" &FD ,0C/>2,+IM2.$OG?0.C$O/>2M$+,J?.UH2./012C K0/>/>2C?I2 >61$$.J0,?/2C $, />2 >$.0Q$,/?% M$+,J?.U @.0J %0,2C !2Z12G/ />2 O$+. 1$.,2. H2./012C#"?,J&FD 1 0C/>2,+IM2.$OG?0.C$O/>2 M$+,J?.UH2./012CK0/>/>2C?I2261$$.J0,?/2C$, />2H2./01?%M$+,J?.U@.0J%0,2C !2Z12G//>2O$+. 1$.,2.H2./012C#& K :’%,*0CG?G2.G.2C2,/C/>2J0I2,C0$,O$.I+%?O$. />2CG%0,2CG?12#!’"("""#"!#$H2.?@2,2.?%F6 I2C>! K>2,’ (("DS?,J( ((#DS2 G2.0$J01CG%0,2 CG?12C J2O0,2J $H2. .2@+%?. F6 I2C>2C?.2J0C1+CC2J?C K2%%&F>2C2J0I2,C0$, O$.I+%?2K0%%M2+C2O+%0,/>2C+.O?12 I$J2%0,@ K0/>/>2C2CG%0,2C"MU/>2,K21?,J2C0@,C$I2 %@$$J&M?C0CO+,1/0$,C&L2K0%%?JJ.2CC/>2M?C0C O+,1/0$,1$,C/.+1/0$,$O/>2CG%0,2CG?12C$H2.F6 I2C>2C0,O+/+.2G?G2.& L>2,’(("DS?,J(((#DS"/>2J0I2,C0$, O$.I+%?$O#!’"("""#"!#0C?%0,2?.1$IM0,?/0$, $O G"E,"E1 ?,J F" K>2.2 />2 1$IM0,?/0$,?% 1$2OO0102,/CJ2G2,J$,%U$,’"("""?,J#0,[ $O/>2 J0I2,C0$, O$.I+%? ?C ? K20@>/2J B+%2. O$.I+%?& 3O K2 .2I$H2 />2 1$,C/.?0,/C’ (("DS?,J(((#DS"/>2NPQ02. $.J0,?/2C0,2H2.U12%%1?,,$/M2J0H0J2J0,/$O$+. G?./C?,J?%%/>2NPQ02.$.J0,?/2C K0%%0,O%+2,12 2?1>$/>2.&F>2J0I2,C0$,O$.I+%? I?UO?0%/$ >$%J&-@2,2.?%J0I2,C0$,O$.I+%?K0/>$+//>2C2 1$,C/.?0,/C0CC/0%%+,?H?0%?M%2&3,O?1/"K22H2,J$ ,$/[,$K K>2/>2./>2J0I2,C0$,.2%02C$,/>2 @2$I2/.U $O />2 F6I2C> $. ,$/&3, O+/+.2 .2C2?.1>"K2K0%%0,H2C/0@?/2/>2C2G.$M%2IC& C$($.$%,$& ’S(W2J2.M2.@F L"=>2,@*"N?[2,$H-"2/?%&F6CG%0,2C ?,JF6’;XVVC’*(&-V‘ F.?,C?1/0$,C$,01C" ())!"((!!#)S"S6S7(& ’((4B’2C’*(&*$+.,?% $OV$IG+/?/0$,?%?,J-GG%02J ‘?/>2I?/01C"())""S9T !(#)("76(8!& ’!(W2J2.M2.@F L"V?.J$,4D"\0,,0@?,01C"())T"(!!!#)(7"6(8!& ’T(\?.0,2J&&W?,\.?,10C1$)‘$.@?,^?+OI?,, _+M%0C>2.C"())(& K"2 40I2,C0$,C$OCG%0,2CG?12C$H2.@2,2.?%F6I2C>2C 58S