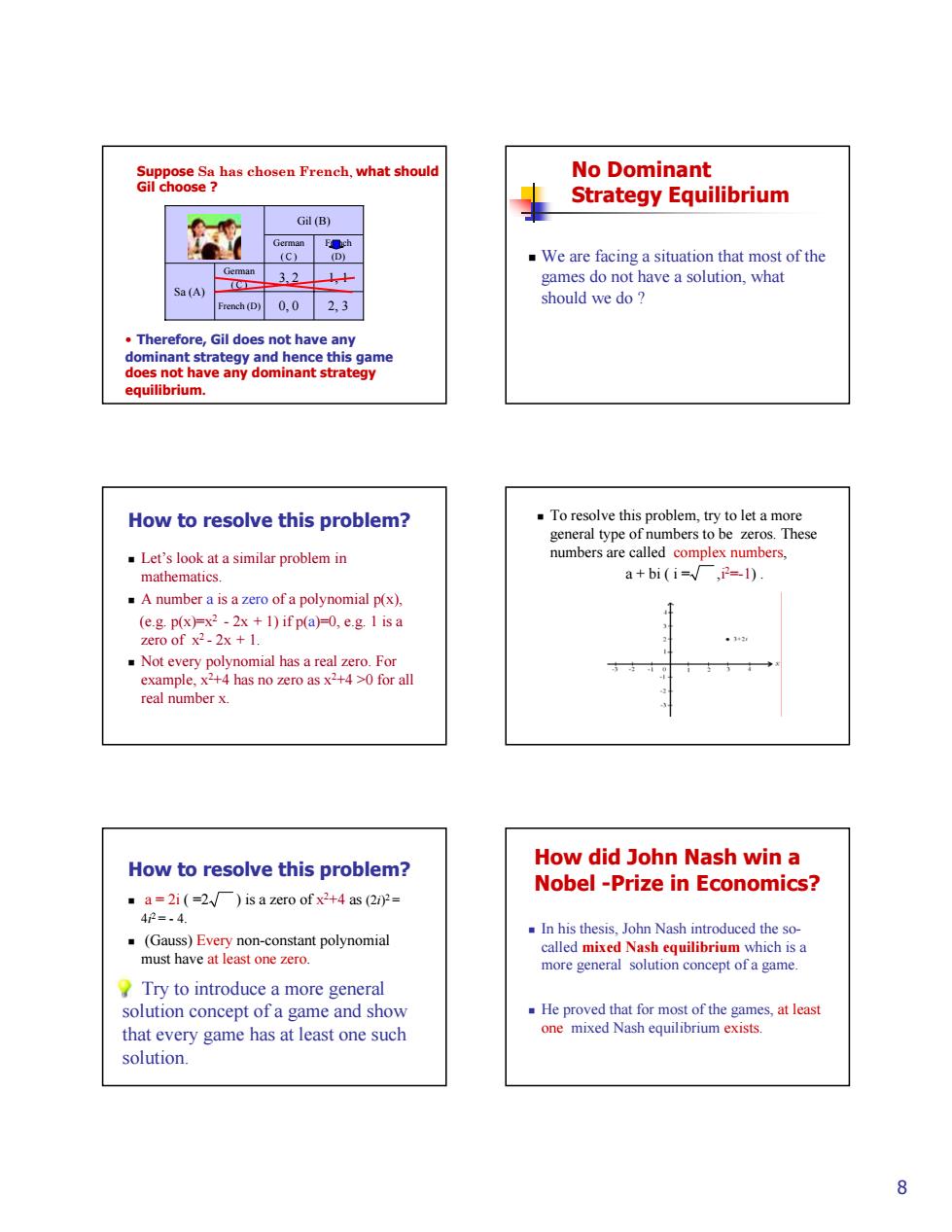
Suppose Sa has chosen French,what should No Dominant Gil choose Strategy Equilibrium G1(B) German Fhch (C) (D) We are facing a situation that most of the (C 32 + games do not have a solution,what Sa(A) French(D) 0,0 2,3 should we do Therefore,Gil does not have any dominant strategy and hence this game does not have any dominant strategy equilibrium. How to resolve this problem? To resolve this problem,try to let a more general type of numbers to be zeros.These Let's look at a similar problem in numbers are called complex numbers, mathematics. a+bi(i=√厂，i2=l). A number a is a zero of a polynomial p(x), (e.g.p(x)=x2-2x +1)ifp(a)=0,e.g.1 is a zero of x2-2x +1. Not every polynomial has a real zero.For example,x2+4 has no zero as x2+4 >0 for all real number x. How did John Nash win a How to resolve this problem? Nobel -Prize in Economics? ■a=2i(=2√厂)is a zero ofx2+4as(2iy= 42=.4. In his thesis.John Nash introduced the so- (Gauss)Every non-constant polynomial called mixed Nash equilibrium which is a must have at least one zero. more general solution concept of a game. Try to introduce a more general solution concept of a game and show He proved that for most of the games,at least that every game has at least one such one mixed Nash equilibrium exists. solution. 88 Suppose Sa has chosen French, what should Gil choose ? French (D) 0, 0 2, 3 3, 2 1, 1 German (Ｃ) Sa (A) French (D) German (Ｃ) Gil (B) • Therefore, Gil does not have any dominant strategy and hence this game does not have any dominant strategy equilibrium. No Dominant Strategy Equilibrium „ We are facing a situation that most of the games do not have a solution, what should we do ? How to resolve this problem? „ Let’s look at a similar problem in mathematics. „ A number a is a zero of a polynomial p(x), (e.g. p(x)=x2 - 2x + 1) if p(a)=0, e.g. 1 is a zero of x2 - 2x + 1. „ Not every polynomial has a real zero. For example, x2+4 has no zero as x2+4 >0 for all real number x. „ To resolve this problem, try to let a more general type of numbers to be zeros. These numbers are called complex numbers, a + bi ( i = ,i 2=-1) . How to resolve this problem? „ a = 2i ( =2 ) is a zero of x2+4 as (2i)2 = 4i 2 = - 4. „ (Gauss) Every non-constant polynomial must have at least one zero. Try to introduce a more general solution concept of a game and show that every game has at least one such solution. How did John Nash win a Nobel -Prize in Economics? „ In his thesis, John Nash introduced the so￾called mixed Nash equilibrium which is a more general solution concept of a game. „ He proved that for most of the games, at least one mixed Nash equilibrium exists