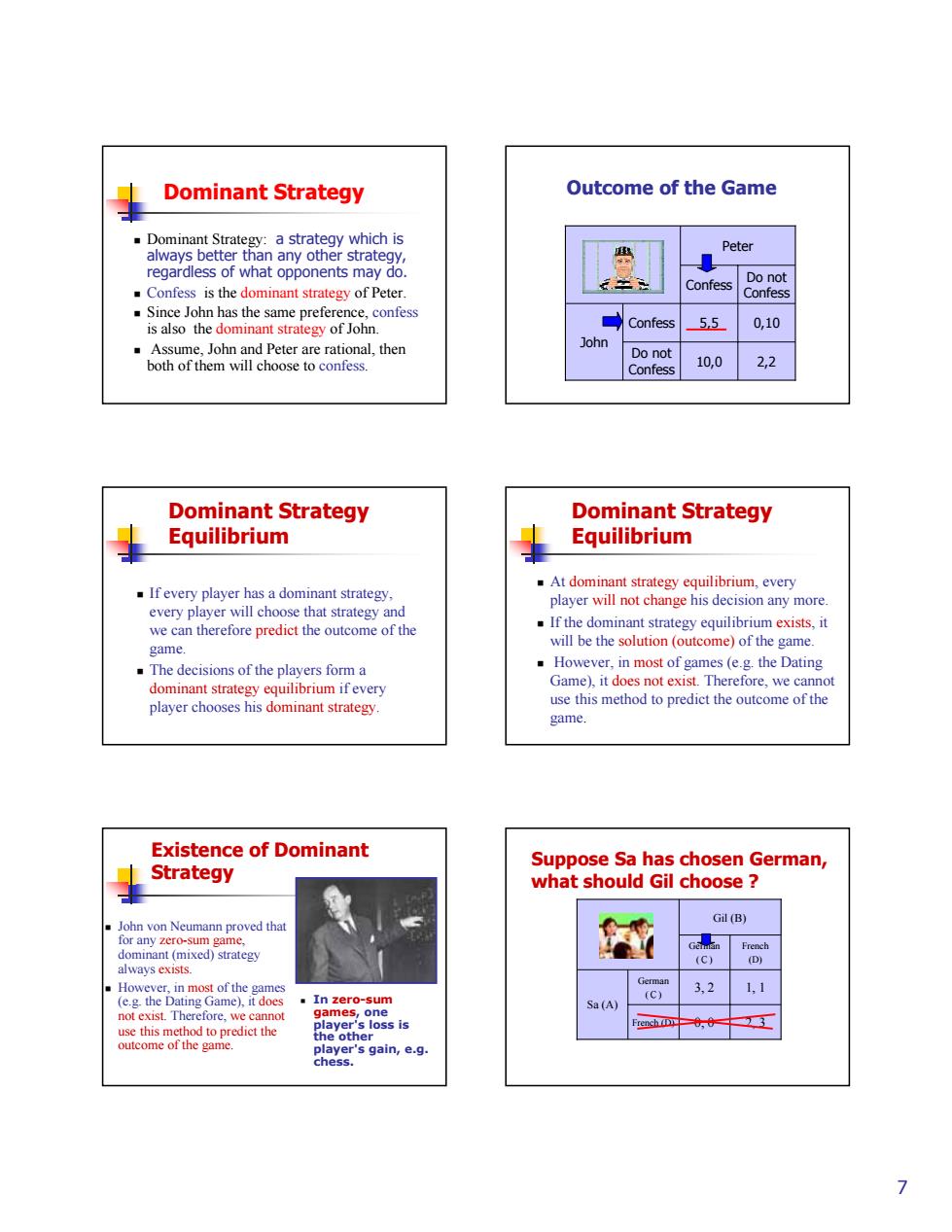
Dominant Strategy Outcome of the Game Dominant Strategy:a strategy which is Peter always better than any other strategy, regardless of what opponents may do. Do not Confess is the dominant strategy of Peter. Confess Confess Since John has the same preference,confess is also the dominant strategy of John. Confess 5.5 0,10 Assume,John and Peter are rational,then John Do not both of them will choose to confess. Confess 10,0 2,2 Dominant Strategy Dominant Strategy Equilibrium Equilibrium At dominant strategy equilibrium,every If every player has a dominant strategy, player will not change his decision any more. every player will choose that strategy and we can therefore predict the outcome of the If the dominant strategy equilibrium exists,it game. will be the solution (outcome)of the game. The decisions of the players form a However,in most of games (e.g.the Dating dominant strategy equilibrium if every Game),it does not exist.Therefore,we cannot player chooses his dominant strategy. use this method to predict the outcome of the game. Existence of Dominant Suppose Sa has chosen German, Strategy what should Gil choose John von Neumann proved that Gil (B) for any zero-sum game, G French dominant(mixed)strategy (C) (D) always exists. However,in most of the games German (e.g.the Dating Game),it does .In zero-sum (C) 3,2 1.1 Sa(A) not exist.Therefore,we cannot 8g28390sis French(D use this method to predict the outcome of the game. the other player's gain,e.g. chess. 77 Dominant Strategy „ Dominant Strategy: a strategy which is always better than any other strategy, regardless of what opponents may do. „ Confess is the dominant strategy of Peter. „ Since John has the same preference, confess is also the dominant strategy of John. „ Assume, John and Peter are rational, then both of them will choose to confess. Outcome of the Game 10,0 2,2 Do not Confess Confess 5,5 0,10 John Do not Confess Confess Peter Dominant Strategy Equilibrium „ If every player has a dominant strategy, every player will choose that strategy and we can therefore predict the outcome of the game. „ The decisions of the players form a dominant strategy equilibrium if every player chooses his dominant strategy. Dominant Strategy Equilibrium „ At dominant strategy equilibrium, every player will not change his decision any more. „ If the dominant strategy equilibrium exists, it will be the solution (outcome) of the game. „ However, in most of games (e.g. the Dating Game), it does not exist. Therefore, we cannot use this method to predict the outcome of the game. Existence of Dominant Strategy „ John von Neumann proved that for any zero-sum game, dominant (mixed) strategy always exists. „ However, in most of the games (e.g. the Dating Game), it does not exist. Therefore, we cannot use this method to predict the outcome of the game. „ In zero-sum games, one player's loss is the other player's gain, e.g. chess. Suppose Sa has chosen German, what should Gil choose ? French (D) 0, 0 2, 3 3, 2 1, 1 German (Ｃ) Sa (A) French (D) German (Ｃ) Gil (B)