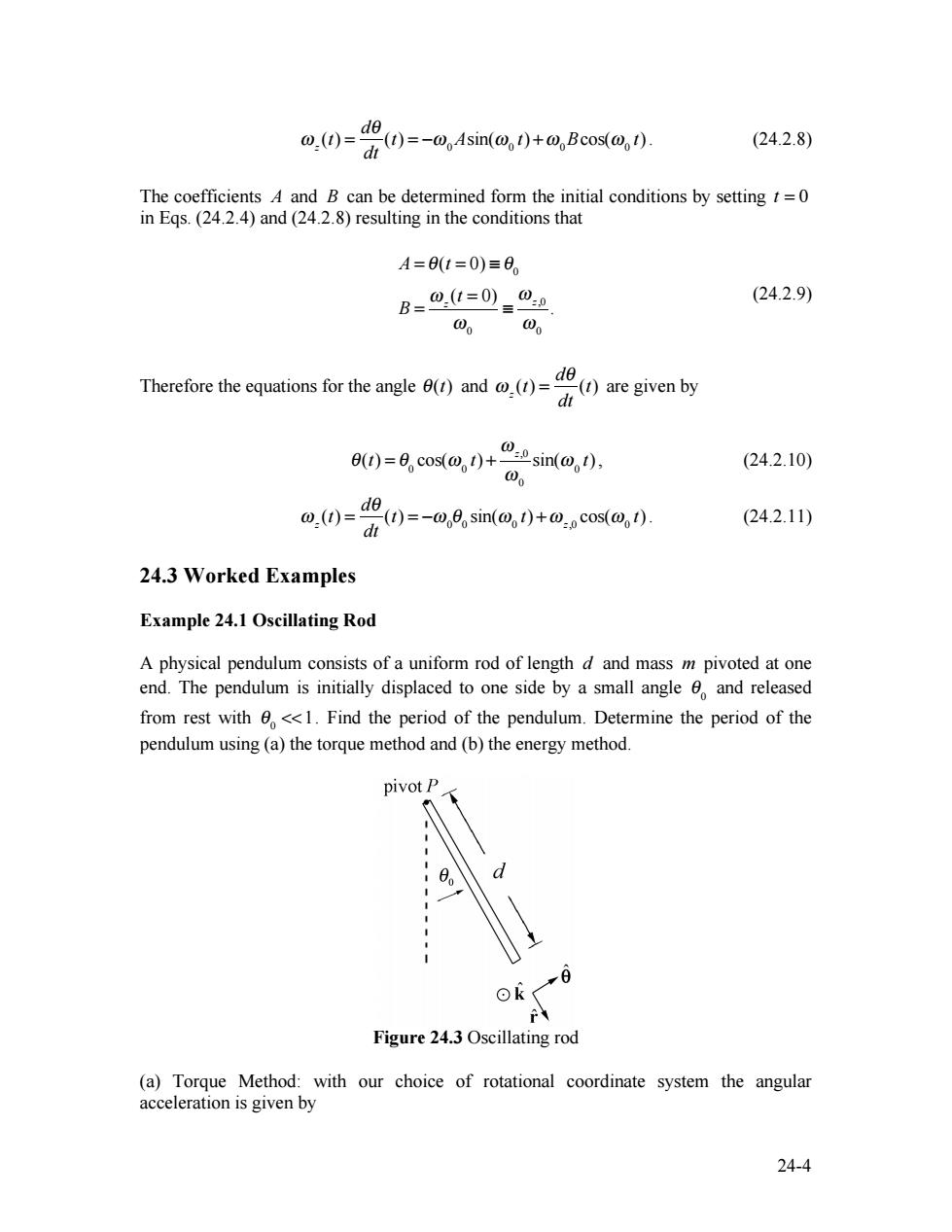
a.(0=B0=-0,4sina,)+,Bcox@,）. (24.2.8) dt The coefficients 4 and B can be determined form the initial conditions by setting t=0 in Egs.(24.2.4)and(24.2.8)resulting in the conditions that A=(1=0)≡0 B=0:1=0=02 (24.2.9) 一三一 00 00 Therefore the nfortheane(r)d))reve by O)=0,cos!)+sin,). (24.2.10) 0.0= ed)=-0,8sin(@,0+0ncos(0,) (24.2.11) 24.3 Worked Examples Example 24.1 Oscillating Rod A physical pendulum consists of a uniform rod of length d and mass m pivoted at one end.The pendulum is initially displaced to one side by a small angle e and released from rest with <<1.Find the period of the pendulum.Determine the period of the pendulum using (a)the torque method and(b)the energy method. pivot P ⊙k *8 Figure 24.3 Oscillating rod (a)Torque Method:with our choice of rotational coordinate system the angular acceleration is given by 24-424-4 ω z (t) = dθ dt (t) = −ω0Asin(ω0 t) +ω0Bcos(ω0 t). (24.2.8) The coefficients A and B can be determined form the initial conditions by setting t = 0 in Eqs. (24.2.4) and (24.2.8) resulting in the conditions that A = θ(t = 0) ≡ θ0 B = ω z (t = 0) ω0 ≡ ω z,0 ω0 . (24.2.9) Therefore the equations for the angle θ(t) and ω z (t) = dθ dt (t) are given by θ(t) = θ0 cos(ω0 t) + ω z,0 ω0 sin(ω0 t), (24.2.10) ω z (t) = dθ dt (t) = −ω0 θ0 sin(ω0 t) +ω z,0 cos(ω0 t). (24.2.11) 24.3 Worked Examples Example 24.1 Oscillating Rod A physical pendulum consists of a uniform rod of length d and mass m pivoted at one end. The pendulum is initially displaced to one side by a small angle θ0 and released from rest with θ0 <<1. Find the period of the pendulum. Determine the period of the pendulum using (a) the torque method and (b) the energy method. Figure 24.3 Oscillating rod (a) Torque Method: with our choice of rotational coordinate system the angular acceleration is given by