正在加载图片...
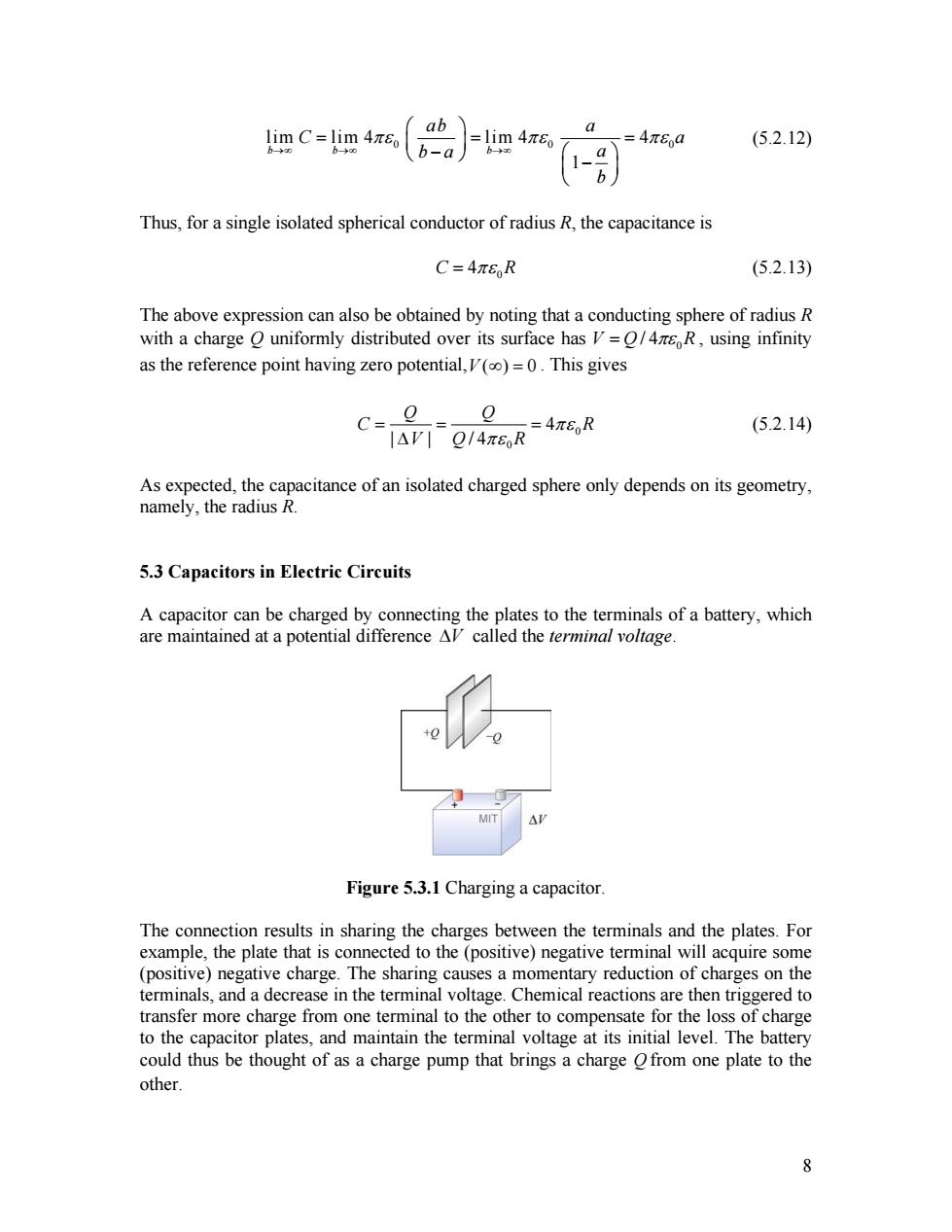
ab limC=lim4πeo =1im4π8o 0 =4π8oa (5.2.12) b-oc b-a b Thus,for a single isolated spherical conductor of radius R,the capacitance is C=4π6R (5.2.13) The above expression can also be obtained by noting that a conducting sphere of radius R with a charge O uniformly distributed over its surface has V=O/4mR,using infinity as the reference point having zero potential,V(o)=0.This gives C= 0 =4π8R (5.2.14) |△V|Q/4πER As expected,the capacitance of an isolated charged sphere only depends on its geometry, namely,the radius R. 5.3 Capacitors in Electric Circuits A capacitor can be charged by connecting the plates to the terminals of a battery,which are maintained at a potential difference AV called the terminal voltage. MIT △V Figure 5.3.1 Charging a capacitor. The connection results in sharing the charges between the terminals and the plates.For example,the plate that is connected to the (positive)negative terminal will acquire some (positive)negative charge.The sharing causes a momentary reduction of charges on the terminals,and a decrease in the terminal voltage.Chemical reactions are then triggered to transfer more charge from one terminal to the other to compensate for the loss of charge to the capacitor plates,and maintain the terminal voltage at its initial level.The battery could thus be thought of as a charge pump that brings a charge Ofrom one plate to the other. 80 0 lim lim 4 lim 4 4 1 b b b ab a C a b a a b π 0 ε πε →∞ →∞ →∞ ⎛ ⎞ = ⎜ ⎟ = ⎝ ⎠ − ⎛ ⎞ ⎜ ⎟ − ⎝ ⎠ = πε R (5.2.12) Thus, for a single isolated spherical conductor of radius R, the capacitance is 0 C = 4πε (5.2.13) The above expression can also be obtained by noting that a conducting sphere of radius R with a charge Q uniformly distributed over its surface has 0 V Q= / 4πε R , using infinity as the reference point having zero potential,V ( ) ∞ = 0 . This gives 0 0 4 | | / 4 Q Q C V Q R πε R πε = = = ∆ (5.2.14) As expected, the capacitance of an isolated charged sphere only depends on its geometry, namely, the radius R. 5.3 Capacitors in Electric Circuits A capacitor can be charged by connecting the plates to the terminals of a battery, which are maintained at a potential difference ∆V called the terminal voltage. Figure 5.3.1 Charging a capacitor. The connection results in sharing the charges between the terminals and the plates. For example, the plate that is connected to the (positive) negative terminal will acquire some (positive) negative charge. The sharing causes a momentary reduction of charges on the terminals, and a decrease in the terminal voltage. Chemical reactions are then triggered to transfer more charge from one terminal to the other to compensate for the loss of charge to the capacitor plates, and maintain the terminal voltage at its initial level. The battery could thus be thought of as a charge pump that brings a charge Q from one plate to the other. 8