
Chapter 5 Capacitance and Dielectrics 5.1 Introduction..… 2 5.2 Calculation of Capacitance................... 3 Example 5.1:Parallel-Plate Capacitor.... 3 Interactive Simulation 5.1:Parallel-Plate Capacitor Example 5.2:Cylindrical Capacitor 5 Example 5.3:Spherical Capacitor.... .7 5.3 Capacitors in Electric Circuits......... .8 5.3.1 Parallel Connection............... .9 5.3.2 Series Connection.... 10 Example 5.4:Equivalent Capacitance.... 11 5.4 Storing Energy in a Capacitor............... 12 5.4.1 Energy Density of the Electric Field....... 13 Interactive Simulation 5.2:Charge Placed between Capacitor Plates 13 Example 5.5:Electric Energy Density of Dry Air..................................... 14 Example 5.6:Energy Stored in a Spherical Shell...... 14 5.5 Dielectrics. 15 5.5.1 Polarization............ 17 5.5.2 Dielectrics without Battery.............. 20 5.5.3 Dielectrics with Battery........... 21 5.5.4 Gauss's Law for Dielectrics.............. 22 Example 5.7:Capacitance with Dielectrics 24 5.6 Creating Electric Fields 25 Animation 5.1:Creating an Electric Dipole..... 25 Animation 5.2:Creating and Destroying Electric Energy 27 5.7 Summary.… 28 5.8 Appendix:Electric Fields Hold Atoms Together............... 29 5.8.1 Ionic and van der Waals Forces.................... 30 Interactive Simulation 5.3:Collection of Charges in Two Dimensions ..31 Interactive Simulation 5.4:Collection of Charges in Three Dimensions 33 Interactive Simulation 5.5: Collection of Dipoles in Two Dimensions.............33 Interactive Simulation 5.6: Charged Particle Trap. 4 Interactive Simulation 5.6:Lattice 3D ........................... 35 Interactive Simulation 5.7:2D Electrostatic Suspension Bridge 35 Interactive Simulation 5.8:3D Electrostatic Suspension Bridge 36 5.9 Problem-Solving Strategy:Calculating Capacitance. 36 0
Chapter 5 Capacitance and Dielectrics 5.1 Introduction.............................................................................................................. 2 5.2 Calculation of Capacitance ...................................................................................... 3 Example 5.1: Parallel-Plate Capacitor....................................................................... 3 Interactive Simulation 5.1: Parallel-Plate Capacitor .............................................. 5 Example 5.2: Cylindrical Capacitor........................................................................... 5 Example 5.3: Spherical Capacitor.............................................................................. 7 5.3 Capacitors in Electric Circuits................................................................................. 8 5.3.1 Parallel Connection........................................................................................... 9 5.3.2 Series Connection ........................................................................................... 10 Example 5.4: Equivalent Capacitance ..................................................................... 11 5.4 Storing Energy in a Capacitor................................................................................ 12 5.4.1 Energy Density of the Electric Field............................................................... 13 Interactive Simulation 5.2: Charge Placed between Capacitor Plates................. 13 Example 5.5: Electric Energy Density of Dry Air................................................... 14 Example 5.6: Energy Stored in a Spherical Shell.................................................... 14 5.5 Dielectrics.............................................................................................................. 15 5.5.1 Polarization ..................................................................................................... 17 5.5.2 Dielectrics without Battery ............................................................................. 20 5.5.3 Dielectrics with Battery .................................................................................. 21 5.5.4 Gauss’s Law for Dielectrics............................................................................ 22 Example 5.7: Capacitance with Dielectrics............................................................. 24 5.6 Creating Electric Fields ......................................................................................... 25 Animation 5.1: Creating an Electric Dipole ........................................................... 25 Animation 5.2: Creating and Destroying Electric Energy...................................... 27 5.7 Summary................................................................................................................ 28 5.8 Appendix: Electric Fields Hold Atoms Together .................................................. 29 5.8.1 Ionic and van der Waals Forces...................................................................... 30 Interactive Simulation 5.3: Collection of Charges in Two Dimensions............... 31 Interactive Simulation 5.4: Collection of Charges in Three Dimensions............. 33 Interactive Simulation 5.5: Collection of Dipoles in Two Dimensions ............... 33 Interactive Simulation 5.6: Charged Particle Trap ............................................... 34 Interactive Simulation 5.6: Lattice 3D ................................................................. 35 Interactive Simulation 5.7: 2D Electrostatic Suspension Bridge ......................... 35 Interactive Simulation 5.8: 3D Electrostatic Suspension Bridge ......................... 36 5.9 Problem-Solving Strategy: Calculating Capacitance............................................. 36 0

5.10 Solved Problems. 38 5.10.1 Equivalent Capacitance. 38 5.10.2 Capacitor Filled with Two Different Dielectrics..................................... 39 5.10.3 Capacitor with Dielectrics..39 5.10.4 Capacitor Connected to a Spring. 41 5.11 Conceptual Questions........... 41 5.12 Additional Problems........ 42 5.12.1 Capacitors in Series and in Parallel 42 5.12.2 Capacitors and Dielectrics............ 42 5.12.3 Gauss's Law in the Presence of a Dielectric. 43 5.12.4 Gauss's Law and Dielectrics........ 43 .12.5 A Capacitor with a Dielectric................... 44 5.12.6 Force on the Plates of a Capacitor................ 44 5.12.7 Energy Density in a Capacitor with a Dielectric........ 45 1
5.10 Solved Problems.................................................................................................. 38 5.10.1 Equivalent Capacitance................................................................................. 38 5.10.2 Capacitor Filled with Two Different Dielectrics........................................... 39 5.10.3 Capacitor with Dielectrics............................................................................. 39 5.10.4 Capacitor Connected to a Spring................................................................... 41 5.11 Conceptual Questions.......................................................................................... 41 5.12 Additional Problems............................................................................................ 42 5.12.1 Capacitors in Series and in Parallel .............................................................. 42 5.12.2 Capacitors and Dielectrics ............................................................................ 42 5.12.3 Gauss’s Law in the Presence of a Dielectric................................................. 43 5.12.4 Gauss’s Law and Dielectrics......................................................................... 43 5.12.5 A Capacitor with a Dielectric ....................................................................... 44 5.12.6 Force on the Plates of a Capacitor ................................................................ 44 5.12.7 Energy Density in a Capacitor with a Dielectric .......................................... 45 1
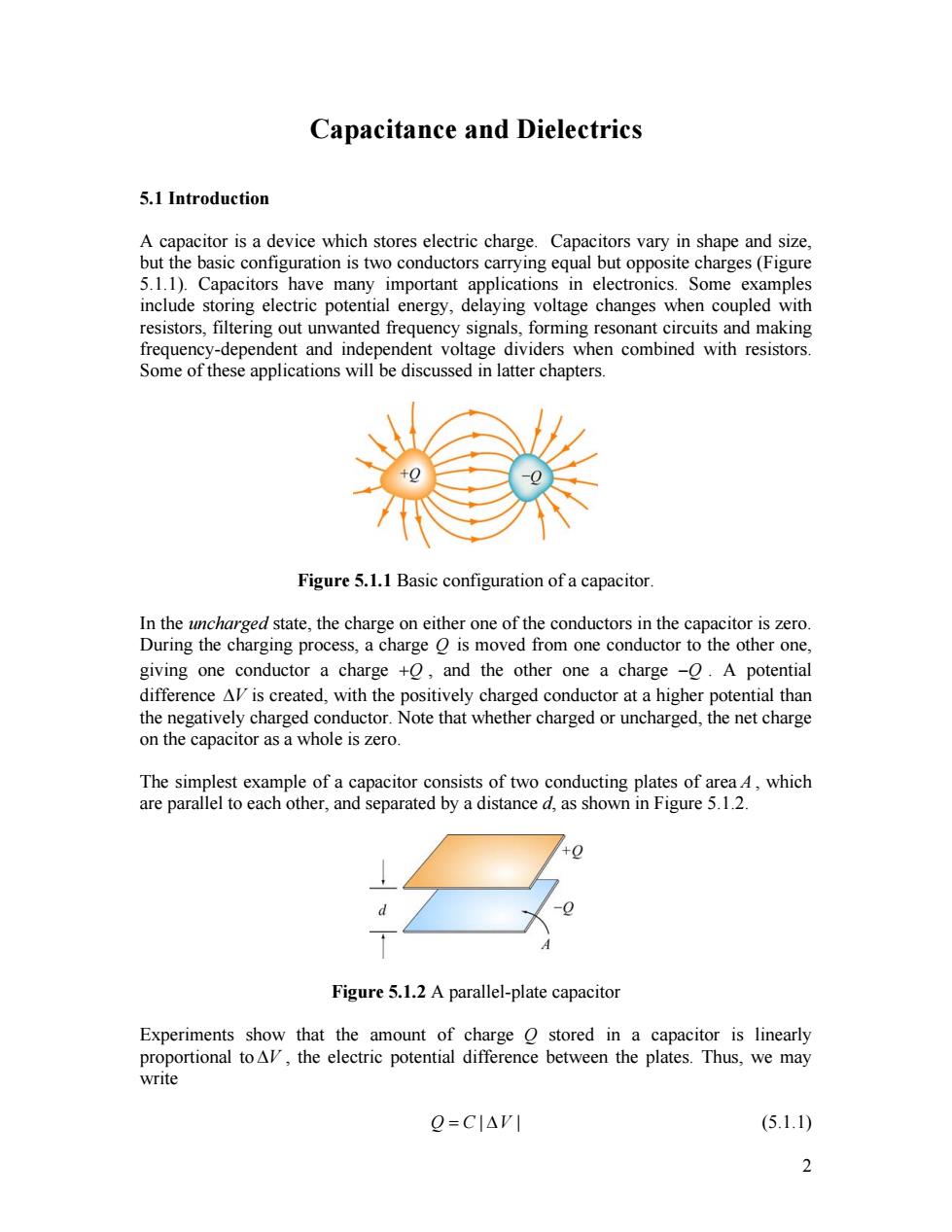
Capacitance and Dielectrics 5.1 Introduction A capacitor is a device which stores electric charge.Capacitors vary in shape and size, but the basic configuration is two conductors carrying equal but opposite charges(Figure 5.1.1).Capacitors have many important applications in electronics.Some examples include storing electric potential energy,delaying voltage changes when coupled with resistors,filtering out unwanted frequency signals,forming resonant circuits and making frequency-dependent and independent voltage dividers when combined with resistors. Some of these applications will be discussed in latter chapters. Figure 5.1.1 Basic configuration of a capacitor. In the uncharged state,the charge on either one of the conductors in the capacitor is zero. During the charging process,a charge o is moved from one conductor to the other one, giving one conductor a charge +0,and the other one a charge -O.A potential difference Al is created,with the positively charged conductor at a higher potential than the negatively charged conductor.Note that whether charged or uncharged,the net charge on the capacitor as a whole is zero. The simplest example of a capacitor consists of two conducting plates of area 4,which are parallel to each other,and separated by a distance d,as shown in Figure 5.1.2. Figure 5.1.2 A parallel-plate capacitor Experiments show that the amount of charge o stored in a capacitor is linearly proportional toAl,the electric potential difference between the plates.Thus,we may write Q=CI△VI (5.1.1) 2
Capacitance and Dielectrics 5.1 Introduction A capacitor is a device which stores electric charge. Capacitors vary in shape and size, but the basic configuration is two conductors carrying equal but opposite charges (Figure 5.1.1). Capacitors have many important applications in electronics. Some examples include storing electric potential energy, delaying voltage changes when coupled with resistors, filtering out unwanted frequency signals, forming resonant circuits and making frequency-dependent and independent voltage dividers when combined with resistors. Some of these applications will be discussed in latter chapters. Figure 5.1.1 Basic configuration of a capacitor. In the uncharged state, the charge on either one of the conductors in the capacitor is zero. During the charging process, a charge Q is moved from one conductor to the other one, giving one conductor a charge +Q , and the other one a charge . A potential difference is created, with the positively charged conductor at a higher potential than the negatively charged conductor. Note that whether charged or uncharged, the net charge on the capacitor as a whole is zero. −Q ∆V The simplest example of a capacitor consists of two conducting plates of area A , which are parallel to each other, and separated by a distance d, as shown in Figure 5.1.2. Figure 5.1.2 A parallel-plate capacitor Experiments show that the amount of charge Q stored in a capacitor is linearly proportional to , the electric potential difference between the plates. Thus, we may write ∆V Q C= | ∆V | (5.1.1) 2

where C is a positive proportionality constant called capacitance.Physically capacitance is a measure of the capacity of storing electric charge for a given potential difference AV.The SI unit of capacitance is the farad (F): 1 F=1 farad=1 coulomb/volt=1 C/V A typical capacitance is in the picofarad (1 pF=10-2F)to millifarad range, (1mF=10-3F=1000F;1F=10-6F) Figure 5.1.3(a)shows the symbol which is used to represent capacitors in circuits.For a polarized fixed capacitor which has a definite polarity,Figure 5.1.3(b)is sometimes used. (a) (b) Figure 5.1.3 Capacitor symbols. 5.2 Calculation of Capacitance Let's see how capacitance can be computed in systems with simple geometry. Example 5.1:Parallel-Plate Capacitor Consider two metallic plates of equal area 4 separated by a distance d,as shown in Figure 5.2.1 below.The top plate carries a charge while the bottom plate carries a charge-O.The charging of the plates can be accomplished by means of a battery which produces a potential difference.Find the capacitance of the system. Figure 5.2.1 The electric field between the plates of a parallel-plate capacitor Solution: To find the capacitance C,we first need to know the electric field between the plates.A real capacitor is finite in size.Thus,the electric field lines at the edge of the plates are not straight lines,and the field is not contained entirely between the plates.This is known as 3
where C is a positive proportionality constant called capacitance. Physically, capacitance is a measure of the capacity of storing electric charge for a given potential difference ∆V . The SI unit of capacitance is the farad (F) : 1 F = = 1 farad 1 coulomb volt = 1 C V A typical capacitance is in the picofarad ( ) to millifarad range, ( ). 12 1 pF 10 F − = 3 6 1 mF 10 F=1000µ µ F; 1 F 10 F − − = = Figure 5.1.3(a) shows the symbol which is used to represent capacitors in circuits. For a polarized fixed capacitor which has a definite polarity, Figure 5.1.3(b) is sometimes used. (a) (b) Figure 5.1.3 Capacitor symbols. 5.2 Calculation of Capacitance Let’s see how capacitance can be computed in systems with simple geometry. Example 5.1: Parallel-Plate Capacitor Consider two metallic plates of equal area A separated by a distance d, as shown in Figure 5.2.1 below. The top plate carries a charge +Q while the bottom plate carries a charge –Q. The charging of the plates can be accomplished by means of a battery which produces a potential difference. Find the capacitance of the system. Figure 5.2.1 The electric field between the plates of a parallel-plate capacitor Solution: To find the capacitance C, we first need to know the electric field between the plates. A real capacitor is finite in size. Thus, the electric field lines at the edge of the plates are not straight lines, and the field is not contained entirely between the plates. This is known as 3

edge effects,and the non-uniform fields near the edge are called the fringing fields.In Figure 5.2.1 the field lines are drawn by taking into consideration edge effects.However, in what follows,we shall ignore such effects and assume an idealized situation,where field lines between the plates are straight lines. In the limit where the plates are infinitely large,the system has planar symmetry and we can calculate the electric field everywhere using Gauss's law given in Eq.(4.2.5): ∯EdA=9 80 By choosing a Gaussian "pillbox"with cap area 4'to enclose the charge on the positive plate(see Figure 5.2.2),the electric field in the region between the plates is EA'-4-A E=o (5.2.1) 6080 Eo The same result has also been obtained in Section 4.8.1 using superposition principle. Gaussian surface +0 A Path of intergration Figure 5.2.2 Gaussian surface for calculating the electric field between the plates. The potential difference between the plates is AV=V.-V.=-f.E.ds=-Ed (5.2.2) where we have taken the path of integration to be a straight line from the positive plate to the negative plate following the field lines(Figure 5.2.2).Since the electric field lines are always directed from higher potential to lower potential,V<V..However,in computing the capacitance C,the relevant quantity is the magnitude of the potential difference: |△V=Ed (5.2.3) and its sign is immaterial.From the definition of capacitance,we have 4
edge effects, and the non-uniform fields near the edge are called the fringing fields. In Figure 5.2.1 the field lines are drawn by taking into consideration edge effects. However, in what follows, we shall ignore such effects and assume an idealized situation, where field lines between the plates are straight lines. In the limit where the plates are infinitely large, the system has planar symmetry and we can calculate the electric field everywhere using Gauss’s law given in Eq. (4.2.5): enc S 0 q d ε ⋅ = ∫∫ E A JG JG w By choosing a Gaussian “pillbox” with cap area A′ to enclose the charge on the positive plate (see Figure 5.2.2), the electric field in the region between the plates is enc 0 0 q A' EA' E 0 σ σ ε ε ε = = ⇒ = (5.2.1) The same result has also been obtained in Section 4.8.1 using superposition principle. Figure 5.2.2 Gaussian surface for calculating the electric field between the plates. The potential difference between the plates is V V V d Ed − − + + ∆ = − = − ⋅ = − ∫ E s G G (5.2.2) where we have taken the path of integration to be a straight line from the positive plate to the negative plate following the field lines (Figure 5.2.2). Since the electric field lines are always directed from higher potential to lower potential, V− < V+ . However, in computing the capacitance C, the relevant quantity is the magnitude of the potential difference: | V∆ | E = d (5.2.3) and its sign is immaterial. From the definition of capacitance, we have 4

Q 60A (parallel plate) (5.2.4) |△d Note that C depends only on the geometric factors 4 and d.The capacitance C increases linearly with the area A since for a given potential difference Al,a bigger plate can hold more charge.On the other hand,C is inversely proportional to d,the distance of separation because the smaller the value of d,the smaller the potential difference AV for a fixed O. Interactive Simulation 5.1:Parallel-Plate Capacitor This simulation shown in Figure 5.2.3 illustrates the interaction of charged particles inside the two plates of a capacitor. 。9,生4。 Figure 5.2.3 Charged particles interacting inside the two plates of a capacitor. Each plate contains twelve charges interacting via Coulomb force,where one plate contains positive charges and the other contains negative charges.Because of their mutual repulsion,the particles in each plate are compelled to maximize the distance between one another,and thus spread themselves evenly around the outer edge of their enclosure.However,the particles in one plate are attracted to the particles in the other,so they attempt to minimize the distance between themselves and their oppositely charged correspondents.Thus,they distribute themselves along the surface of their bounding box closest to the other plate. Example 5.2:Cylindrical Capacitor Consider next a solid cylindrical conductor of radius a surrounded by a coaxial cylindrical shell of inner radius b,as shown in Figure 5.2.4.The length of both cylinders is L and we take this length to be much larger than b-a,the separation of the cylinders, so that edge effects can be neglected.The capacitor is charged so that the inner cylinder has charge +O while the outer shell has a charge-O.What is the capacitance? 5
0 parallel plate Q A C | V | d ε = = ∆ ( ) (5.2.4) Note that C depends only on the geometric factors A and d. The capacitance C increases linearly with the area A since for a given potential difference ∆V , a bigger plate can hold more charge. On the other hand, C is inversely proportional to d, the distance of separation because the smaller the value of d, the smaller the potential difference | | ∆V for a fixed Q. Interactive Simulation 5.1: Parallel-Plate Capacitor This simulation shown in Figure 5.2.3 illustrates the interaction of charged particles inside the two plates of a capacitor. Figure 5.2.3 Charged particles interacting inside the two plates of a capacitor. Each plate contains twelve charges interacting via Coulomb force, where one plate contains positive charges and the other contains negative charges. Because of their mutual repulsion, the particles in each plate are compelled to maximize the distance between one another, and thus spread themselves evenly around the outer edge of their enclosure. However, the particles in one plate are attracted to the particles in the other, so they attempt to minimize the distance between themselves and their oppositely charged correspondents. Thus, they distribute themselves along the surface of their bounding box closest to the other plate. Example 5.2: Cylindrical Capacitor Consider next a solid cylindrical conductor of radius a surrounded by a coaxial cylindrical shell of inner radius b, as shown in Figure 5.2.4. The length of both cylinders is L and we take this length to be much larger than b− a, the separation of the cylinders, so that edge effects can be neglected. The capacitor is charged so that the inner cylinder has charge +Q while the outer shell has a charge –Q. What is the capacitance? 5

(a) (b) Figure 5.2.4 (a)A cylindrical capacitor.(b)End view of the capacitor.The electric field is non-vanishing only in the region a b,the enclosed charge is gne=-=0 since the Gaussian surface encloses equal but opposite charges from both conductors The potential difference is given by Ar=-=-ed=2 (5.2.6) π6oJar where we have chosen the integration path to be along the direction of the electric field lines.As expected,the outer conductor with negative charge has a lower potential.This gives Q L 2π6,L (5.2.7) |△V|ln(b/a)/2πEo In(b/a) Once again,we see that the capacitance C depends only on the geometrical factors,L,a and b. 6
(a) (b) Figure 5.2.4 (a) A cylindrical capacitor. (b) End view of the capacitor. The electric field is non-vanishing only in the region a b enc q = λA A − λ = 0 since the Gaussian surface encloses equal but opposite charges from both conductors. The potential difference is given by 0 0 ln 2 2 b b a r a b a dr b V V V E dr r a λ λ πε πε ⎛ ⎞ ∆ = − = − = − = − ⎜ ⎟ ⎝ ⎠ ∫ ∫ (5.2.6) where we have chosen the integration path to be along the direction of the electric field lines. As expected, the outer conductor with negative charge has a lower potential. This gives 0 0 2 | | ln( / )/ 2 ln( / ) Q L L C V b a b λ πε λ πε = = = ∆ a (5.2.7) Once again, we see that the capacitance C depends only on the geometrical factors, L, a and b. 6
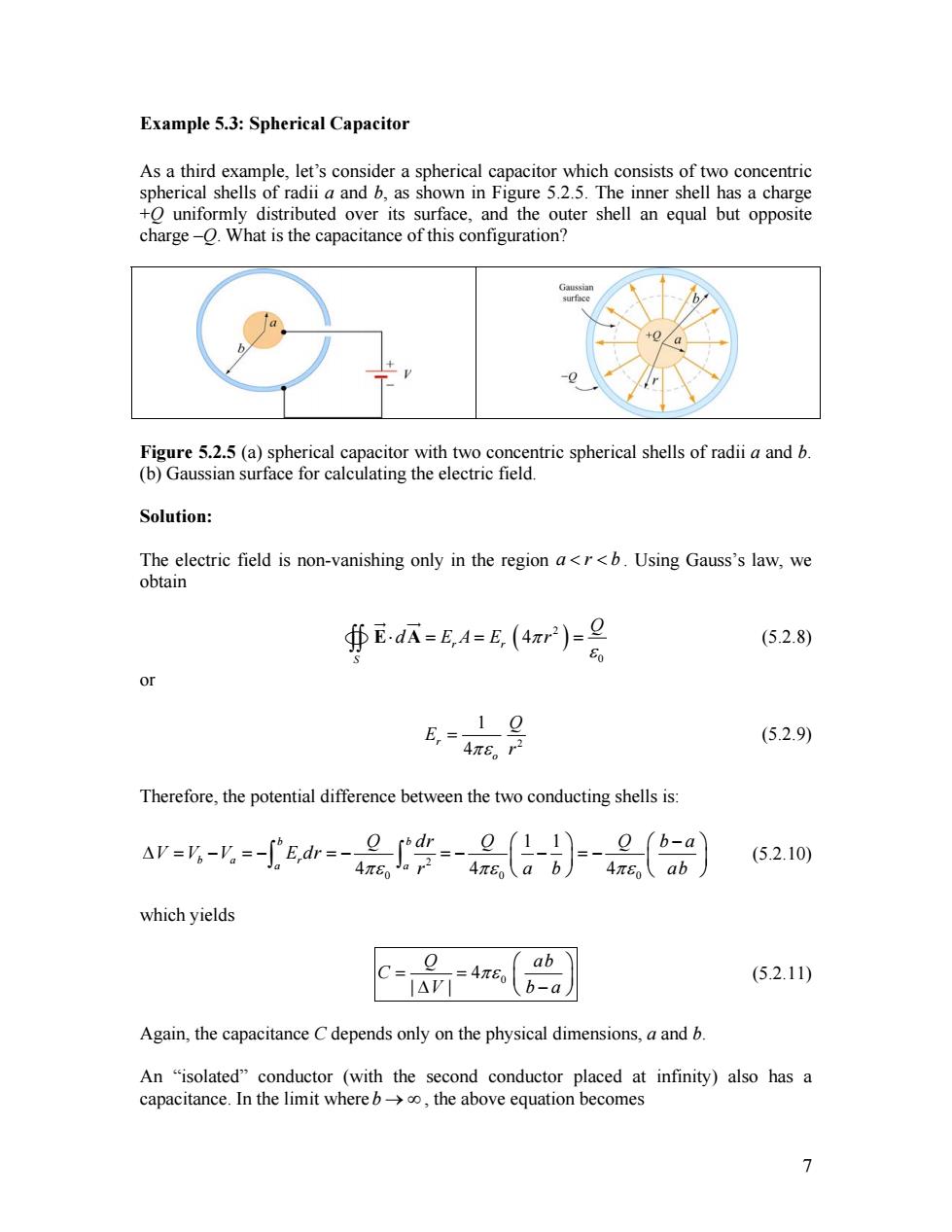
Example 5.3:Spherical Capacitor As a third example,let's consider a spherical capacitor which consists of two concentric spherical shells of radii a and b,as shown in Figure 5.2.5.The inner shell has a charge +O uniformly distributed over its surface,and the outer shell an equal but opposite charge-O.What is the capacitance of this configuration? Gaussian surface Figure 5.2.5 (a)spherical capacitor with two concentric spherical shells of radii a and b. (b)Gaussian surface for calculating the electric field. Solution: The electric field is non-vanishing only in the region aoo,the above equation becomes 7
Example 5.3: Spherical Capacitor As a third example, let’s consider a spherical capacitor which consists of two concentric spherical shells of radii a and b, as shown in Figure 5.2.5. The inner shell has a charge +Q uniformly distributed over its surface, and the outer shell an equal but opposite charge –Q. What is the capacitance of this configuration? Figure 5.2.5 (a) spherical capacitor with two concentric spherical shells of radii a and b. (b) Gaussian surface for calculating the electric field. Solution: The electric field is non-vanishing only in the region a r < < b . Using Gauss’s law, we obtain ( ) 2 0 4 r r S Q d E A E π r ε ⋅ = = = ∫∫ E A JG JG w (5.2.8) or 2 1 4 r o Q E πε r = (5.2.9) Therefore, the potential difference between the two conducting shells is: 2 0 0 0 1 1 4 4 4 b b b a r a a Q dr Q Q b a V V V E dr πε r a πε b πε ⎛ ⎞ ⎛ − ∆ = − = − = − = − ⎜ ⎟ − = − ⎜ ⎝ ⎠ ⎝ ∫ ∫ ab ⎞ ⎟ ⎠ (5.2.10) which yields 0 4 | | Q C V b πε ⎛ = = ⎜ ∆ ⎝ ⎠ ab a ⎞ ⎟ − (5.2.11) Again, the capacitance C depends only on the physical dimensions, a and b. An “isolated” conductor (with the second conductor placed at infinity) also has a capacitance. In the limit whereb → ∞ , the above equation becomes 7
ab limC=lim4πeo =1im4π8o 0 =4π8oa (5.2.12) b-oc b-a b Thus,for a single isolated spherical conductor of radius R,the capacitance is C=4π6R (5.2.13) The above expression can also be obtained by noting that a conducting sphere of radius R with a charge O uniformly distributed over its surface has V=O/4mR,using infinity as the reference point having zero potential,V(o)=0.This gives C= 0 =4π8R (5.2.14) |△V|Q/4πER As expected,the capacitance of an isolated charged sphere only depends on its geometry, namely,the radius R. 5.3 Capacitors in Electric Circuits A capacitor can be charged by connecting the plates to the terminals of a battery,which are maintained at a potential difference AV called the terminal voltage. MIT △V Figure 5.3.1 Charging a capacitor. The connection results in sharing the charges between the terminals and the plates.For example,the plate that is connected to the (positive)negative terminal will acquire some (positive)negative charge.The sharing causes a momentary reduction of charges on the terminals,and a decrease in the terminal voltage.Chemical reactions are then triggered to transfer more charge from one terminal to the other to compensate for the loss of charge to the capacitor plates,and maintain the terminal voltage at its initial level.The battery could thus be thought of as a charge pump that brings a charge Ofrom one plate to the other. 8
0 0 lim lim 4 lim 4 4 1 b b b ab a C a b a a b π 0 ε πε →∞ →∞ →∞ ⎛ ⎞ = ⎜ ⎟ = ⎝ ⎠ − ⎛ ⎞ ⎜ ⎟ − ⎝ ⎠ = πε R (5.2.12) Thus, for a single isolated spherical conductor of radius R, the capacitance is 0 C = 4πε (5.2.13) The above expression can also be obtained by noting that a conducting sphere of radius R with a charge Q uniformly distributed over its surface has 0 V Q= / 4πε R , using infinity as the reference point having zero potential,V ( ) ∞ = 0 . This gives 0 0 4 | | / 4 Q Q C V Q R πε R πε = = = ∆ (5.2.14) As expected, the capacitance of an isolated charged sphere only depends on its geometry, namely, the radius R. 5.3 Capacitors in Electric Circuits A capacitor can be charged by connecting the plates to the terminals of a battery, which are maintained at a potential difference ∆V called the terminal voltage. Figure 5.3.1 Charging a capacitor. The connection results in sharing the charges between the terminals and the plates. For example, the plate that is connected to the (positive) negative terminal will acquire some (positive) negative charge. The sharing causes a momentary reduction of charges on the terminals, and a decrease in the terminal voltage. Chemical reactions are then triggered to transfer more charge from one terminal to the other to compensate for the loss of charge to the capacitor plates, and maintain the terminal voltage at its initial level. The battery could thus be thought of as a charge pump that brings a charge Q from one plate to the other. 8

5.3.1 Parallel Connection Suppose we have two capacitors C with charge O and C2 with charge that are connected in parallel,as shown in Figure 5.3.2. IAKHAKH-AYI C Ceq-C1+C2 C 9 lrl ArI Figure 5.3.2 Capacitors in parallel and an equivalent capacitor. The left plates of both capacitors Ci and C2 are connected to the positive terminal of the battery and have the same electric potential as the positive terminal.Similarly,both right plates are negatively charged and have the same potential as the negative terminal.Thus, the potential difference Al is the same across each capacitor.This gives 品 6& (5.3.1) These two capacitors can be replaced by a single equivalent capacitor Cwith a total charge Osupplied by the battery.However,since O is shared by the two capacitors,we must have Q=g+Q2=CI△VI+C21△V=(C+C2)IAVI (5.3.2) The equivalent capacitance is then seen to be given by Coa=AV =C+C2 (5.3.3) Thus,capacitors that are connected in parallel add.The generalization to any number of capacitors is Cm=C+C2+C3++Cw=∑C (parallel) (5.3.4) i=l 9
5.3.1 Parallel Connection Suppose we have two capacitors C1 with charge Q1 and C with charge 2 Q2 that are connected in parallel, as shown in Figure 5.3.2. Figure 5.3.2 Capacitors in parallel and an equivalent capacitor. The left plates of both capacitors C1 and C2 are connected to the positive terminal of the battery and have the same electric potential as the positive terminal. Similarly, both right plates are negatively charged and have the same potential as the negative terminal. Thus, the potential difference | ∆V | is the same across each capacitor. This gives 1 2 1 2 , | | | Q Q C C V = = ∆ ∆V | (5.3.1) These two capacitors can be replaced by a single equivalent capacitor with a total charge Q Ceq supplied by the battery. However, since Q is shared by the two capacitors, we must have Q Q= +1 2 Q = C1 | | ∆V +C2 | | ∆V = ( C1 +C2 )| ∆V | (5.3.2) The equivalent capacitance is then seen to be given by eq 1 2 | | Q C C V = = +C ∆ (5.3.3) Thus, capacitors that are connected in parallel add. The generalization to any number of capacitors is eq 1 2 3 1 (parallel) N N i i C C C C C C = = + + +"+ = ∑ (5.3.4) 9