
MASSACHUSETTS INSTITUTE OF TECHNOLOGY Department of Physics 8.02 Spring 2013 Exam 1 Practice Problems Solutions Part I:Short Questions and Concept Questions Problem 1:Spark Plug Pictured at right is a typical spark plug(for scale,the thread diameter is about 10 mm). 10 mm About what voltage does your car ignition system need to generate to make a spark,if the breakdown field in a gas/air mixture is about 10 times higher than in air?For those of you unfamiliar with spark plugs,the spark is generated in the gap at the left of the top picture(top of the bottom picture).The white on the right is a ceramic which acts as an insulator between the high voltage center and the grounded outer(threaded)part. Answer. From the picture,if the threads have a diameter of 10 mm,then the gap is about 0.5 mm.The breakdown is 10 times higher than in air,so its 3 x 10'V/m.So to get breakdown you need a potential difference across the gap of △V=Ed=(3×10V-m-)5×104m)=1.5×104V 1
1 MASSACHUSETTS INSTITUTE OF TECHNOLOGY Department of Physics 8.02 Spring 2013 Exam 1 Practice Problems Solutions Part I: Short Questions and Concept Questions Problem 1: Spark Plug Pictured at right is a typical spark plug (for scale, the thread diameter is about 10 mm). About what voltage does your car ignition system need to generate to make a spark, if the breakdown field in a gas/air mixture is about 10 times higher than in air? For those of you unfamiliar with spark plugs, the spark is generated in the gap at the left of the top picture (top of the bottom picture). The white on the right is a ceramic which acts as an insulator between the high voltage center and the grounded outer (threaded) part. Answer. From the picture, if the threads have a diameter of 10 mm, then the gap is about 0.5 mm. The breakdown is 10 times higher than in air, so its 3 x 107 V/m. So to get breakdown you need a potential difference across the gap of !V ! Ed = (3"107 V#m$1 )(5"10$4 m) = 1.5"104 V
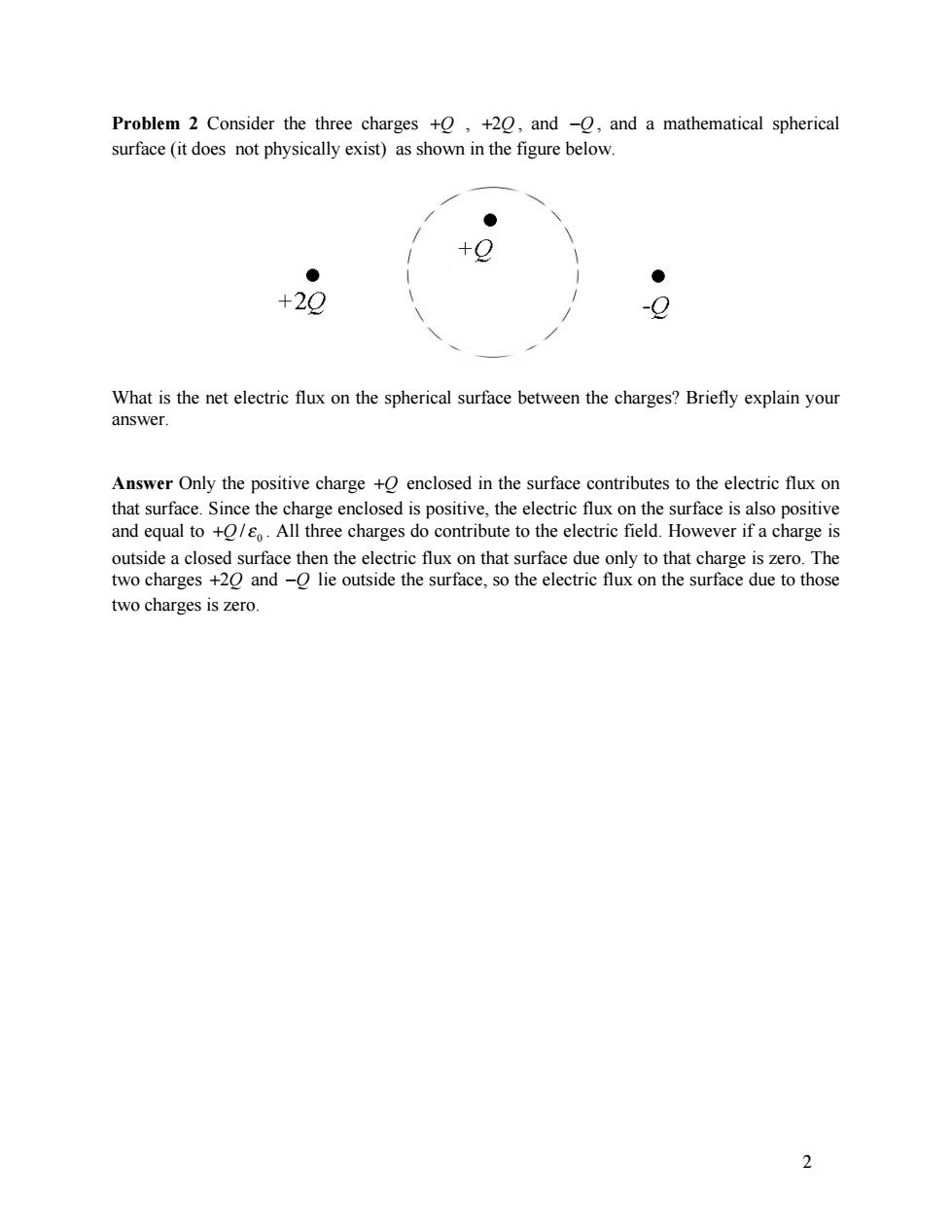
Problem 2 Consider the three charges +o,+20,and -O,and a mathematical spherical surface(it does not physically exist)as shown in the figure below. ● ● +2Q Q What is the net electric flux on the spherical surface between the charges?Briefly explain your answer. Answer Only the positive charge enclosed in the surface contributes to the electric flux on that surface.Since the charge enclosed is positive,the electric flux on the surface is also positive and equal to +/.All three charges do contribute to the electric field.However if a charge is outside a closed surface then the electric flux on that surface due only to that charge is zero.The two charges +20 and-O lie outside the surface,so the electric flux on the surface due to those two charges is zero. 2
2 Problem 2 Consider the three charges +Q , +2Q , and !Q , and a mathematical spherical surface (it does not physically exist) as shown in the figure below. What is the net electric flux on the spherical surface between the charges? Briefly explain your answer. Answer Only the positive charge +Q enclosed in the surface contributes to the electric flux on that surface. Since the charge enclosed is positive, the electric flux on the surface is also positive and equal to 0 +Q / ! . All three charges do contribute to the electric field. However if a charge is outside a closed surface then the electric flux on that surface due only to that charge is zero. The two charges +2Q and !Q lie outside the surface, so the electric flux on the surface due to those two charges is zero

Problem 3:A pyramid has a square base of side a,and four faces which are equilateral triangles.A charge Q is placed on the center of the base of the pyramid.What is the net flux of electric field emerging from one of the triangular faces of the pyramid? 1. 0 2. Q 8Eo 3. Q 2e0 4. 旦 2Eo 5. Undetermined:we must know whether Q is infinitesimally above or below the plane? Answer 2:Explain your reasoning:Construct an eight faced closed surface consisting of two pyramids with the charge at the center.The total flux by Gauss's law is just/Since each face is identical,the flux through each face is one eight the total flux or /88 3
3 Problem 3: A pyramid has a square base of side a, and four faces which are equilateral triangles. A charge Q is placed on the center of the base of the pyramid. What is the net flux of electric field emerging from one of the triangular faces of the pyramid? 1. 0 2. Q 8! 0 3. Qa 2 2! 0 4. Q 2! 0 5. Undetermined: we must know whether Q is infinitesimally above or below the plane? Answer 2: Explain your reasoning: Construct an eight faced closed surface consisting of two pyramids with the charge at the center. The total flux by Gauss’s law is justQ / ! 0 . Since each face is identical, the flux through each face is one eight the total flux or Q / 8! 0

Problem 4:The work done by an external agent in moving a positively charged object that starts from rest at infinity and ends at rest at the point P midway between two charges of magnitude +0 and -20, starts at infinity ● 9>0 ends at midpoint p ● -29 1.is positive. 2.is negative 3.is zero 4.cannot be determined-not enough info is given. Answer 2 negative. The work done by the external agent in moving the positive charge g from infinity to the point P is equal to the negative of the work done by the electrostatic force.Each charged object is the same distance d from the point P.The work done by the electrostatic force is equal to the potential energy difference between infinity and the point P,which is given by A=qar=g号629}0 4
4 Problem 4: The work done by an external agent in moving a positively charged object that starts from rest at infinity and ends at rest at the point P midway between two charges of magnitude +Q and !2Q , 1. is positive. 2. is negative. 3. is zero. 4. cannot be determined – not enough info is given. Answer 2 negative. The work done by the external agent in moving the positive charge q from infinity to the point P is equal to the negative of the work done by the electrostatic force. Each charged object is the same distance d from the point P. The work done by the electrostatic force is equal to the potential energy difference between infinity and the point P, which is given by !U = q!V = q ke Q d " ke 2Q d # $ % & ' ( < 0
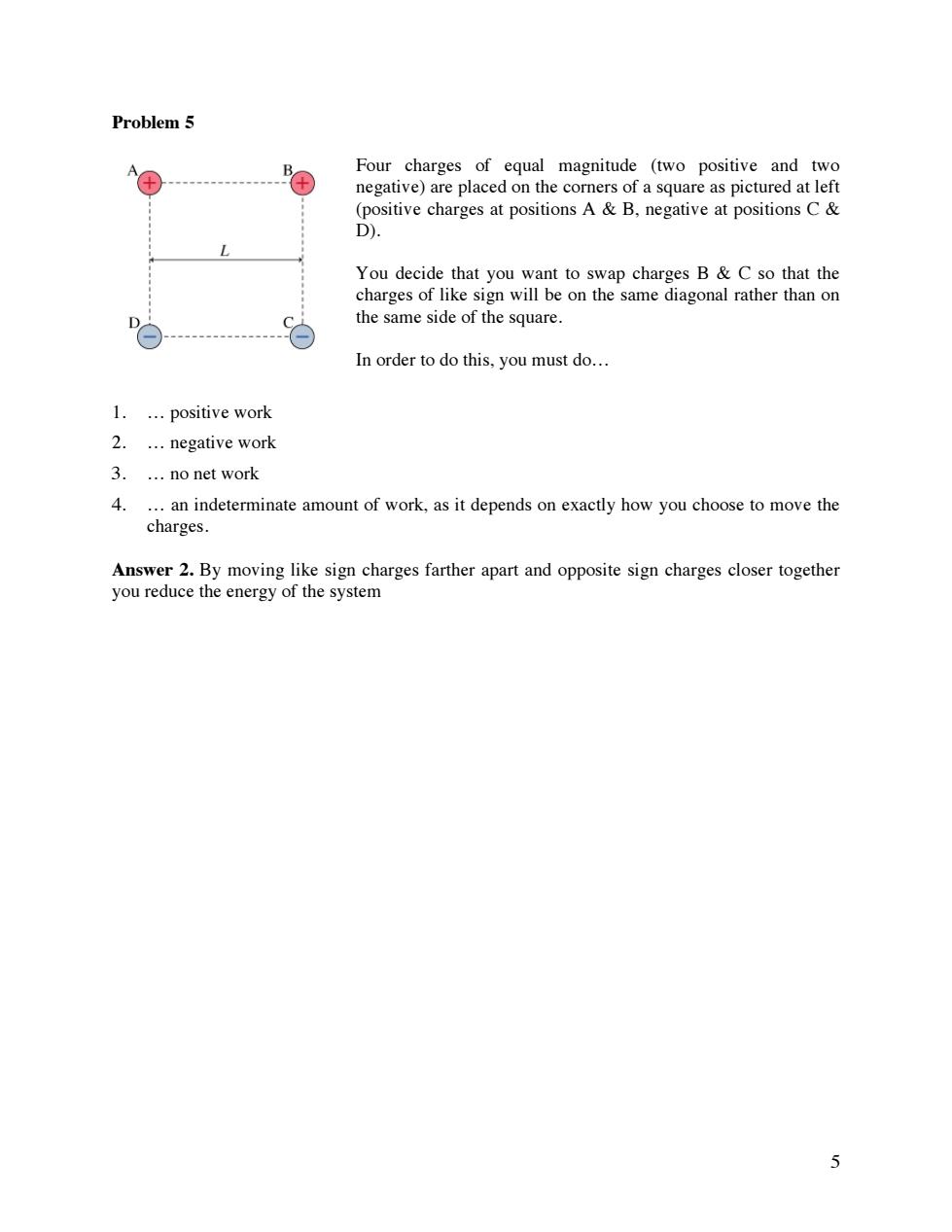
Problem 5 Four charges of equal magnitude (two positive and two negative)are placed on the corners of a square as pictured at left (positive charges at positions A &B,negative at positions C& D). You decide that you want to swap charges B C so that the charges of like sign will be on the same diagonal rather than on the same side of the square. In order to do this,you must do... 1. ..positive work 2. ..negative work 3. ..no net work 4. ..an indeterminate amount of work,as it depends on exactly how you choose to move the charges. Answer 2.By moving like sign charges farther apart and opposite sign charges closer together you reduce the energy of the system
5 Problem 5 Four charges of equal magnitude (two positive and two negative) are placed on the corners of a square as pictured at left (positive charges at positions A & B, negative at positions C & D). You decide that you want to swap charges B & C so that the charges of like sign will be on the same diagonal rather than on the same side of the square. In order to do this, you must do… 1. … positive work 2. … negative work 3. … no net work 4. … an indeterminate amount of work, as it depends on exactly how you choose to move the charges. Answer 2. By moving like sign charges farther apart and opposite sign charges closer together you reduce the energy of the system

Problem 6 Two opposite charges are placed on a line as shown in the figure below. 34 The charge on the right is three times the magnitude of the charge on the left.Besides infinite, where else can electric field possibly be zero? 1. between the two charges. 2.to the right of the charge on the right. 3.to the left of the charge on the left 4. the electric field is nowhere zero. Answer:3.The electric field from the positive charge E and the electric field from the negative charge E are shown for different regions with the arrow size indicative of the magnitude in the figure below. 30 飞9 +E。 3q E。← The only place the fields can cancel is to the left of the negative charge.For the region to the right of the charge of greater magnitude,the smaller charge is further away and so the magnitude of the field of the smaller charge can never equal the magnitude of the field of the larger charge. 6
6 Problem 6 Two opposite charges are placed on a line as shown in the figure below. The charge on the right is three times the magnitude of the charge on the left. Besides infinite, where else can electric field possibly be zero? 1. between the two charges. 2. to the right of the charge on the right. 3. to the left of the charge on the left. 4. the electric field is nowhere zero. Answer: 3. The electric field from the positive charge ! E+3q and the electric field from the negative charge ! E!q are shown for different regions with the arrow size indicative of the magnitude in the figure below. The only place the fields can cancel is to the left of the negative charge. For the region to the right of the charge of greater magnitude, the smaller charge is further away and so the magnitude of the field of the smaller charge can never equal the magnitude of the field of the larger charge

Part II Analytic Problems Problem 1 Non-uniformly charged sphere A sphere of radius R has a charge density p=p(r/R)where po is a constant and r is the distance from the center of the sphere. a)What is the total charge inside the sphere? Solution: The total charge inside the sphere is the integral Q=∫p4mrt=了p,r1r4r'dh=P4r r=R r=R Jrdh=P4πR4 R R4 =PoTR r'=0 r'=0 r=0 b)Find the electric field everywhere (both inside and outside the sphere). Solution: There are two regions of space:region I:rR so we apply Gauss'Law to each region to find the electric field. For region I:r<R,we choose a sphere of radius r as our Gaussian surface.Then,the F<E electric flux through this closed surface is ∯E,·aA=E,4πr2 Since the charge distribution is non-uniform,we will need to integrate the charge density to find the charge enclosed in our Gaussian surface.In the integral below we use the integration variable r'in order to distinguish it from the radius r of the Gaussian sphere. g=了p4ard=E了,1R4rw=了ra=- r'er Eo e0r'=0 4RE0 REo Notice that the integration is primed and the radius of the Gaussian sphere appears as a limit of the integral. 7
7 Part II Analytic Problems Problem 1 Non-uniformly charged sphere A sphere of radius R has a charge density ! = !0 (r / R) where !0 is a constant and r is the distance from the center of the sphere. a) What is the total charge inside the sphere? Solution: The total charge inside the sphere is the integral Q = !4"r 2 r#=0 r = R $ dr = !0 (r / R)4"r 2 r#=0 r = R $ dr = !0 4" R r 3 r =0 r = R $ dr = !0 4" R R4 4 = !0 " R3 b) Find the electric field everywhere (both inside and outside the sphere). Solution: There are two regions of space: region I:r R so we apply Gauss’ Law to each region to find the electric field. For region I:r < R , we choose a sphere of radius r as our Gaussian surface. Then, the electric flux through this closed surface is ! EI ! d ! """ A = EI ! 4#r 2 . Since the charge distribution is non-uniform, we will need to integrate the charge density to find the charge enclosed in our Gaussian surface. In the integral below we use the integration variable r! in order to distinguish it from the radius r of the Gaussian sphere. Qenc ! 0 = 1 ! 0 "4#r$ 2 r$=0 r$=r % dr$ = 1 ! 0 "0 (r$ / R)4#r$ 2 r$=0 r$=r % dr$ = "0 4# R! 0 r$ 3 r$=0 r$=r % dr$ = "0 4#r 4 4R! 0 = "0 #r 4 R! 0 . Notice that the integration is primed and the radius of the Gaussian sphere appears as a limit of the integral
Recall that Gauss's Law equates electric flux to charge enclosed: ∯E,dA= Eo So we substitute the two calculations above into Gauss's Law to arrive at: E,·4πr2=Pr REo We can solve this equation for the electric field Bi-F 05rR:we choose the same r>R spherical Gaussian surface of radiusr>R, and the electric flux has the same form ∯Ed瓜=En4πr2. All the charge is now enclosed,==PoR,so the right hand side of Gauss's Law becomes g-旦_PπR EoEo Eo Then Gauss's Law becomes En·4πr2=PπR We can solve this equation for the electric field 8
8 Recall that Gauss’s Law equates electric flux to charge enclosed: ! EI ! d ! """ A = Qenc # 0 . So we substitute the two calculations above into Gauss’s Law to arrive at: EI ! 4"r 2 = #0 "r 4 R$ 0 . We can solve this equation for the electric field ! EI = EI rˆ = !0 r 2 4R" 0 rˆ , 0 R : we choose the same spherical Gaussian surface of radiusr > R , and the electric flux has the same form ! EII ! d ! """ A = EII ! 4#r 2 . All the charge is now enclosed, Qenc = Q = !0 " R3 , so the right hand side of Gauss’s Law becomes Qenc ! 0 = Q ! 0 = "0 # R3 ! 0 . Then Gauss’s Law becomes EII ! 4"r 2 = #0 " R3 $ 0 . We can solve this equation for the electric field

En=Eyf=PoR f,r>R. 4Epr In this region of space,the electric field points radially outward and has magnitudeR e2,>R,so it falls off as 1/r as we expect since outside the charg distribution,the sphere acts as if it all the charge were concentrated at the origin. 9
9 ! EII = EII rˆ = !0R3 4" 0 r 2 rˆ , r > R . In this region of space, the electric field points radially outward and has magnitude ! EII = !0R3 4" 0 r 2 , r > R , so it falls off as 1 / r 2 as we expect since outside the charge distribution, the sphere acts as if it all the charge were concentrated at the origin
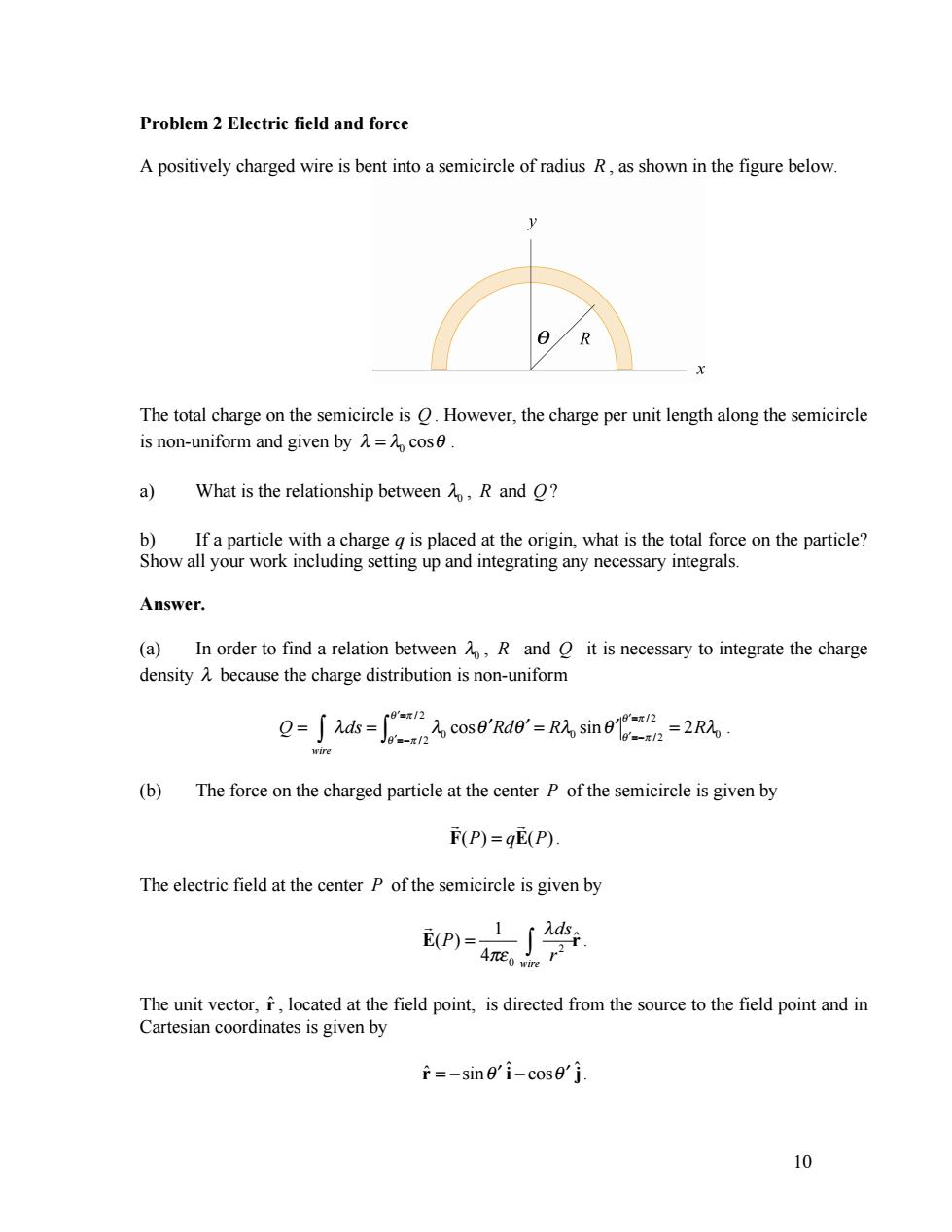
Problem 2 Electric field and force A positively charged wire is bent into a semicircle of radius R,as shown in the figure below. 0 R The total charge on the semicircle is O.However,the charge per unit length along the semicircle is non-uniform and given by入=,cos8. a) What is the relationship between R and O? b)If a particle with a charge g is placed at the origin,what is the total force on the particle? Show all your work including setting up and integrating any necessary integrals. Answer. (a)In order to find a relation between R and it is necessary to integrate the charge density A because the charge distribution is non-uniform Q=∫h=名,eos0Ra6=队sm9rn=2队. wire (b)The force on the charged particle at the center P of the semicircle is given by F(P)=qE(P). The electric field at the center P of the semicircle is given by (P)= ATEo wire The unit vector,f,located at the field point,is directed from the source to the field point and in Cartesian coordinates is given by f=-sin@'i-cos'j. 10
10 Problem 2 Electric field and force A positively charged wire is bent into a semicircle of radius R , as shown in the figure below. The total charge on the semicircle is Q . However, the charge per unit length along the semicircle is non-uniform and given by 0 ! = ! cos" . a) What is the relationship between !0 , R and Q ? b) If a particle with a charge q is placed at the origin, what is the total force on the particle? Show all your work including setting up and integrating any necessary integrals. Answer. (a) In order to find a relation between !0 , R and Q it is necessary to integrate the charge density ! because the charge distribution is non-uniform / 2 / 2 0 0 / 2 0 / 2 cos sin 2 wire Q ds Rd R R ! " ! " ! " ! " # # ! ! # ! # $= $= $=% $=% = = $ $ = $ = & & . (b) The force on the charged particle at the center P of the semicircle is given by F(P) = qE(P) ! ! . The electric field at the center P of the semicircle is given by 2 0 1 ( ) ˆ 4 wire ds P r ! "# = $ E r ! . The unit vector, rˆ , located at the field point, is directed from the source to the field point and in Cartesian coordinates is given by ˆ ˆ rˆ = #sin!" i # cos!" j