
Chapter 2 Coulomb's Law 2.1 Electric Charge.… 2-3 2.2 Coulomb's Law.............. 2-3 Animation 2.1:Van de Graaff Generator.................................. 2-4 2.3 Principle of Superposition.............. 2-5 Example 2.1:Three Charges.................... 2-5 2.4 Electric Field......... 2-7 Animation 2.2:Electric Field of Point Charges 2-8 2.5 Electric Field Lines....... 2-9 2.6 Force on a Charged Particle in an Electric Field 2-10 2.7 Electric Dipole… 2-11 2.7.1 The Electric Field of a Dipole. .2-12 Animation 2.3:Electric Dipole............ 2-13 2.8 Dipole in Electric Field........... .2-13 2.8.1 Potential Energy of an Electric Dipole ..2-14 2.9 Charge Density........... 2-16 2.9.1 Volume Charge Density................ 2-16 2.9.2 Surface Charge Density 2-17 2.9.3 Line Charge Density................ .2-17 2.10 Electric Fields due to Continuous Charge Distributions........................ .2-18 Example 2.2:Electric Field on the Axis of a Rod..... 2-18 Example 2.3:Electric Field on the Perpendicular Bisector.................. 2-19 Example 2.4:Electric Field on the Axis of a Ring.....................2-21 Example 2.5:Electric Field Due to a Uniformly Charged Disk.............................2-23 2.1ISummary 2-25 2.12 Problem-Solving Strategies.................... 2-27 2.13 Solved problems 2-29 2.13.1 Hydrogen Atom229 2.13.2 Millikan Oil-Drop Experiment .............. 2-30 2.13.3 Charge Moving Perpendicularly to an Electric Field 2-31 2.13.4 Electric Field of a Dipole................. 2-33 2-1
Chapter 2 Coulomb’s Law 2.1 Electric Charge.....................................................................................................2-3 2.2 Coulomb's Law ....................................................................................................2-3 Animation 2.1: Van de Graaff Generator...............................................................2-4 2.3 Principle of Superposition....................................................................................2-5 Example 2.1: Three Charges....................................................................................2-5 2.4 Electric Field........................................................................................................2-7 Animation 2.2: Electric Field of Point Charges.....................................................2-8 2.5 Electric Field Lines..............................................................................................2-9 2.6 Force on a Charged Particle in an Electric Field ...............................................2-10 2.7 Electric Dipole ...................................................................................................2-11 2.7.1 The Electric Field of a Dipole......................................................................2-12 Animation 2.3: Electric Dipole.............................................................................2-13 2.8 Dipole in Electric Field......................................................................................2-13 2.8.1 Potential Energy of an Electric Dipole ........................................................2-14 2.9 Charge Density...................................................................................................2-16 2.9.1 Volume Charge Density...............................................................................2-16 2.9.2 Surface Charge Density ...............................................................................2-17 2.9.3 Line Charge Density ....................................................................................2-17 2.10 Electric Fields due to Continuous Charge Distributions....................................2-18 Example 2.2: Electric Field on the Axis of a Rod .................................................2-18 Example 2.3: Electric Field on the Perpendicular Bisector...................................2-19 Example 2.4: Electric Field on the Axis of a Ring ................................................2-21 Example 2.5: Electric Field Due to a Uniformly Charged Disk............................2-23 2.11 Summary............................................................................................................2-25 2.12 Problem-Solving Strategies ...............................................................................2-27 2.13 Solved Problems ................................................................................................2-29 2.13.1 Hydrogen Atom ........................................................................................2-29 2.13.2 Millikan Oil-Drop Experiment .................................................................2-30 2.13.3 Charge Moving Perpendicularly to an Electric Field ...............................2-31 2.13.4 Electric Field of a Dipole..........................................................................2-33 2-1

2.13.5 Electric Field of an Arc-36 2.13.6 Electric Field Off the Axis of a Finite Rod.......................................2.-37 2.14 Conceptual Questions. 2-39 2.15 Additional Problems .2-40 2.15.1 Three Point Charges........ .2-40 2.15.2 Three point Charges..2-40 2.15.3 Four Point Charges 2-41 2.15.4 Semicircular Wire. 2-41 2.15.5 Electric Dipole.......... 2-42 2.15.6 Charged Cylindrical Shell and Cylinder 2-42 2.15.7 Two Conducting Balls....................... 2-43 2.15.8 Torque on an Electric Dipole................. .2-43 2-2
2.13.5 Electric Field of an Arc.............................................................................2-36 2.13.6 Electric Field Off the Axis of a Finite Rod...............................................2-37 2.14 Conceptual Questions........................................................................................2-39 2.15 Additional Problems..........................................................................................2-40 2.15.1 Three Point Charges..................................................................................2-40 2.15.2 Three Point Charges..................................................................................2-40 2.15.3 Four Point Charges ...................................................................................2-41 2.15.4 Semicircular Wire .....................................................................................2-41 2.15.5 Electric Dipole ..........................................................................................2-42 2.15.6 Charged Cylindrical Shell and Cylinder...................................................2-42 2.15.7 Two Conducting Balls..............................................................................2-43 2.15.8 Torque on an Electric Dipole....................................................................2-43 2-2
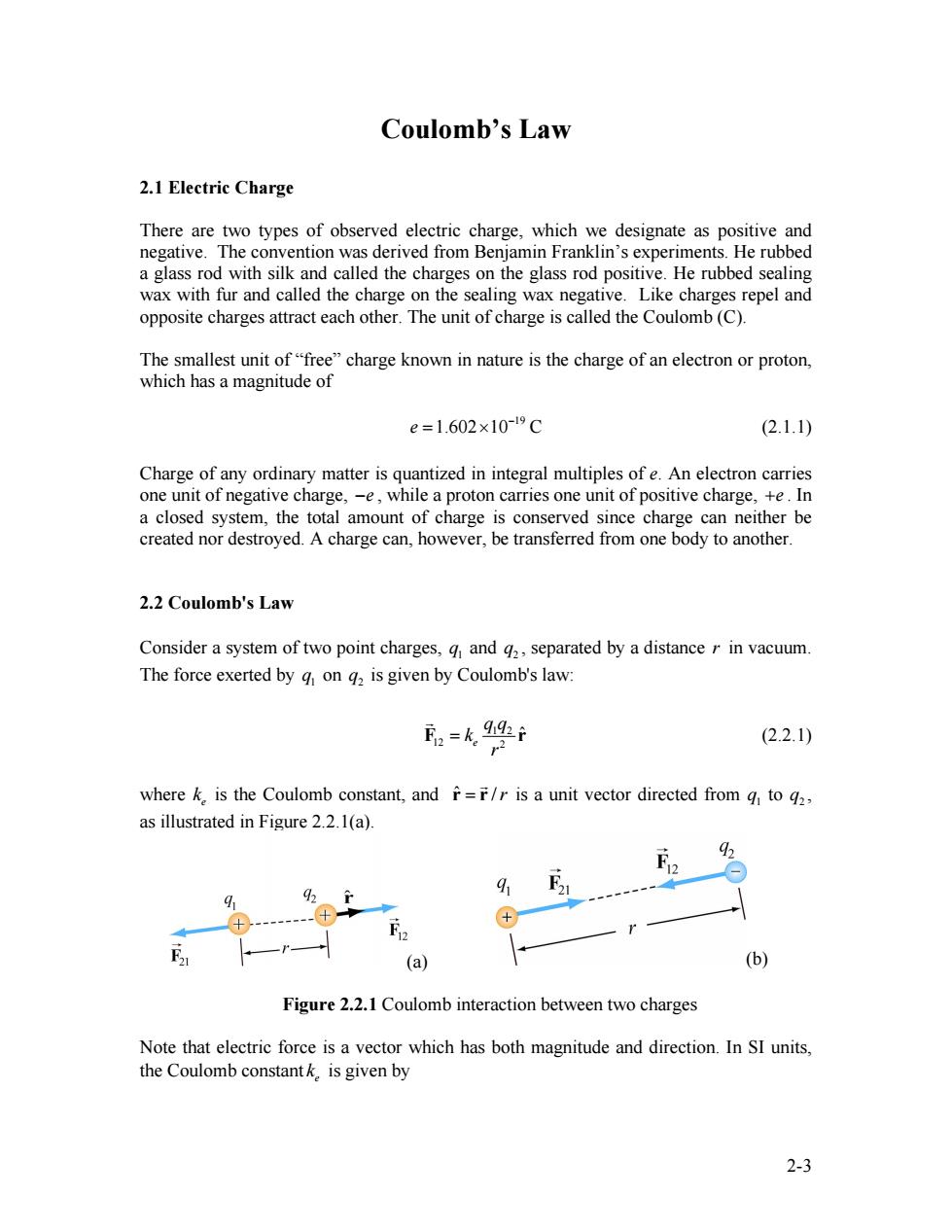
Coulomb's Law 2.1 Electric Charge There are two types of observed electric charge,which we designate as positive and negative.The convention was derived from Benjamin Franklin's experiments.He rubbed a glass rod with silk and called the charges on the glass rod positive.He rubbed sealing wax with fur and called the charge on the sealing wax negative.Like charges repel and opposite charges attract each other.The unit of charge is called the Coulomb(C). The smallest unit of"free"charge known in nature is the charge of an electron or proton, which has a magnitude of e=1.602×10-19C (2.1.1) Charge of any ordinary matter is quantized in integral multiples of e.An electron carries one unit of negative charge,-e,while a proton carries one unit of positive charge,+e.In a closed system,the total amount of charge is conserved since charge can neither be created nor destroyed.A charge can,however,be transferred from one body to another. 2.2 Coulomb's Law Consider a system of two point charges,g and g2,separated by a distance r in vacuum The force exerted by g on g2 is given by Coulomb's law: F2=k 9i (2.2.1) 2 where k.is the Coulomb constant,and r=r/r is a unit vector directed from g to g2, as illustrated in Figure 2.2.1(a). 0 + (a) (b) Figure 2.2.1 Coulomb interaction between two charges Note that electric force is a vector which has both magnitude and direction.In SI units, the Coulomb constantk.is given by 2-3
Coulomb’s Law 2.1 Electric Charge There are two types of observed electric charge, which we designate as positive and negative. The convention was derived from Benjamin Franklin’s experiments. He rubbed a glass rod with silk and called the charges on the glass rod positive. He rubbed sealing wax with fur and called the charge on the sealing wax negative. Like charges repel and opposite charges attract each other. The unit of charge is called the Coulomb (C). The smallest unit of “free” charge known in nature is the charge of an electron or proton, which has a magnitude of (2.1.1) 19 e 1.602 10 C − = × Charge of any ordinary matter is quantized in integral multiples of e. An electron carries one unit of negative charge, −e , while a proton carries one unit of positive charge, +e . In a closed system, the total amount of charge is conserved since charge can neither be created nor destroyed. A charge can, however, be transferred from one body to another. 2.2 Coulomb's Law Consider a system of two point charges, and , separated by a distance in vacuum. The force exerted by on is given by Coulomb's law: 1 q 2 q r 1 q 2 q 1 2 12 2 ˆ e q q k r F = r G (2.2.1) where is the Coulomb constant, and e k r r ˆ = /r G is a unit vector directed from to , as illustrated in Figure 2.2.1(a). 1 q 2 q (a) (b) Figure 2.2.1 Coulomb interaction between two charges Note that electric force is a vector which has both magnitude and direction. In SI units, the Coulomb constant is given by e k 2-3

1=89875×10N.m21C k。二4π (2.2.2) where 8=4899x10N-m/C-8.85x10-CN-m (2.2.3) is known as the "permittivity of free space."Similarly,the force on g due to g2 is given by F=-F2,as illustrated in Figure 2.2.1(b).This is consistent with Newton's third law. As an example,consider a hydrogen atom in which the proton (nucleus)and the electron are separated by a distance r=5.3x10-m.The electrostatic force between the two particles is approximately F=ke2/r2=8.2x10N.On the other hand,one may show that the gravitational force is only F3.6x10-47N.Thus,gravitational effect can be neglected when dealing with electrostatic forces! Animation 2.1:Van de Graaff Generator Consider Figure 2.2.2(a)below.The figure illustrates the repulsive force transmitted between two objects by their electric fields.The system consists of a charged metal sphere of a van de Graaff generator.This sphere is fixed in space and is not free to move. The other object is a small charged sphere that is free to move(we neglect the force of gravity on this sphere).According to Coulomb's law,these two like charges repel each another.That is,the small sphere experiences a repulsive force away from the van de Graaff sphere. 市m3本 Figure 2.2.2 (a)Two charges of the same sign that repel one another because of the “stresses'”transmitted by electric fields.We use both the“grass seeds”representation and the "field lines"representation of the electric field of the two charges.(b)Two charges of opposite sign that attract one another because of the stresses transmitted by electric fields. The animation depicts the motion of the small sphere and the electric fields in this situation.Note that to repeat the motion of the small sphere in the animation,we have 2-4
9 2 0 1 8.9875 10 N m /C 4 e k πε = = × ⋅ 2 (2.2.2) where 12 2 2 0 9 2 2 1 8 85 10 C N m 4 (8.99 10 N m C ) ε . π − = = × × ⋅ ⋅ (2.2.3) is known as the “permittivity of free space.” Similarly, the force on due to is given by , as illustrated in Figure 2.2.1(b). This is consistent with Newton's third law. 1 q 2 q F21 = −F12 G G As an example, consider a hydrogen atom in which the proton (nucleus) and the electron are separated by a distance . The electrostatic force between the two particles is approximately . On the other hand, one may show that the gravitational force is only . Thus, gravitational effect can be neglected when dealing with electrostatic forces! 11 r 5.3 10 m − = × 2 2 8 F k e ee /r 8.2 10 N − = = × 47 F g 3.6 10 N − ≈ × Animation 2.1: Van de Graaff Generator Consider Figure 2.2.2(a) below. The figure illustrates the repulsive force transmitted between two objects by their electric fields. The system consists of a charged metal sphere of a van de Graaff generator. This sphere is fixed in space and is not free to move. The other object is a small charged sphere that is free to move (we neglect the force of gravity on this sphere). According to Coulomb’s law, these two like charges repel each another. That is, the small sphere experiences a repulsive force away from the van de Graaff sphere. Figure 2.2.2 (a) Two charges of the same sign that repel one another because of the “stresses” transmitted by electric fields. We use both the “grass seeds” representation and the ”field lines” representation of the electric field of the two charges. (b) Two charges of opposite sign that attract one another because of the stresses transmitted by electric fields. The animation depicts the motion of the small sphere and the electric fields in this situation. Note that to repeat the motion of the small sphere in the animation, we have 2-4

the small sphere "bounce off'of a small square fixed in space some distance from the van de Graaff generator. Before we discuss this animation,consider Figure 2.2.2(b),which shows one frame of a movie of the interaction of two charges with opposite signs.Here the charge on the small sphere is opposite to that on the van de Graaff sphere.By Coulomb's law,the two objects now attract one another,and the small sphere feels a force attracting it toward the van de Graaff.To repeat the motion of the small sphere in the animation,we have that charge "bounce off'of a square fixed in space near the van de Graaff. The point of these two animations is to underscore the fact that the Coulomb force between the two charges is not "action at a distance."Rather,the stress is transmitted by direct "contact"from the van de Graaff to the immediately surrounding space,via the electric field of the charge on the van de Graaff.That stress is then transmitted from one element of space to a neighboring element,in a continuous manner,until it is transmitted to the region of space contiguous to the small sphere,and thus ultimately to the small sphere itself.Although the two spheres are not in direct contact with one another,they are in direct contact with a medium or mechanism that exists between them.The force between the small sphere and the van de Graaff is transmitted (at a finite speed)by stresses induced in the intervening space by their presence. Michael Faraday invented field theory;drawing "lines of force"or "field lines"was his way of representing the fields.He also used his drawings of the lines of force to gain insight into the stresses that the fields transmit.He was the first to suggest that these fields,which exist continuously in the space between charged objects,transmit the stresses that result in forces between the objects. 2.3 Principle of Superposition Coulomb's law applies to any pair of point charges.When more than two charges are present,the net force on any one charge is simply the vector sum of the forces exerted on it by the other charges.For example,if three charges are present,the resultant force experienced by g3 due to g and g2 will be F3=F3+F23 (2.3.1) The superposition principle is illustrated in the example below. Example 2.1:Three Charges Three charges are arranged as shown in Figure 2.3.1.Find the force on the charge gs assuming that g=6.0×10-6C,q2=-q,=-6.0×10-6C,q3=+3.0×10-6Cand a=2.0×10-2m. 2-5
the small sphere “bounce off” of a small square fixed in space some distance from the van de Graaff generator. Before we discuss this animation, consider Figure 2.2.2(b), which shows one frame of a movie of the interaction of two charges with opposite signs. Here the charge on the small sphere is opposite to that on the van de Graaff sphere. By Coulomb’s law, the two objects now attract one another, and the small sphere feels a force attracting it toward the van de Graaff. To repeat the motion of the small sphere in the animation, we have that charge “bounce off” of a square fixed in space near the van de Graaff. The point of these two animations is to underscore the fact that the Coulomb force between the two charges is not “action at a distance.” Rather, the stress is transmitted by direct “contact” from the van de Graaff to the immediately surrounding space, via the electric field of the charge on the van de Graaff. That stress is then transmitted from one element of space to a neighboring element, in a continuous manner, until it is transmitted to the region of space contiguous to the small sphere, and thus ultimately to the small sphere itself. Although the two spheres are not in direct contact with one another, they are in direct contact with a medium or mechanism that exists between them. The force between the small sphere and the van de Graaff is transmitted (at a finite speed) by stresses induced in the intervening space by their presence. Michael Faraday invented field theory; drawing “lines of force” or “field lines” was his way of representing the fields. He also used his drawings of the lines of force to gain insight into the stresses that the fields transmit. He was the first to suggest that these fields, which exist continuously in the space between charged objects, transmit the stresses that result in forces between the objects. 2.3 Principle of Superposition Coulomb’s law applies to any pair of point charges. When more than two charges are present, the net force on any one charge is simply the vector sum of the forces exerted on it by the other charges. For example, if three charges are present, the resultant force experienced by due to and will be 3 q 1 q 2 q F F 3 1 = +3 F23 G G G (2.3.1) The superposition principle is illustrated in the example below. Example 2.1: Three Charges Three charges are arranged as shown in Figure 2.3.1. Find the force on the charge assuming that , , and . 3 q 6 1 q 6.0 10 C− = × 6 2 1 q q 6.0 10 C− = − = − × 6 3 q 3.0 10 C− = + × 2 a 2.0 10 m− = × 2-5
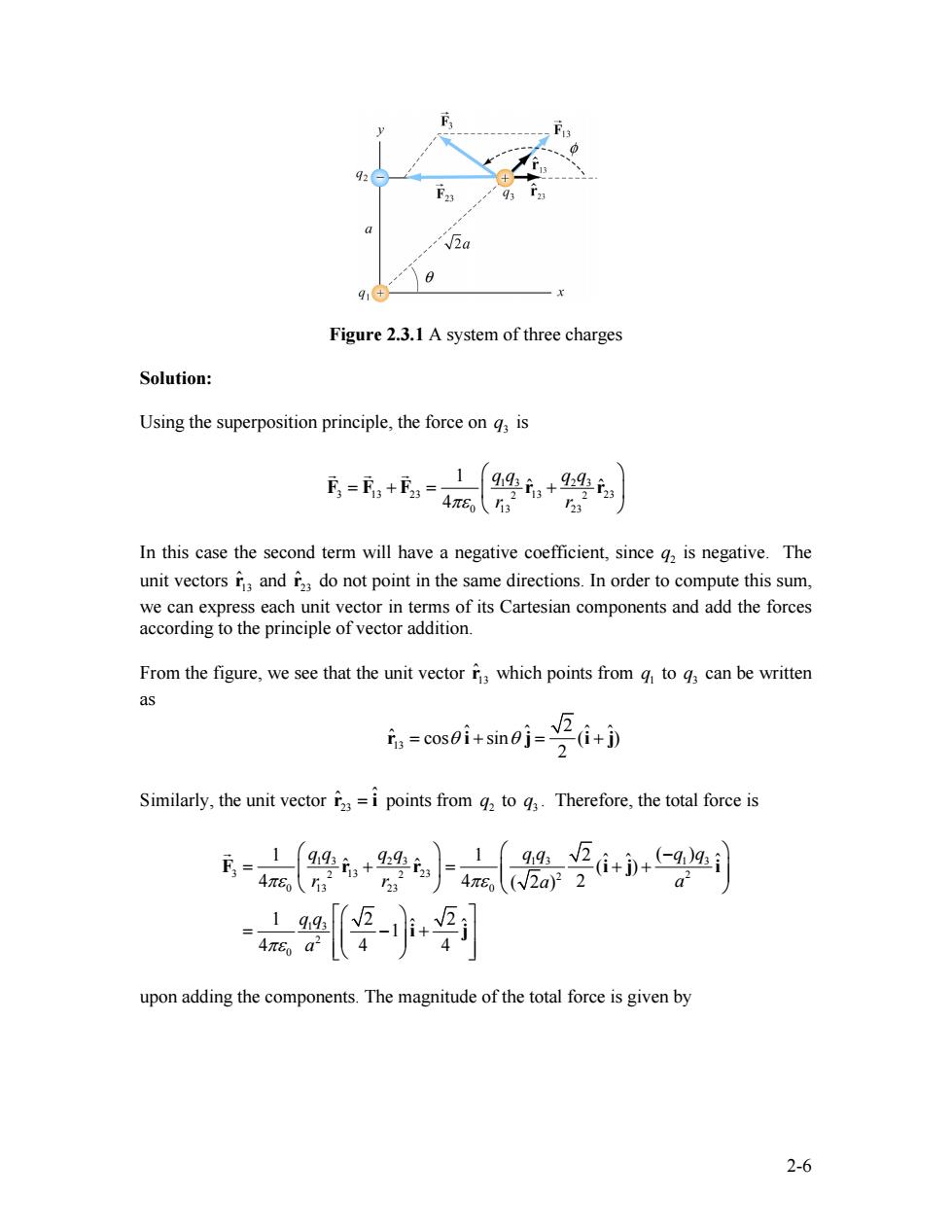
1 92- a 91+ Figure 2.3.1 A system of three charges Solution: Using the superposition principle,the force on g:is 10 E=+= 49i+99i In this case the second term will have a negative coefficient,since g2 is negative.The unit vectors and do not point in the same directions.In order to compute this sum, we can express each unit vector in terms of its Cartesian components and add the forces according to the principle of vector addition. From the figure,we see that the unit vector r which points from g to g3 can be written as -oim0j-9i+i Similarly,the unit vector=i points from to Therefore,the total force is F= 1 警+学小{%n, (2a)22 1 4T60 upon adding the components.The magnitude of the total force is given by 2-6
Figure 2.3.1 A system of three charges Solution: Using the superposition principle, the force on is 3 q 1 3 2 3 3 13 23 2 2 13 23 0 13 23 1 ˆ ˆ 4 q q q q πε r r ⎛ ⎞ = + = ⎜ ⎟ + ⎝ ⎠ F F F r r G G G In this case the second term will have a negative coefficient, since is negative. The unit vectors and do not point in the same directions. In order to compute this sum, we can express each unit vector in terms of its Cartesian components and add the forces according to the principle of vector addition. 2 q 13 rˆ 23 rˆ From the figure, we see that the unit vector which points from to can be written as 13 rˆ 1 q 3 q 13 2 ˆ ˆ ˆ ˆ cos sin ( ) 2 ˆ r i = θ θ + =j i + j Similarly, the unit vector points from to . Therefore, the total force is 23 ˆ rˆ = i 2 q 3 q 1 3 2 3 1 3 1 3 3 1 2 2 3 23 2 2 0 13 23 0 1 3 2 0 1 1 2 ( ) ˆ ˆ ˆ ˆ ˆ ( ) 4 4 ( 2 ) 2 1 2 2 ˆ ˆ 1 4 4 4 q q q q q q q q r r a a q q a πε πε πε ⎛ ⎞ ⎛ ⎞ − = + ⎜ ⎟ = ⎜ ⎟ + + ⎝ ⎠ ⎝ ⎠ ⎡ ⎤ ⎛ ⎞ = − ⎢ ⎥ ⎜ ⎟ + ⎣ ⎦ ⎝ ⎠ F r r i j i i j G upon adding the components. The magnitude of the total force is given by 2-6

-婴停-j =9.0x10N.m21c6.0x10C30x10*C0.74=30N (2.0×10-2m)2 The angle that the force makes with the positive x-axis is tar tan-i 514 =151.3° -1+V2/4 Note there are two solutions to this equation.The second solution 6=-28.7 is incorrect because it would indicate that the force has positive i and negative j components For a system of N charges,the net force experienced by the jth particle would be (2.3.2) i法j where F denotes the force between particles i and j.The superposition principle implies that the net force between any two charges is independent of the presence of other charges.This is true if the charges are in fixed positions. 2.4 Electric Field The electrostatic force,like the gravitational force,is a force that acts at a distance,even when the objects are not in contact with one another.To justify such the notion we rationalize action at a distance by saying that one charge creates a field which in turn acts on the other charge. An electric charge g produces an electric field everywhere.To quantify the strength of the field created by that charge,we can measure the force a positive "test charge"o experiences at some point.The electric field E is defined as: E=limE (2.4.1) 9%0q0 We take goto be infinitesimally small so that the field go generates does not disturb the "source charges."The analogy between the electric field and the gravitational field g=lim F/m is depicted in Figure 2.4.1. 2-7
1 2 2 2 1 3 3 2 0 6 6 9 2 2 2 2 1 2 2 1 4 4 4 (6.0 10 C)(3.0 10 C) (9.0 10 N m /C ) (0.74) 3.0 N (2.0 10 m) q q F πε a − − − ⎡ ⎤ ⎛ ⎞ ⎛ ⎞ = − ⎢ ⎥ ⎜ ⎟ + ⎜ ⎟ ⎜ ⎟ ⎢ ⎥ ⎝ ⎠ ⎝ ⎠ ⎣ ⎦ × × = × ⋅ = × The angle that the force makes with the positive x -axis is 1 1 3, 3, 2 / 4 tan tan 151.3 1 2 / 4 y x F F φ − − ⎛ ⎞ ⎡ ⎤ = = ⎜ ⎟ ⎢ ⎥ = ⎜ ⎟ ⎣ ⎦ − + ⎝ ⎠ ° Note there are two solutions to this equation. The second solution φ = −28.7° is incorrect because it would indicate that the force has positive and negative ˆ ˆ i j components. For a system of N charges, the net force experienced by the jth particle would be 1 N j i i j = ≠ F = ∑Fij G G (2.3.2) where denotes the force between particles i and Fij G j . The superposition principle implies that the net force between any two charges is independent of the presence of other charges. This is true if the charges are in fixed positions. 2.4 Electric Field The electrostatic force, like the gravitational force, is a force that acts at a distance, even when the objects are not in contact with one another. To justify such the notion we rationalize action at a distance by saying that one charge creates a field which in turn acts on the other charge. An electric charge q produces an electric field everywhere. To quantify the strength of the field created by that charge, we can measure the force a positive “test charge” experiences at some point. The electric field E 0 q G is defined as: 0 0 0 lim e q → q F E = G G (2.4.1) We take to be infinitesimally small so that the field generates does not disturb the “source charges.” The analogy between the electric field and the gravitational field is depicted in Figure 2.4.1. 0 q 0 q 0 0 0 lim / m m m → g = F G G 2-7
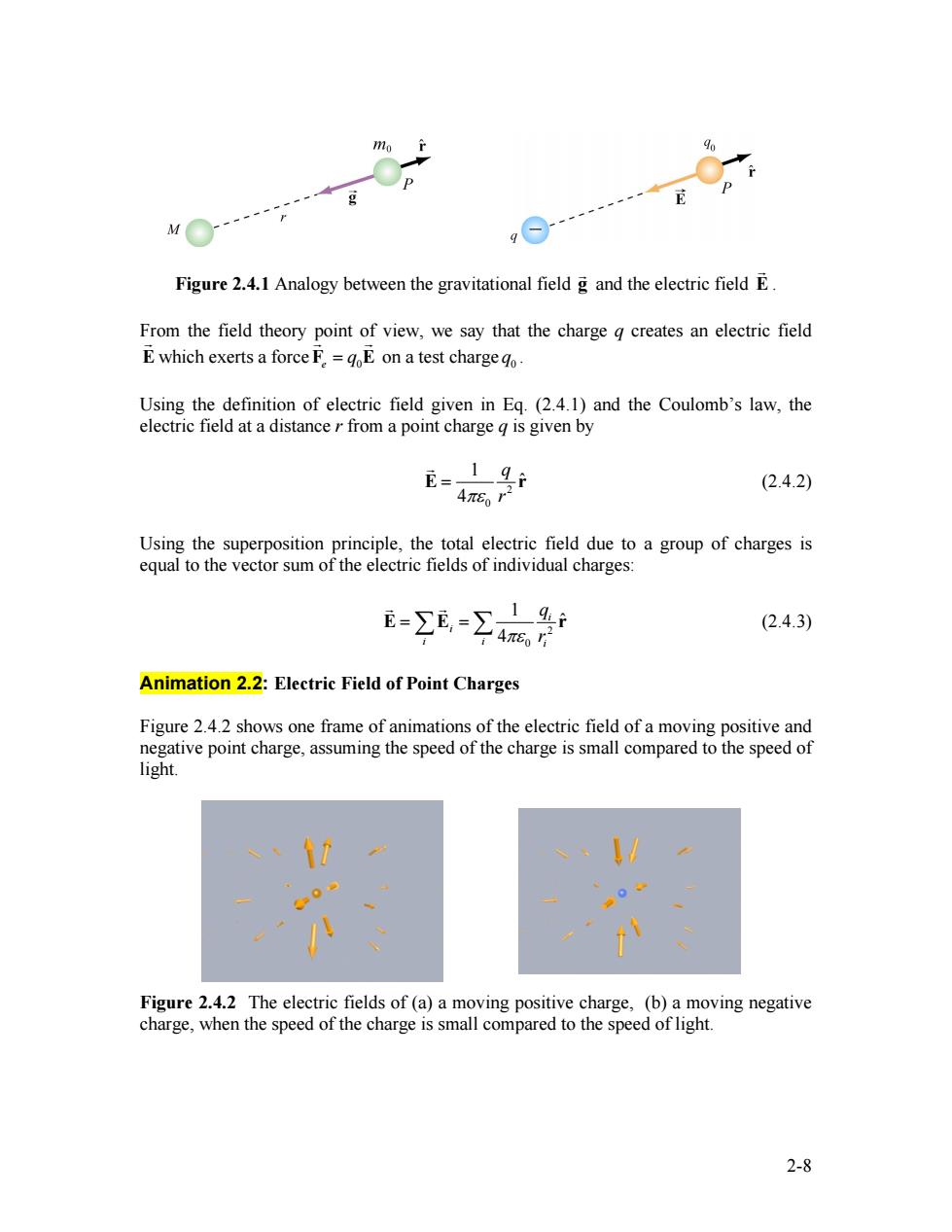
m Figure 2.4.1 Analogy between the gravitational field g and the electric field E. From the field theory point of view,we say that the charge g creates an electric field E which exerts a forceF=oE on a test charge do. Using the definition of electric field given in Eq.(2.4.1)and the Coulomb's law,the electric field at a distance r from a point charge g is given by E=,19: (2.4.2) 4π60r1 Using the superposition principle,the total electric field due to a group of charges is equal to the vector sum of the electric fields of individual charges: 重-2E-2是 (2.4.3) Animation 2.2:Electric Field of Point Charges Figure 2.4.2 shows one frame of animations of the electric field of a moving positive and negative point charge,assuming the speed of the charge is small compared to the speed of light. Figure 2.4.2 The electric fields of(a)a moving positive charge,(b)a moving negative charge,when the speed of the charge is small compared to the speed of light. 2-8
Figure 2.4.1 Analogy between the gravitational field g G and the electric field E . G From the field theory point of view, we say that the charge q creates an electric field E which exerts a force on a test charge . G e 0 F = q G GE 0 q Using the definition of electric field given in Eq. (2.4.1) and the Coulomb’s law, the electric field at a distance r from a point charge q is given by 2 0 1 ˆ 4 q πε r E = r G (2.4.2) Using the superposition principle, the total electric field due to a group of charges is equal to the vector sum of the electric fields of individual charges: 2 0 1 ˆ 4 i i i i i q πε r E E = = ∑ ∑ r G G (2.4.3) Animation 2.2: Electric Field of Point Charges Figure 2.4.2 shows one frame of animations of the electric field of a moving positive and negative point charge, assuming the speed of the charge is small compared to the speed of light. Figure 2.4.2 The electric fields of (a) a moving positive charge, (b) a moving negative charge, when the speed of the charge is small compared to the speed of light. 2-8

2.5 Electric Field Lines Electric field lines provide a convenient graphical representation of the electric field in space.The field lines for a positive and a negative charges are shown in Figure 2.5.1. (b) Figure 2.5.1 Field lines for (a)positive and (b)negative charges. Notice that the direction of field lines is radially outward for a positive charge and radially inward for a negative charge.For a pair of charges of equal magnitude but opposite sign(an electric dipole),the field lines are shown in Figure 2.5.2. Figure 2.5.2 Field lines for an electric dipole The pattern of electric field lines can be obtained by considering the following: (1)Symmetry:For every point above the line joining the two charges there is an equivalent point below it.Therefore,the pattern must be symmetrical about the line joining the two charges (2)Near field:Very close to a charge,the field due to that charge predominates. Therefore,the lines are radial and spherically symmetric. (3)Far field:Far from the system of charges,the pattern should look like that of a single point charge of value =Thus,the lines should be radially outward,unless Q=0. (4)Null point:This is a point at which E=0,and no field lines should pass through it. 2-9
2.5 Electric Field Lines Electric field lines provide a convenient graphical representation of the electric field in space. The field lines for a positive and a negative charges are shown in Figure 2.5.1. (a) (b) Figure 2.5.1 Field lines for (a) positive and (b) negative charges. Notice that the direction of field lines is radially outward for a positive charge and radially inward for a negative charge. For a pair of charges of equal magnitude but opposite sign (an electric dipole), the field lines are shown in Figure 2.5.2. Figure 2.5.2 Field lines for an electric dipole. The pattern of electric field lines can be obtained by considering the following: (1) Symmetry: For every point above the line joining the two charges there is an equivalent point below it. Therefore, the pattern must be symmetrical about the line joining the two charges (2) Near field: Very close to a charge, the field due to that charge predominates. Therefore, the lines are radial and spherically symmetric. (3) Far field: Far from the system of charges, the pattern should look like that of a single point charge of value . Thus, the lines should be radially outward, unless . i i Q = ∑ Q Q = 0 (4) Null point: This is a point at which E = 0 G G , and no field lines should pass through it. 2-9

The properties of electric field lines may be summarized as follows: The direction of the electric field vector E at a point is tangent to the field lines. The number of lines per unit area through a surface perpendicular to the line is devised to be proportional to the magnitude of the electric field in a given region. The field lines must begin on positive charges(or at infinity)and then terminate on negative charges (or at infinity). The number of lines that originate from a positive charge or terminating on a negative charge must be proportional to the magnitude of the charge. No two field lines can cross each other;otherwise the field would be pointing in two different directions at the same point. 2.6 Force on a Charged Particle in an Electric Field Consider a charge +q moving between two parallel plates of opposite charges,as shown in Figure 2.6.1. v=0 ⊕g Figure 2.6.1 Charge moving in a constant electric field Let the electric field between the plates be E=-E j,with E0.(In Chapter 4,we shall show that the electric field in the region between two infinitely large plates of opposite charges is uniform.)The charge will experience a downward Coulomb force F=gE (2.6.1) Note the distinction between the charge q that is experiencing a force and the charges on the plates that are the sources of the electric field.Even though the charge g is also a source of an electric field,by Newton's third law,the charge cannot exert a force on itself.Therefore,E is the field that arises from the"source"charges only. According to Newton's second law,a net force will cause the charge to accelerate with an acceleration 2-10
The properties of electric field lines may be summarized as follows: • The direction of the electric field vector E G at a point is tangent to the field lines. • The number of lines per unit area through a surface perpendicular to the line is devised to be proportional to the magnitude of the electric field in a given region. • The field lines must begin on positive charges (or at infinity) and then terminate on negative charges (or at infinity). • The number of lines that originate from a positive charge or terminating on a negative charge must be proportional to the magnitude of the charge. • No two field lines can cross each other; otherwise the field would be pointing in two different directions at the same point. 2.6 Force on a Charged Particle in an Electric Field Consider a charge moving between two parallel plates of opposite charges, as shown in Figure 2.6.1. +q Figure 2.6.1 Charge moving in a constant electric field Let the electric field between the plates be ˆ E = −E y j G , with . (In Chapter 4, we shall show that the electric field in the region between two infinitely large plates of opposite charges is uniform.) The charge will experience a downward Coulomb force 0 E y > e F = qE G G (2.6.1) Note the distinction between the charge that is experiencing a force and the charges on the plates that are the sources of the electric field. Even though the charge is also a source of an electric field, by Newton’s third law, the charge cannot exert a force on itself. Therefore, E G is the field that arises from the “source” charges only. q q According to Newton’s second law, a net force will cause the charge to accelerate with an acceleration 2-10