
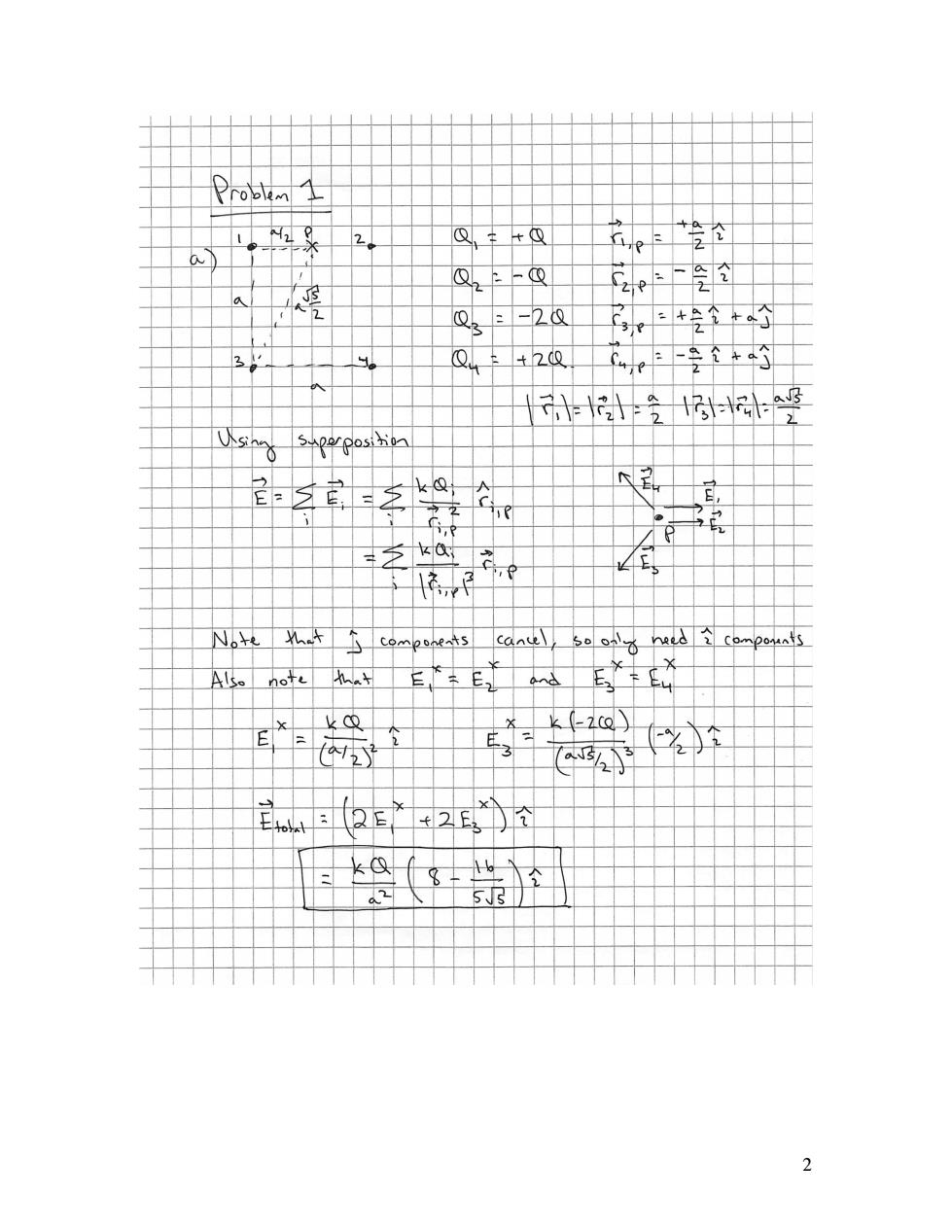
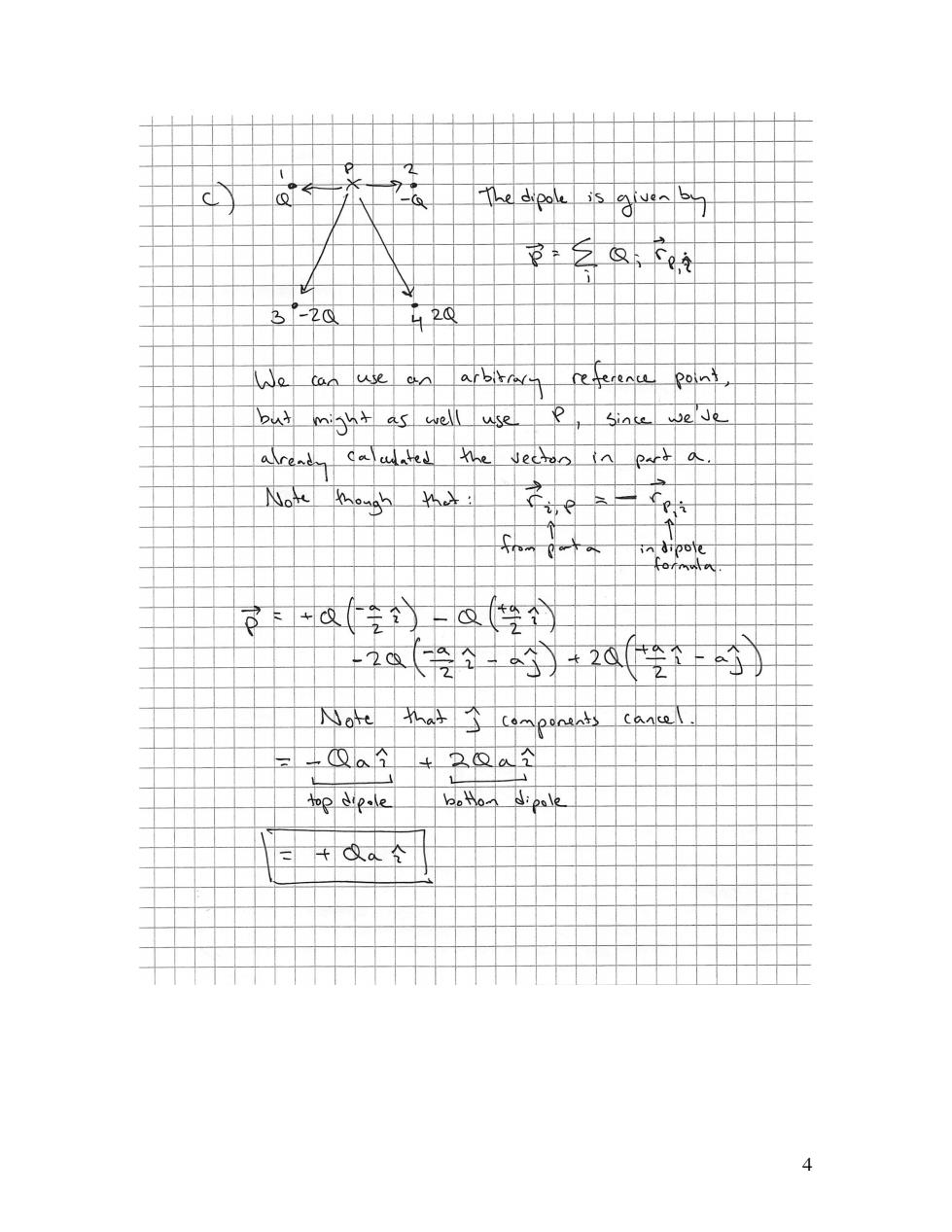
MASSACHUSETTS INSTITUTE OF TECHNOLOGY Department of Physics 8.02 Spring 2014 Exam One Solutions Problem 1(25 points):answers without work shown will not be given any credit. Four point-like objects of charge-20,+,-O,and +20 respectively are placed at the corners of a square of side a as shown in the figure below. +0 -0 a/2 a/2 +x + -2Q +2Q Part(a) Determine the electric field at the point P,which has coordinates()=(a/2,a) Express your answer in terms of k=1/4re,,a,i,and j as needed. Part(b) Choose infinity to be the zero point for the electric potential (V()=0).Determine the electric potential at the point P,which has coordinates (x)=(a/2,a).Express your answer in terms of k=1/4,a,i,and j as needed. Part(c) Determine the direction and magnitude of the electric dipole moment of this charge configuration.Express your answer in terms of k=1/4re,,a,i,and j as needed. 1
1 MASSACHUSETTS INSTITUTE OF TECHNOLOGY Department of Physics 8.02 Spring 2014 Exam One Solutions Problem 1 (25 points): answers without work shown will not be given any credit. Four point-like objects of charge −2Q , +Q , −Q , and +2Q respectively are placed at the corners of a square of side a as shown in the figure below. Part (a) Determine the electric field at the point P , which has coordinates (xP , yP ) = (a / 2,a). Express your answer in terms of k = 1/ 4πε 0 , Q, a , ˆ i , and ˆ j as needed. Part (b) Choose infinity to be the zero point for the electric potential (V (∞) = 0 ). Determine the electric potential at the point P , which has coordinates (xP , yP ) = (a / 2,a). Express your answer in terms of k = 1/ 4πε 0 , Q , a , ˆ i , and ˆ j as needed. Part (c) Determine the direction and magnitude of the electric dipole moment of this charge configuration. Express your answer in terms of k = 1/ 4πε 0 , Q , a , ˆ i , and ˆ j as needed
Probkm 2 Q++双 中的 一双 的 Q -2Q ++的 。 +2Q 引2t引3 s shpposibim 25 N。+eL 礼F componets cantel hse componnts AI5。 nott 九于 E and E日 业Q Xk(-2C t 3 宝回 k⑨ 53 2
2

+Q c V+∞)a -2 +2设 Nole 82 The same is bhu foc So h pofeak. P:o s n4s4 be caw¥hgi5oSn5t owo di eole5 and siagk dipou equi polmsials look Lile is 的个 ⊙ ps3中9 v70 VO V-6 pecpendic lisecfo 3
3
+个了N 7 -6 The dipoleis ah fet 3-2d 120 We u52 ce serence point but m3+ Sinte deJo alresi calud led 山o Fi,e 冠下+双()-Q(分 Note a于 cancel Qa +2Q公 too dipsle boton dipole =+Q& 4
4

v Sehine A 12 eoints L0fe1 7 1:414 dedhetons ouiclaepins 心 + Cance -3 3. not sib E evegukere 3 31 ecror i 31 3的4ro4 老F&s -21 componeat 2 calalho 日&。 a人db mislake 士2 oJal elcoc h小 生aA5ipom etror on las' 5
5

可 6 Qoints fotel ceductros oupap ∽e thinki a V was (.43 i o -4: No■ cealitic ha&4s的 -3 Bandom sign ercocS d 可 PorA13 Y Reia+已e anctions -5 甲a5als,0cwc-y4hn 一 is a vecfo - Doin o。hycembis4iorq)p4ir :orin 士cinon0mth中以 0 -1 3■ onS w:☒ 的9 ecrocs +24W8 o 49f西r 2.OUerall s的7 6
6

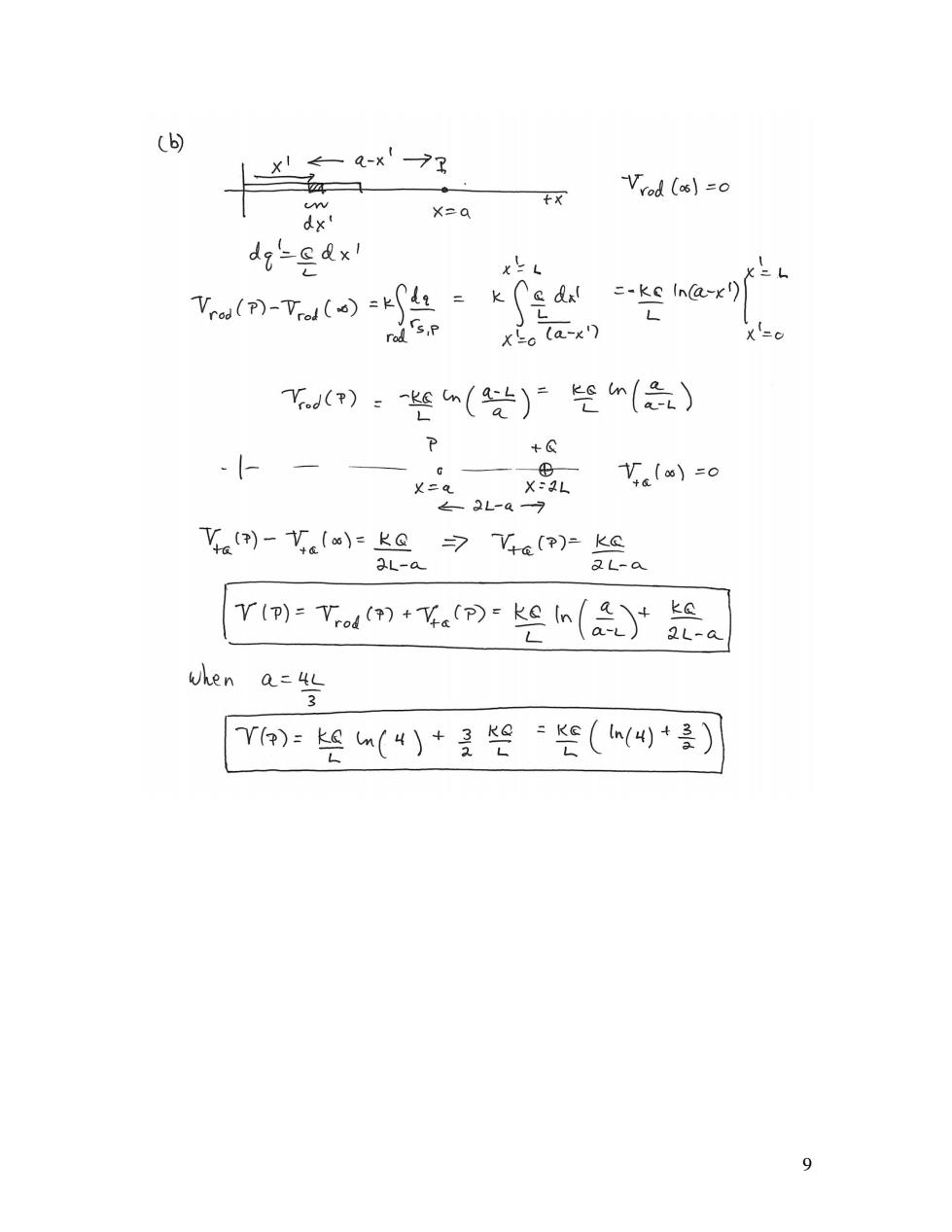
Problem 2(25 points):answers without work shown will not be given any credit. A stick with length L has a positive charge O uniformly distributed on it.It lies along the x-axis between the points x=0 and x=L.A point-like object with identical positive charge O lies on the x-axis at the point x=2L. uniformly charged stick point-like object of length L and charge O with charge O x=0 x=L x=a x=2L You may find the following integrals useful: 人 =-ln(a-x) x dx J (a-x)(a-x) jeya严 dx 1 xdx 1 62+y Part(a) Let x=a be the point on the x-axis between the two objects where the electric field is zero.Find a.Express your answer in terms of k=1/4,,and L as needed. Part(b) Choose the zero point for the electric potential to be at infinity V()=0.Determine the electric potential at the point x=a.You may express your answer in terms of any of the quantities mentioned in part (a),whether or not you answered part (a);in particular in terms of k=1/4n,,L,and a as needed. 7
7 Problem 2 (25 points): answers without work shown will not be given any credit. A stick with length L has a positive charge Q uniformly distributed on it. It lies along the x -axis between the points x = 0 and x = L . A point-like object with identical positive charge Q lies on the x -axis at the point x = 2L . You may find the following integrals useful: dx a − x = −ln(a − x) ∫ dx (a − x) 2 = 1 (a − x) ∫ dx (x 2 + y 2 ) 3/2 = 1 y 2 x (x 2 + y 2 ) ∫ 1/2 xdx (x 2 + y 2 ) 3/2 = − 1 (x 2 + y 2 ) ∫ 1/2 Part (a) Let x = a be the point on the x -axis between the two objects where the electric field is zero. Find a . Express your answer in terms of k = 1/ 4πε 0 , Q , and L as needed. Part (b) Choose the zero point for the electric potential to be at infinity V (∞) = 0 . Determine the electric potential at the point x = a . You may express your answer in terms of any of the quantities mentioned in part (a), whether or not you answered part (a); in particular in terms of k = 1/ 4πε 0 , Q , L , and a as needed

ccblcm 2 (c) dg=sax! P X=a X=L Erod()=k k L X=o X-。(a-x2 yL CR- :e()9 ←2L-&7 K=( Ee(m:k&,(-) 2L-a)2 E(P)=Er(p)+E。()-⊙ (e()经)父à 习 t(i)ca 之 (a-L)a BL-a →@L-)=@-1)a> 42-4La+a2-a2-4L今4L2=3L之 =4L 8
8
(6) ←-x73 a口 +X Vrod los)=o w dx X=a dj-cdx! x上L x-L e-kcIn@~x) rod s,P XEo la-x') (=c ):(g)m(是 +& G X二气 X=AL Vs(o)=0 ∠2L-47 Vo(3)-Vs(0)-KQ Vc(?)-kc aL-a L-a V)-V(nv()kei(t ke 2L-& ena二4 Ym)=竖n(4)+是e=((4)+) 9
9

Problem Two gfading scfeme Rav+(a\13fa(pe十s 它e4(woltk+6Pan古 Eig(p)woorte t3 pots E(P)二Ered(+Ee〔 )+1Po cocect inteqvation ti poit coroo soluton foc a +2 pomts Pirt (6)12 tofa(poils Vrod(p) +6 poinls Via (p)+3 point corloction integration +l point svperpositicn Trod ()+V(p)=vre)+a points 10
10