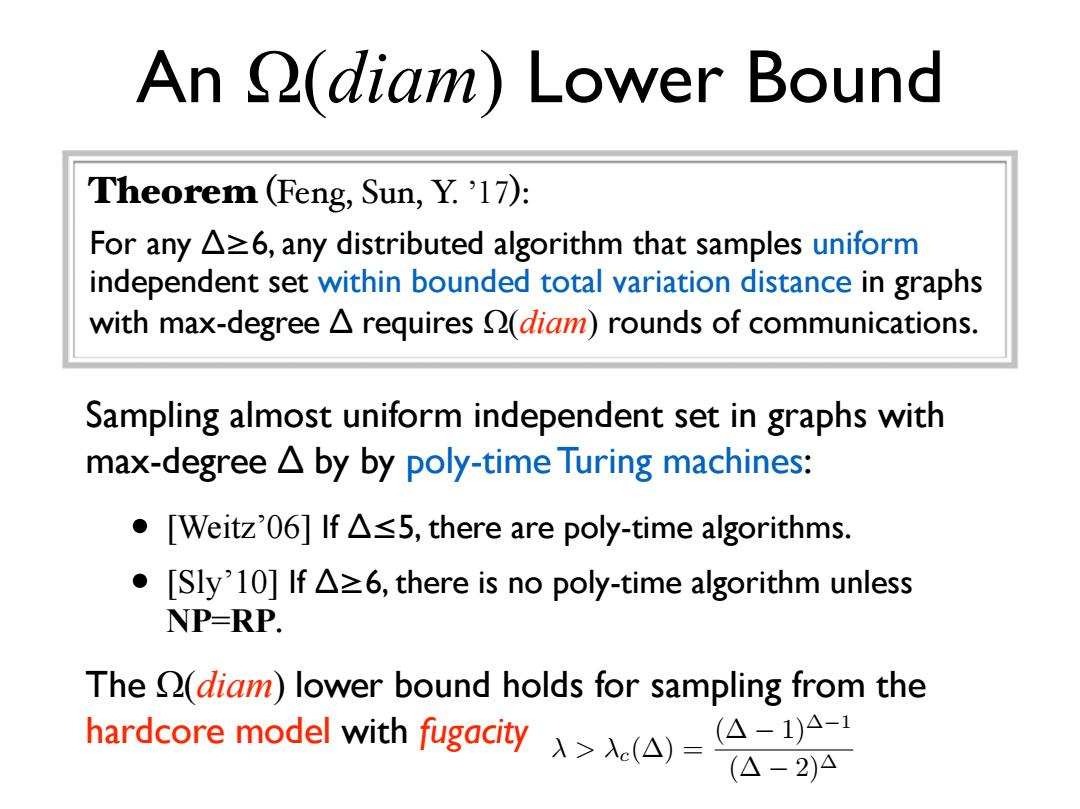
An (diam)Lower Bound Theorem (Feng,Sun,Y.'17): For any△≥6，any distributed algorithm that samples uniform independent set within bounded total variation distance in graphs with max-degree A requires (diam)rounds of communications. Sampling almost uniform independent set in graphs with max-degree A byby poly-time Turing machines: ●[Weitz06]lf△≤5，there are poly--time algorithms. [Sly'l0]lf△≥6，there is no poly-time algorithm unless NP=RP. The (diam)lower bound holds for sampling from the hardcore model with fugacity(A-1) (△-2)△• [Weitz’06] If ∆≤5, there are poly-time algorithms. • [Sly’10] If ∆≥6, there is no poly-time algorithm unless NP=RP. Sampling almost uniform independent set in graphs with max-degree ∆ by by poly-time Turing machines: The Ω(diam) lower bound holds for sampling from the hardcore model with fugacity ￾ > ￾c(￾) = (￾ ￾ 1)￾￾1 (￾ ￾ 2)￾ An Ω(diam) Lower Bound Theorem (Feng, Sun, Y. ’17): For any ∆≥6, any distributed algorithm that samples uniform independent set within bounded total variation distance in graphs with max-degree ∆ requires Ω(diam) rounds of communications