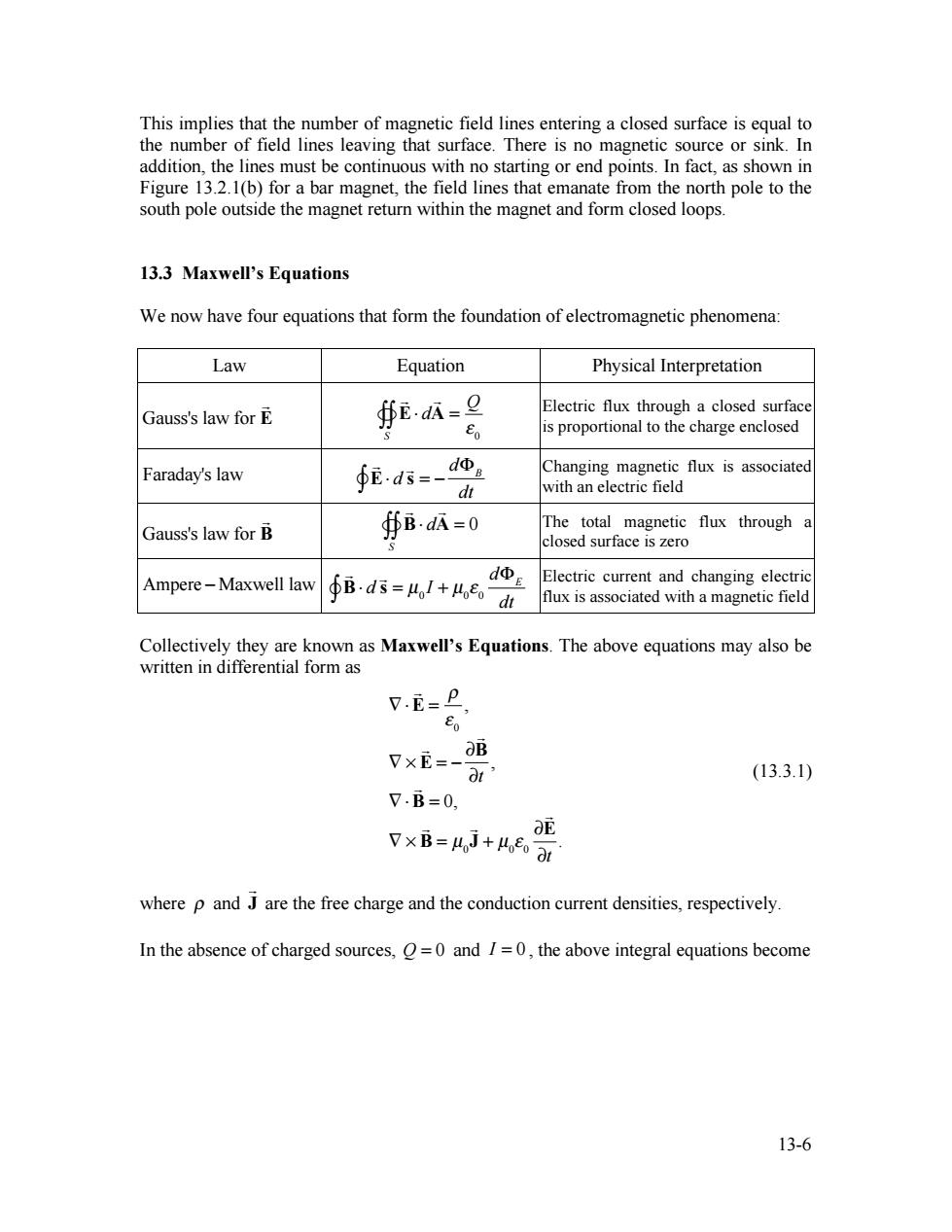
This implies that the number of magnetic field lines entering a closed surface is equal to the number of field lines leaving that surface.There is no magnetic source or sink.In addition,the lines must be continuous with no starting or end points.In fact,as shown in Figure 13.2.1(b)for a bar magnet,the field lines that emanate from the north pole to the south pole outside the magnet return within the magnet and form closed loops. 13.3 Maxwell's Equations We now have four equations that form the foundation of electromagnetic phenomena: Law Equation Physical Interpretation Gauss's law for E ∯E.A= Electric flux through a closed surface Eo is proportional to the charge enclosed Faraday's law ∮Ed5=-Φ2 Changing magnetic flux is associated with an electric field Gauss's law for B ∯B=0 The total magnetic flux through a closed surface is zero Ampere-Maxwell law dΦE fB.ds=1+4e。di Electric current and changing electric flux is associated with a magnetic field Collectively they are known as Maxwell's Equations.The above equations may also be written in differential form as V.E=P Eo P×E=-那 (13.3.1) 7.B=0, V×B=4,J+ea where p and J are the free charge and the conduction current densities,respectively. In the absence of charged sources,O=0 and I=0,the above integral equations become 13-613-6 This implies that the number of magnetic field lines entering a closed surface is equal to the number of field lines leaving that surface. There is no magnetic source or sink. In addition, the lines must be continuous with no starting or end points. In fact, as shown in Figure 13.2.1(b) for a bar magnet, the field lines that emanate from the north pole to the south pole outside the magnet return within the magnet and form closed loops. 13.3 Maxwell’s Equations We now have four equations that form the foundation of electromagnetic phenomena: Law Equation Physical Interpretation Gauss's law for E ! ! E! d ! A S """ = Q # 0 Electric flux through a closed surface is proportional to the charge enclosed Faraday's law ! E! d ! s = " d#B dt "$ Changing magnetic flux is associated with an electric field Gauss's law for B ! ! B! d ! A S """ = 0 The total magnetic flux through a closed surface is zero Ampere ! Maxwell law ! B! d ! s = µ0 I + µ0 " 0 d#E dt "$ Electric current and changing electric flux is associated with a magnetic field Collectively they are known as Maxwell’s Equations. The above equations may also be written in differential form as ! " ! E = # $ 0 , ! % ! E = & ' ! B 't , ! " ! B = 0, ! % ! B = µ0 ! J + µ0 $ 0 ' ! E 't . (13.3.1) where ! and J ! are the free charge and the conduction current densities, respectively. In the absence of charged sources, Q = 0 and I = 0 , the above integral equations become