正在加载图片...
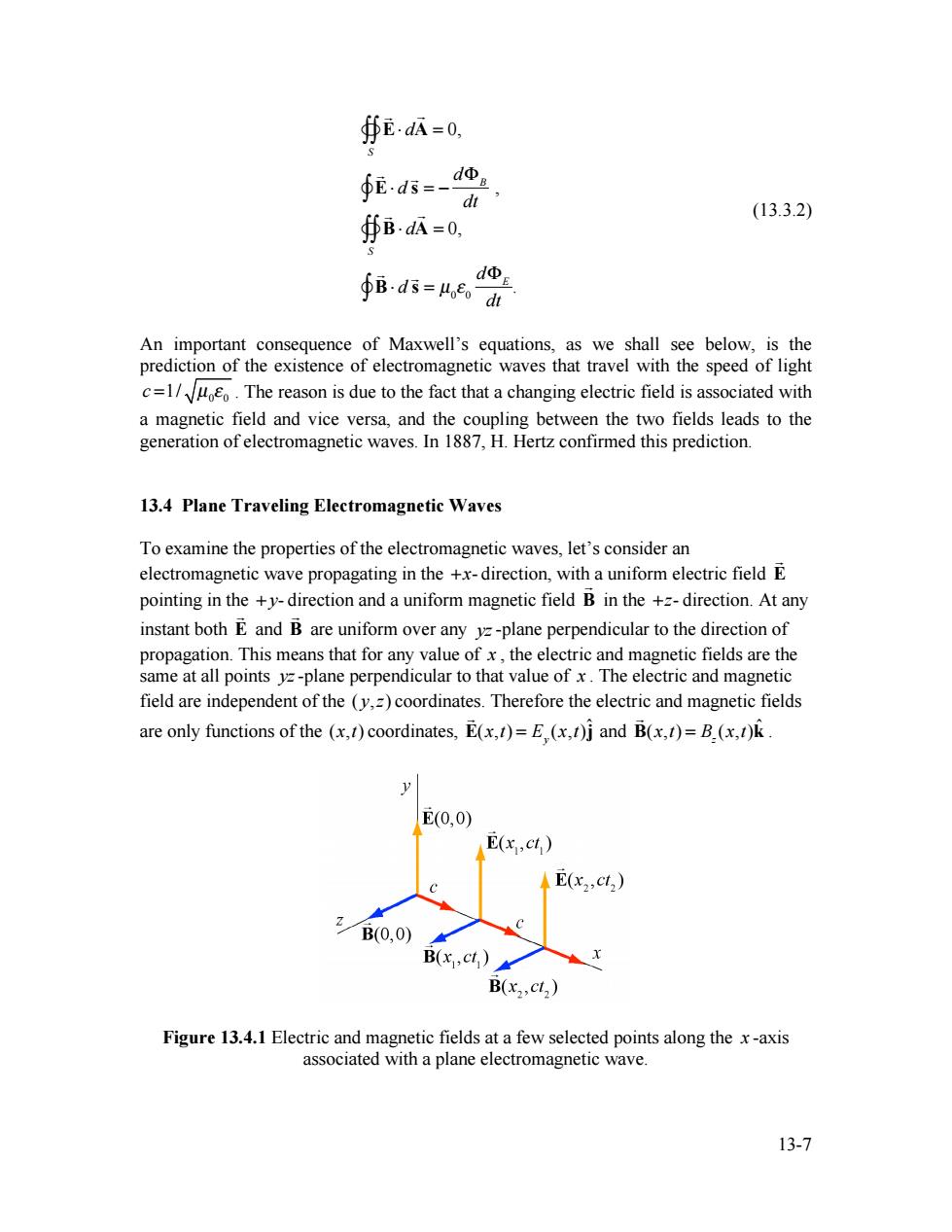
∯EdA=0, ∮2ds= dΦB dt (13.3.2) ∯B.dA=0, dΦE ∮B-ds=4edh An important consequence of Maxwell's equations,as we shall see below,is the prediction of the existence of electromagnetic waves that travel with the speed of light c=1/.The reason is due to the fact that a changing electric field is associated with a magnetic field and vice versa,and the coupling between the two fields leads to the generation of electromagnetic waves.In 1887,H.Hertz confirmed this prediction. 13.4 Plane Traveling Electromagnetic Waves To examine the properties of the electromagnetic waves,let's consider an electromagnetic wave propagating in the +x-direction,with a uniform electric field E pointing in the +y-direction and a uniform magnetic field B in the +z-direction.At any instant both E and B are uniform over any yz-plane perpendicular to the direction of propagation.This means that for any value of x,the electric and magnetic fields are the same at all points yz-plane perpendicular to that value ofx.The electric and magnetic field are independent of the (y,z)coordinates.Therefore the electric and magnetic fields are only functions of the (x,t)coordinates,E(x,t)=E (x,t)j and B(x,t)=B.(x,t)k E(0,0) E(x,ct) E(x2,ct2) B(0,0) B(x.cl) B(x,,ct) Figure 13.4.1 Electric and magnetic fields at a few selected points along the x-axis associated with a plane electromagnetic wave. 13-713-7 ! E! d ! A S """ = 0, ! E! d ! s = # d$B dt #" , ! B! d ! A S """ = 0, ! B! d ! s = µ0 % 0 d$E dt #" . (13.3.2) An important consequence of Maxwell’s equations, as we shall see below, is the prediction of the existence of electromagnetic waves that travel with the speed of light 0 0 c =1/ µ ! . The reason is due to the fact that a changing electric field is associated with a magnetic field and vice versa, and the coupling between the two fields leads to the generation of electromagnetic waves. In 1887, H. Hertz confirmed this prediction. 13.4 Plane Traveling Electromagnetic Waves To examine the properties of the electromagnetic waves, let’s consider an electromagnetic wave propagating in the +x- direction, with a uniform electric field E ! pointing in the + y- direction and a uniform magnetic field B ! in the +z- direction. At any instant both E ! and B ! are uniform over any yz-plane perpendicular to the direction of propagation. This means that for any value of x , the electric and magnetic fields are the same at all points yz-plane perpendicular to that value of x . The electric and magnetic field are independent of the ( y,z) coordinates. Therefore the electric and magnetic fields are only functions of the (x,t) coordinates, ! E(x,t) = E y (x,t)ˆ j and ! B(x,t) = Bz (x,t)kˆ . Figure 13.4.1 Electric and magnetic fields at a few selected points along the x -axis associated with a plane electromagnetic wave