正在加载图片...
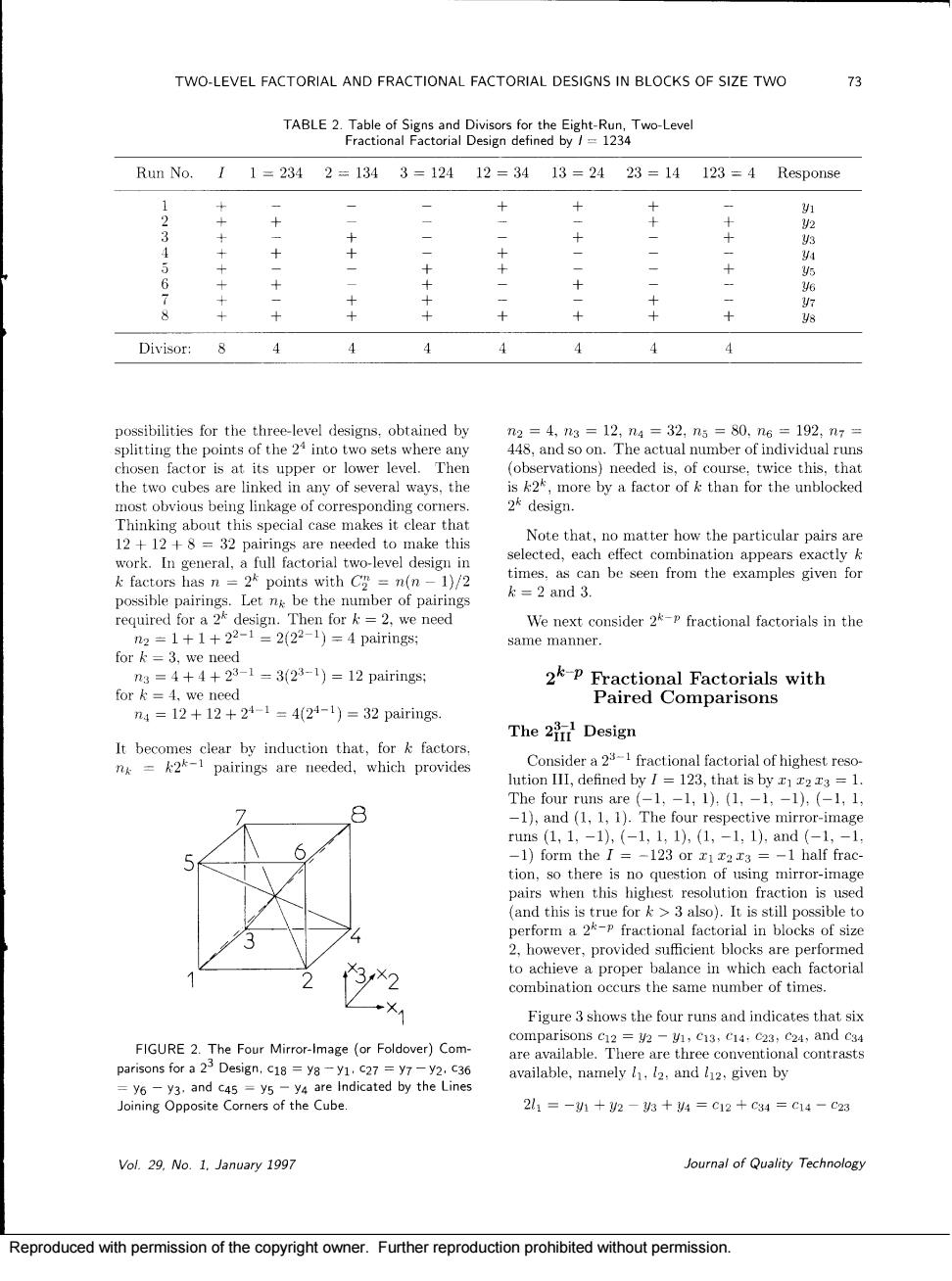
TWO-LEVEL FACTORIAL AND FRACTIONAL FACTORIAL DESIGNS IN BLOCKS OF SIZE TWO RunN0.11=2342=1343=12412=3413=2423=14123=4 Response 十 6- + + + Divisor:8 4 4 4 4 4 4 4 ibilities for th 12 =》 80 he tw cube I ways,th mctor of ha for the unlocled Thinking about this special case makes it clear that Note that,no matter how the particular pairs are -leve possible pairin Let n be the number of pairings 222e We next consider 2-fractional factorials in the same manner. =4+4+23 1 =3(23-1)12 pairings: 2%P Fra ctional Factorials with Paired Comparisons The 21 Design t is byi -1),and (1.1.D).The four pective mirror-image runs (1,1. the)(1 1.D).and tion of using mirror-im airs when this highest resolution fraction is used and thiss k>3 a 2.however.provided sufficient blocks are performed to achieve a proper balance in which each factoria combination occurs the same number of times Figure 3 shows the four runs and indicates that siy comparisons c c13,14C cand ca FIGURE 2 The Four Mirro mage for foldover)com parisons for a23 Design.18 =y y6- d the 2弘1=-1+2-+4=c12+c4=C14-c2 Vol.29.No.1,January 1997 Journal of Quality Technology Reproduced with permission of the copyright owner.Further reproduction prohibited without permission. Reproduced with permission of the copyright owner. Further reproduction prohibited without permission