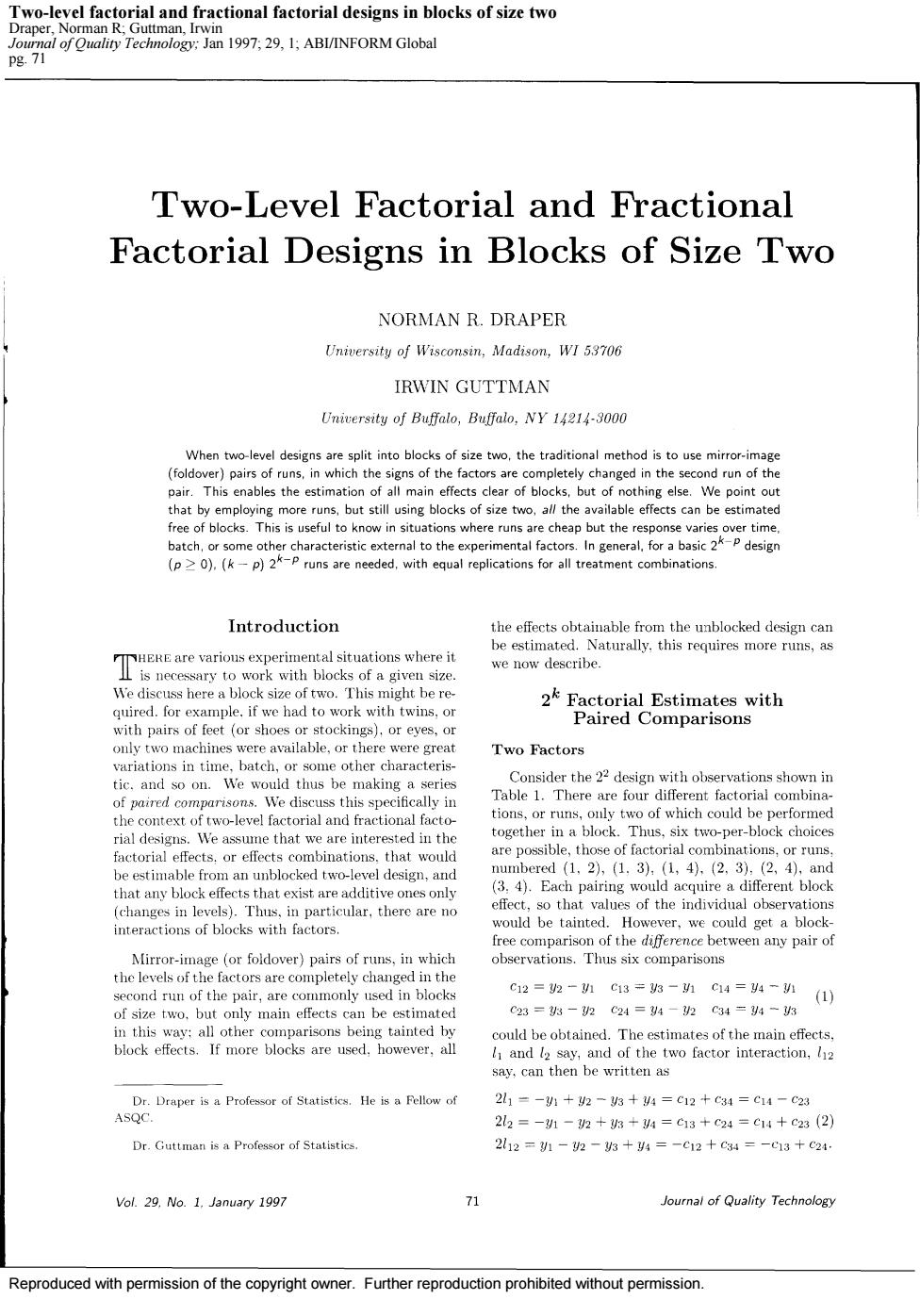
Two-level factorial and fractional factorial designs in blocks of size two .1NORM Coal Two-Level Factorial and Fractional Factorial Designs in Blocks of Size Two NORMAN R.DRAPER University of Wisconsin,Madison,WI5706 IRWIN GUTTMAN University of Buffolo,Buffalo,NY 14214-3000 the tradonmthod tomm When two-level d s but stil using blocks of si two.al isisuseful to ow in sit tothe Introduction the effects obtainable from the unblocked design can aturally.this requires more runs,as 2tEastorialLEatiatonswith only two machines were available.or there were great Two Factors acteris We discuss this specificallyn factorialadfiactionelacto of which coud be perfo Thus,six tw factorial efects.or effects combinations.that would choice design,and uuhered d.2)d.3).0 2 3).12.D.and erent block changes in levels).Thus.in particular,there are no ver.we could get a blocks interactions of blocks with factors. ond run of the pair,are commonly used in block C2=-1C13=6一功C14=y4-1 hewo.bu C23=-欢24=4-欢4=4- block effects.If more blocks are used.however,all sav.can then be written as 2弘1=-1+h-+=c12+c3=c14-c2 22=-1-+班+=G3+c24=c14+c23(2) Dr.Guttman is a Professor of Statistic 212=1一2一+4 -C12十C34 一C3十24 Vol.29.No.1.January 1997 71 Journal of Quality Technology Reproduced with permission of the copyright owner.Further reproduction prohibited without permission
Reproduced with permission of the copyright owner. Further reproduction prohibited without permission. Two-level factorial and fractional factorial designs in blocks of size two Draper, Norman R; Guttman, Irwin Journal of Quality Technology; Jan 1997; 29, 1; ABI/INFORM Global pg. 71
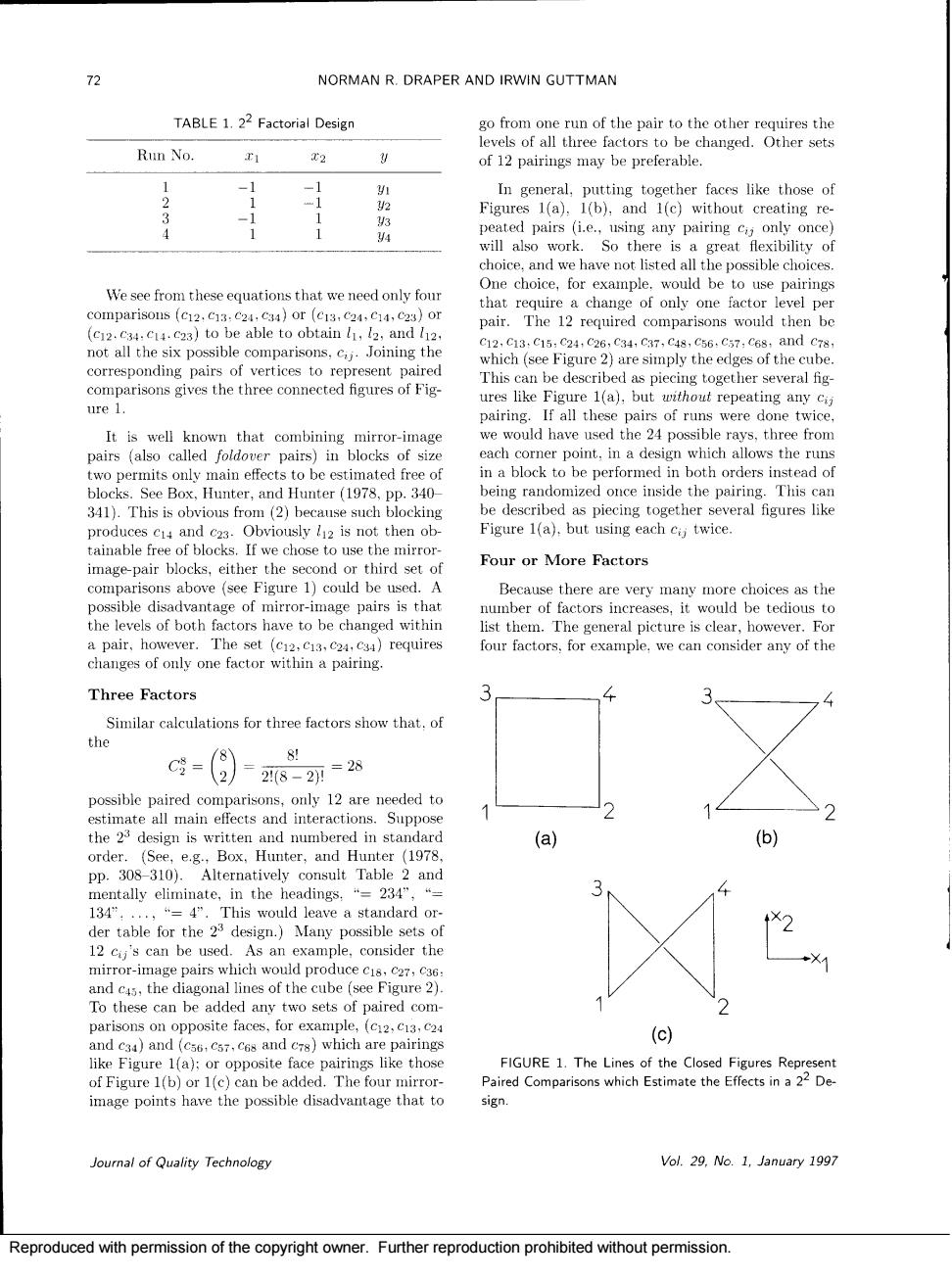
72 NORMAN R.DRAPER AND IRWIN GUTTMAN TABLE 1.22 Factorial Design Run No. 2 of 12 pairings may be preferable. aces like thseo 4 ted pairs (i.e. using any pairing ca only once So th a great fle We see from these equations that we need only four el r comparisons (c12. and c ges of the eub It is well k e each corner point.in a design which allows the runs to be estimated free a block to be performed in both orders in tead o 341).This and Obviously h2 is not then ob Figure 1(a).but using each ca twice. ainable I we chose use hemirrol Four or More Factors comparisons above (see Figure D could be used Because there are very many more choices as the that mumber of factors increases,it would be tedious to pair.how The set (e .a.,reguire changes of only one factor within a pairing Three Factors Similar calculations for three factors show that,of the 8 cts a (a) order. (Se Bo.(7. hernaitielycost and de ign)Many possible parisons on opposite faces.for example. k and (c) 56,c57,C66an0d EIGURE 1 The Lines a the Closed E: image points have the possible disadvantage that to sign Vol.29.No.1,January 1997 Reproduced with permission of the copyright owner.Further reproduction prohibited without permission
Reproduced with permission of the copyright owner. Further reproduction prohibited without permission
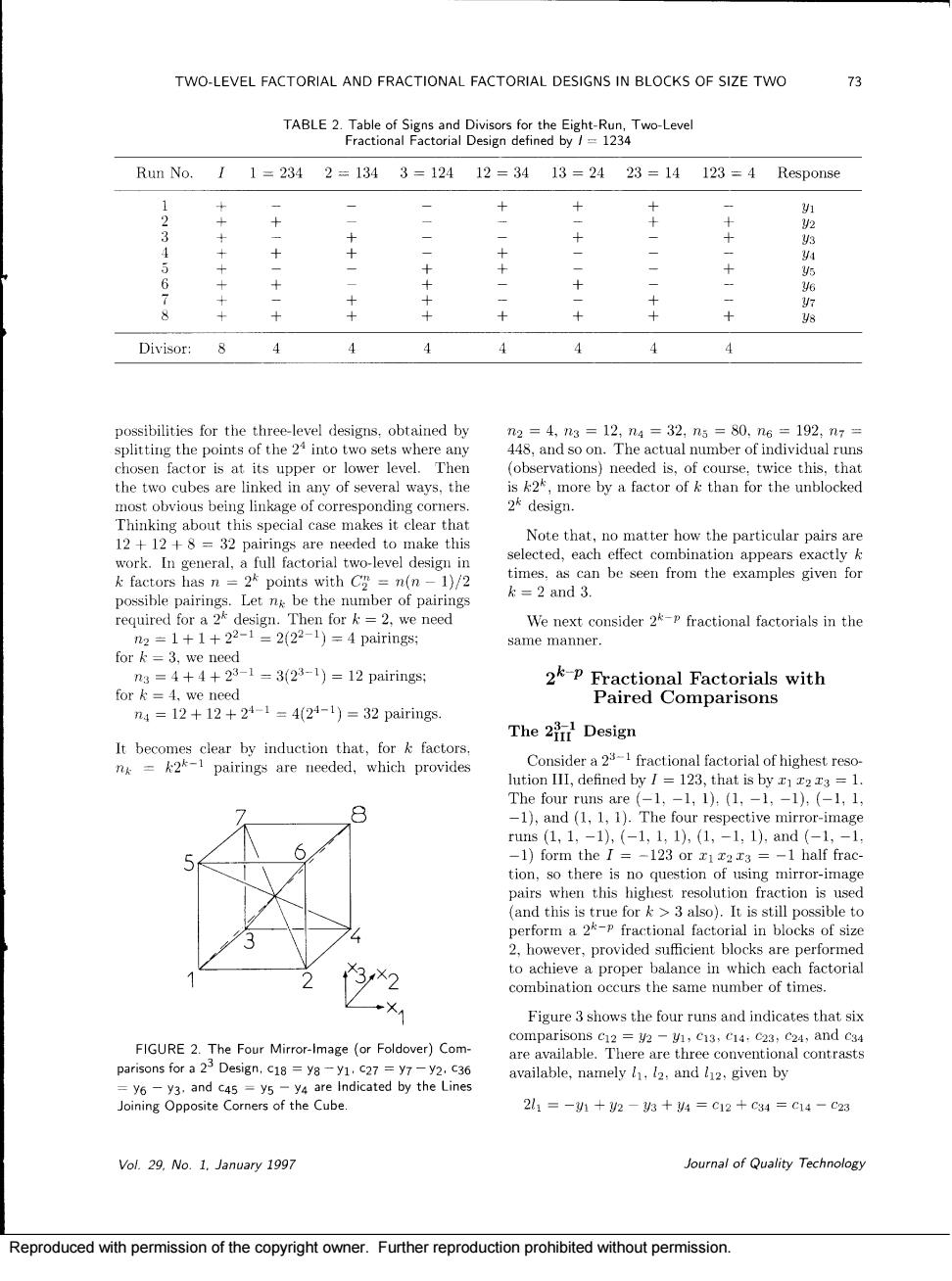
TWO-LEVEL FACTORIAL AND FRACTIONAL FACTORIAL DESIGNS IN BLOCKS OF SIZE TWO RunN0.11=2342=1343=12412=3413=2423=14123=4 Response 十 6- + + + Divisor:8 4 4 4 4 4 4 4 ibilities for th 12 =》 80 he tw cube I ways,th mctor of ha for the unlocled Thinking about this special case makes it clear that Note that,no matter how the particular pairs are -leve possible pairin Let n be the number of pairings 222e We next consider 2-fractional factorials in the same manner. =4+4+23 1 =3(23-1)12 pairings: 2%P Fra ctional Factorials with Paired Comparisons The 21 Design t is byi -1),and (1.1.D).The four pective mirror-image runs (1,1. the)(1 1.D).and tion of using mirror-im airs when this highest resolution fraction is used and thiss k>3 a 2.however.provided sufficient blocks are performed to achieve a proper balance in which each factoria combination occurs the same number of times Figure 3 shows the four runs and indicates that siy comparisons c c13,14C cand ca FIGURE 2 The Four Mirro mage for foldover)com parisons for a23 Design.18 =y y6- d the 2弘1=-1+2-+4=c12+c4=C14-c2 Vol.29.No.1,January 1997 Journal of Quality Technology Reproduced with permission of the copyright owner.Further reproduction prohibited without permission
Reproduced with permission of the copyright owner. Further reproduction prohibited without permission
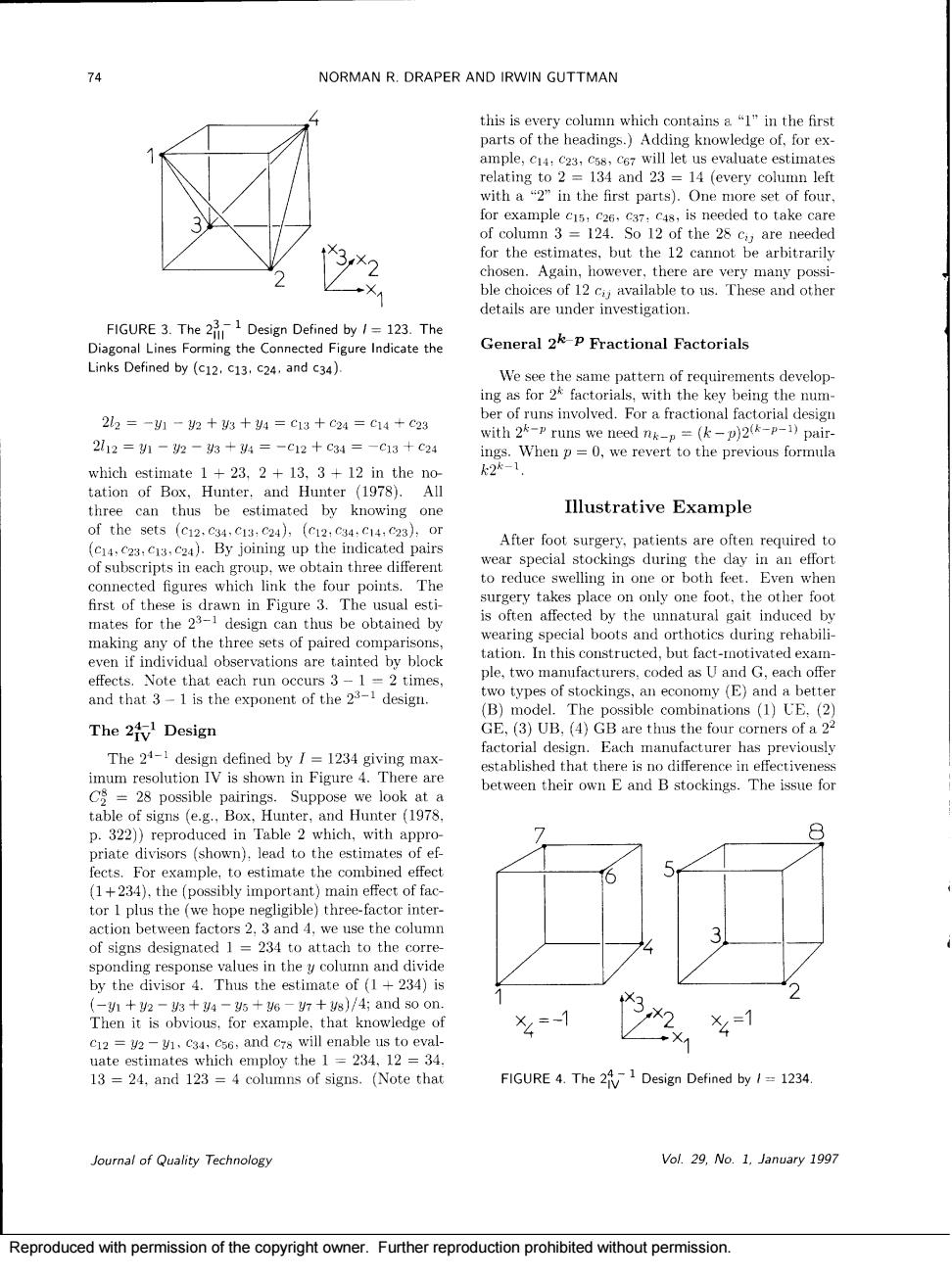
74 NORMAN R.DRAPER AND IRWIN GUTTMAN this is every column which contains a"1"in the first apec23%23et5 ate with a"2"in the first parts).One more set of four osen.Again,however,there are very many po e to us.These and other General 2k-P Fractional Factorial Links Defined by (c12.c13.c24.and c34) 2l2=-1-2+3十1=C13+C24=C14+C23 with 2 212=1-2-为+4=-012+c34=-C13+c24 pa and Hunter Illustrative Example to reduce sweing both feet connected figures which link the four points. Th ral gai making any of the three sets of paired comparison even if individual observations are tainted by block tation.In this constructed,but fact-motivated exam ple.two manufacturers,coded as U and G.each ofer ny CE The 21 Design GE,(3)UB,()GB are thus the four corners of a 2 Tn之 s shown in Figure een their B The issue for table of signs(e.g.Box,Hunter.and Hunter (197 (1+234).the (possibly important)main effect of fac tor I plus the (w of signs designated 1=234 to attach to the corre by the diviso Then itis for example.that knowledge 名=-1 3 =1 f signs. (Note that FIGURE 4.The21 Design Defined by /-1234. Journal of Quality Technology Vol.29,No.1.January 1997 Reproduced with permission of the copyright owner.Further reproduction prohibited without permission
Reproduced with permission of the copyright owner. Further reproduction prohibited without permission

TWO-LEVEL FACTORIAL AND FRACTIONAL FACTORIAL DESIGNS IN BLOCKS OF SIZE TWO 75 TABLE 3.Results from Paired Comparison 22 Design Testers Treatments Combination Results,Days Comparisons E GB the orthopedic staff and their HMO (Health Main Whatever specific choice is made for 7.we can fol- sible. y high,so gs were nee prices c Variation between patients is very the four types. sense o use washed and dried overnight,and worn until they be come damaged and ineffective,when the numberso tions dependent onan assumed (oron the observed) mean valuei Conclusion are given in Table 3. m ons.In gen tion 4/21. rod that is (k-p)locke runs or )times the number of runs in ter quality stockings.Also the better stockings last t. type mar make sense when runs are extremely variable ove ment average values from these data due to the un- ded The ve the est be back-solved to give individual pseudo-data values ould be th mean value for the data.The estimation equations Acknowledgments +2+g+4=4(unknown) We thank both referees for a very detailed analy +-+=30 -++4=126 under Grant MDA04-95-H-1020 from the National 1-2-始+4=22 Security Agency. References data,484/8=60.5,as y.We then obtain the array of mean responses G Key Words:Blocking.Factorial Designs.Fructional Factorial Designs. Vol.29.No.1.January 1997 Journal of Quality Technology Reproduced with permission of the copyright owner.Further reproduction prohibited without permission
Reproduced with permission of the copyright owner. Further reproduction prohibited without permission