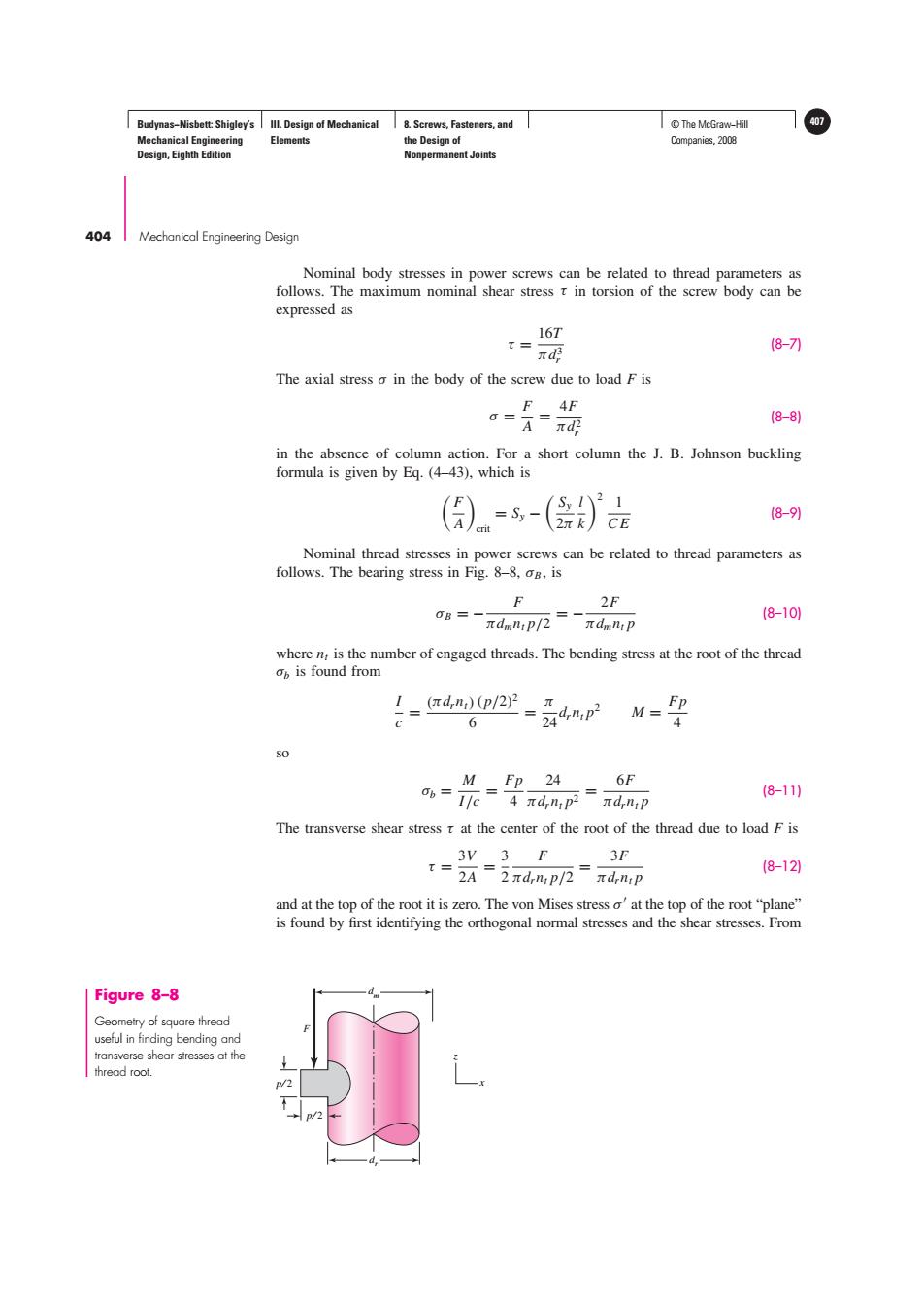
Budynas-Nisbett:Shigley's Ill.Design of Mechanical 8.Screws,Fasteners,and T©The McGraw-Hill 07 Mechanical Engineering Elements the Design of Companies,2008 Design,Eighth Edition Nonpermanent Joints 404 Mechanical Engineering Design Nominal body stresses in power screws can be related to thread parameters as follows.The maximum nominal shear stress t in torsion of the screw body can be expressed as 16T T= πd (8-7 The axial stress a in the body of the screw due to load F is F 4F =A=元d 8-8) in the absence of column action.For a short column the J.B.Johnson buckling formula is given by Eq.(4-43),which is (份)=-() (8-91 Nominal thread stresses in power screws can be related to thread parameters as follows.The bearing stress in Fig.8-8,og,is 2F B=一 dan,p/2= (8-101 πdmnip where n,is the number of engaged threads.The bending stress at the root of the thread op is found from -42心-4pM= 6 sO M Fp 24 6F %=元=4dn,p=4m,p (8-11) The transverse shear stress r at the center of the root of the thread due to load F is 3V 3 F 3F 【=2A=2rd,np/2-Td,np (8-12) and at the top of the root it is zero.The von Mises stress o'at the top of the root"plane" is found by first identifying the orthogonal normal stresses and the shear stresses.From Figure 8-8 Geometry of square thread useful in finding bending and transverse shear stresses at the thread root.Budynas−Nisbett: Shigley’s Mechanical Engineering Design, Eighth Edition III. Design of Mechanical Elements 8. Screws, Fasteners, and the Design of Nonpermanent Joints © The McGraw−Hill 407 Companies, 2008 404 Mechanical Engineering Design dm dr F p ⁄ 2 p ⁄ 2 z x Figure 8–8 Geometry of square thread useful in finding bending and transverse shear stresses at the thread root. Nominal body stresses in power screws can be related to thread parameters as follows. The maximum nominal shear stress τ in torsion of the screw body can be expressed as τ = 16T πd3 r (8–7) The axial stress σ in the body of the screw due to load F is σ = F A = 4F πd2 r (8–8) in the absence of column action. For a short column the J. B. Johnson buckling formula is given by Eq. (4–43), which is F A  crit = Sy − Sy 2π l k 2 1 C E (8–9) Nominal thread stresses in power screws can be related to thread parameters as follows. The bearing stress in Fig. 8–8, σB, is σB = − F πdmnt p/2 = − 2F πdmnt p (8–10) where nt is the number of engaged threads. The bending stress at the root of the thread σb is found from I c = (πdrnt)(p/2)2 6 = π 24 drnt p2 M = Fp 4 so σb = M I/c = Fp 4 24 πdrnt p2 = 6F πdrnt p (8–11) The transverse shear stress τ at the center of the root of the thread due to load F is τ = 3V 2A = 3 2 F πdrnt p/2 = 3F πdrnt p (8–12) and at the top of the root it is zero. The von Mises stress σ at the top of the root “plane” is found by first identifying the orthogonal normal stresses and the shear stresses. From