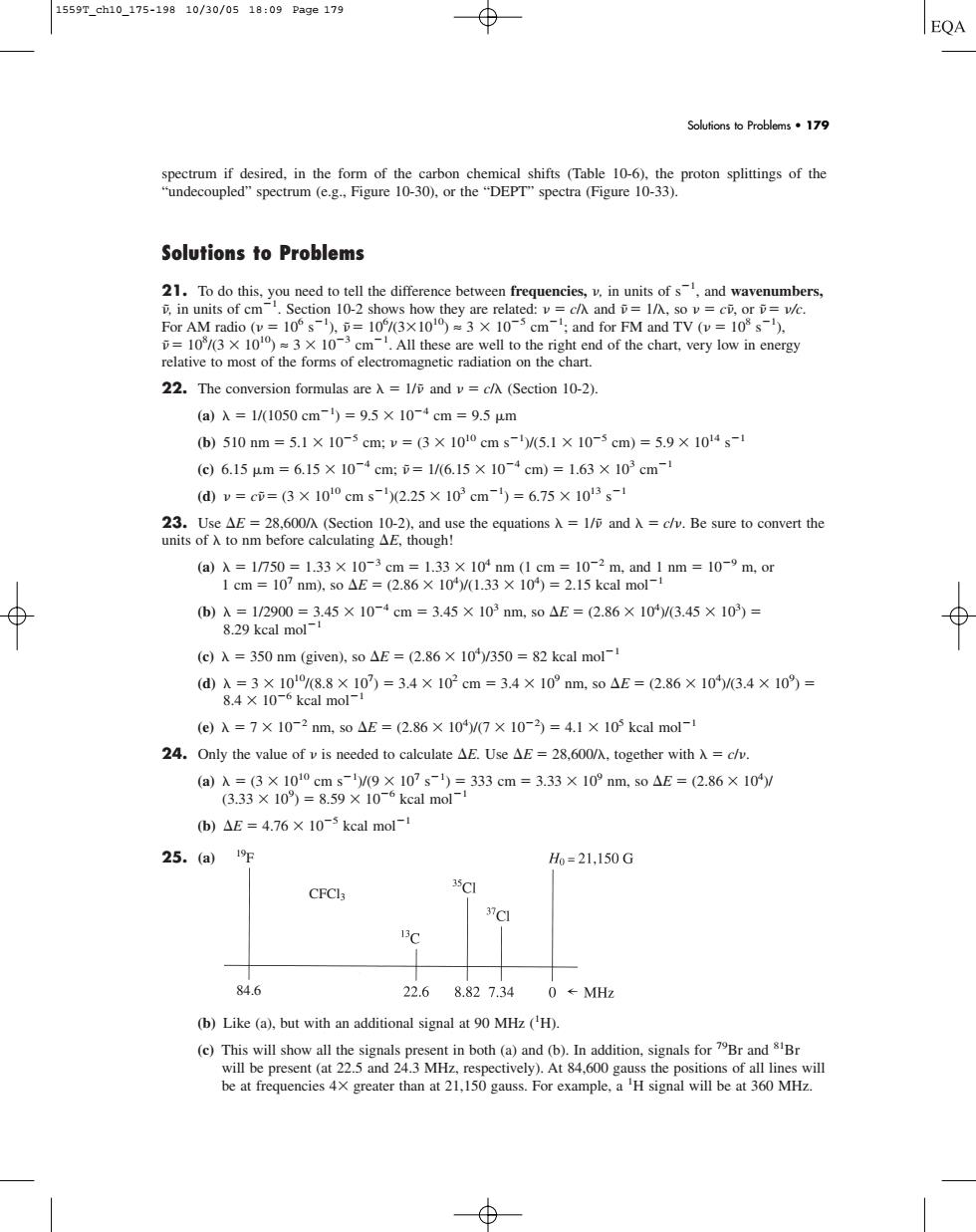
15597ch10175-19810/30/0518:09Page179 EQA Solutions to Problems179 spectrum (e.g..Pigure spectra Solutions to Problems 21.To do this.you need to tell the difference between frequencies,v.in units of sand wavenumbers, on 10-2 sh 6 EM =1013×100= relative to most of the forms of electromagnetic radiation on the chart. 22.The conversion formulas are=1/p andv=c(Section 10-2). (a)λ=1/1050cm-)=9.5×10-4cm=9.5um d)510nm=5.1×10-5cmp=(3×100cms-5.1×10-5cm=5.9×104s (g6.15μm=6.15×10-4cm:=1/6.15×10-4cm)=1.63×103cm (dv=c=(6×10cms-2.25×103cm-=6.75×103s1 23.Use AE=28.600/A(Section 10-2).and use the equations=1/and =c/v.Be sure to convert the units of入to nm before calculating△E.though (a)=1750=1.33×10-3cm=1.33×10nm1 ☒ (c)λ=350nm(given).s0△E=(2.86×10/350=82 keal mol- @A10280=34×1cm=34×10m0△E=6×104×10- (e)=7×10-2m,s0△E=(2.86×107×10)=4.1×10 kcal mol 24.Only the value of vis needed to calculate AE.Use AE=28,600/A.together with=c/v. (b)AE=4.76 X 10-5 kcal mol-1 25.(a1℉ H0=21,150G 84.6 22.68.827.340←MHz (b)Like (a),but with an additional signal at 90 MHz (H). s will be at frequencies 4X greater than at 21.150 gauss.For example.a'H signal will be at 360 MHz.spectrum if desired, in the form of the carbon chemical shifts (Table 10-6), the proton splittings of the “undecoupled” spectrum (e.g., Figure 10-30), or the “DEPT” spectra (Figure 10-33). Solutions to Problems 21. To do this, you need to tell the difference between frequencies, , in units of s1 , and wavenumbers, ˜, in units of cm1 . Section 10-2 shows how they are related:  c/ and ˜ 1/ , so  c˜, or ˜ /c. For AM radio ( 106 s 1 ), ˜ 106 /(31010) ≈ 3  105 cm1 ; and for FM and TV ( 108 s 1 ), ˜ 108 /(3  1010) ≈ 3  103 cm1 . All these are well to the right end of the chart, very low in energy relative to most of the forms of electromagnetic radiation on the chart. 22. The conversion formulas are 1/˜ and  c/ (Section 10-2). (a) 1/(1050 cm1 ) 9.5  104 cm 9.5 m (b) 510 nm 5.1  105 cm;  (3  1010 cm s1 )/(5.1  105 cm) 5.9  1014 s 1 (c) 6.15 m 6.15  104 cm; ˜ 1/(6.15  104 cm) 1.63  103 cm1 (d)  c˜ (3  1010 cm s1 )(2.25  103 cm1 ) 6.75  1013 s 1 23. Use E 28,600/ (Section 10-2), and use the equations 1/˜ and c/. Be sure to convert the units of to nm before calculating E, though! (a) 1/750 1.33  103 cm 1.33  104 nm (1 cm 102 m, and 1 nm 109 m, or 1 cm 107 nm), so E (2.86  104 )/(1.33  104 ) 2.15 kcal mol1 (b) 1/2900 3.45  104 cm 3.45  103 nm, so E (2.86  104 )/(3.45  103 ) 8.29 kcal mol1 (c) 350 nm (given), so E (2.86  104 )/350 82 kcal mol1 (d) 3  1010/(8.8  107 ) 3.4  102 cm 3.4  109 nm, so E (2.86  104 )/(3.4  109 ) 8.4  106 kcal mol1 (e) 7  102 nm, so E (2.86  104 )/(7  102 ) 4.1  105 kcal mol1 24. Only the value of  is needed to calculate E. Use E 28,600/ , together with c/. (a) (3  1010 cm s1 )/(9  107 s 1 ) 333 cm 3.33  109 nm, so E (2.86  104 )/ (3.33  109 ) 8.59  106 kcal mol1 (b) E 4.76  105 kcal mol1 25. (a) (b) Like (a), but with an additional signal at 90 MHz (1 H). (c) This will show all the signals present in both (a) and (b). In addition, signals for 79Br and 81Br will be present (at 22.5 and 24.3 MHz, respectively). At 84,600 gauss the positions of all lines will be at frequencies 4 greater than at 21,150 gauss. For example, a 1 H signal will be at 360 MHz. CFCl3 84.6 19F Solutions to Problems • 179 1559T_ch10_175-198 10/30/05 18:09 Page 179