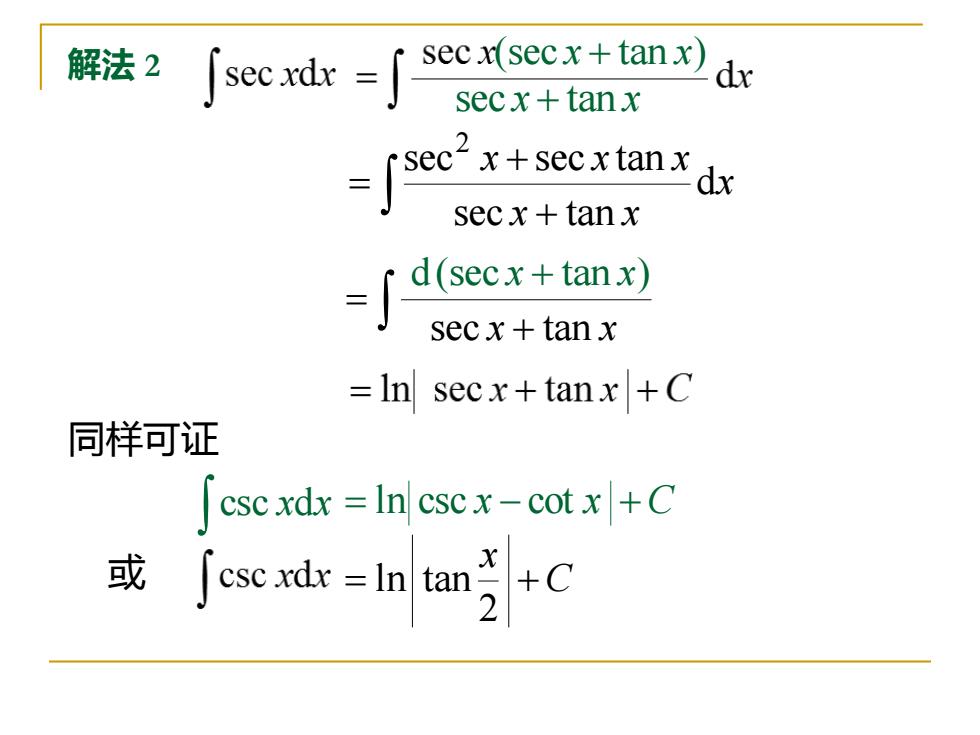
解法2 ∫secxx=∫ secx(secx+tanx) dx secx+tanx =∫Scf+secxtanx secx+tanx d(secx+tanx) secx+tanx =In secx+tanx +C 同样可证 csc xdx Incscx-cot x+C 或 ∫cd=lnam2+C  + = sec x tan x 解法 2 sec x + tan x (sec x + tan x) x x x x x x d sec tan sec sec tan 2  + + = d(sec x + tan x) 同样可证  csc xdx = ln csc x − cot x +C 或 C x = + 2 ln tan