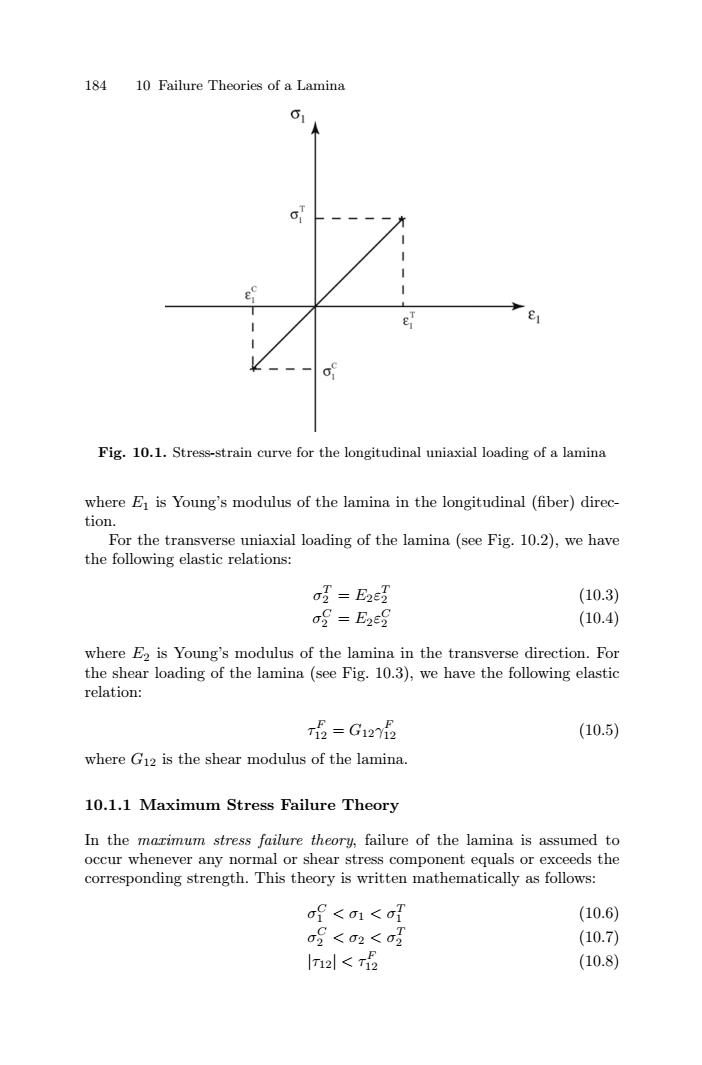
184 10 Failure Theories of a Lamina 1 e e Fig.10.1.Stress-strain curve for the longitudinal uniaxial loading of a lamina where E is Young's modulus of the lamina in the longitudinal (fiber)direc- tion. For the transverse uniaxial loading of the lamina (see Fig.10.2),we have the following elastic relations: g=E2话 (10.3) =E2eS (10.4) where E2 is Young's modulus of the lamina in the transverse direction.For the shear loading of the lamina (see Fig.10.3),we have the following elastic relation: T12 G12712 (10.5) where G12 is the shear modulus of the lamina. 10.1.1 Maximum Stress Failure Theory In the marimum stress failure theory,failure of the lamina is assumed to occur whenever any normal or shear stress component equals or exceeds the corresponding strength.This theory is written mathematically as follows: o9<1<l (10.6) S<2< (10.7) Inal< (10.8)184 10 Failure Theories of a Lamina Fig. 10.1. Stress-strain curve for the longitudinal uniaxial loading of a lamina where E1 is Young’s modulus of the lamina in the longitudinal (fiber) direc￾tion. For the transverse uniaxial loading of the lamina (see Fig. 10.2), we have the following elastic relations: σT 2 = E2εT 2 (10.3) σC 2 = E2εC 2 (10.4) where E2 is Young’s modulus of the lamina in the transverse direction. For the shear loading of the lamina (see Fig. 10.3), we have the following elastic relation: τ F 12 = G12γF 12 (10.5) where G12 is the shear modulus of the lamina. 10.1.1 Maximum Stress Failure Theory In the maximum stress failure theory, failure of the lamina is assumed to occur whenever any normal or shear stress component equals or exceeds the corresponding strength. This theory is written mathematically as follows: σC 1 < σ1 < σT 1 (10.6) σC 2 < σ2 < σT 2 (10.7) |τ12| < τ F 12 (10.8)