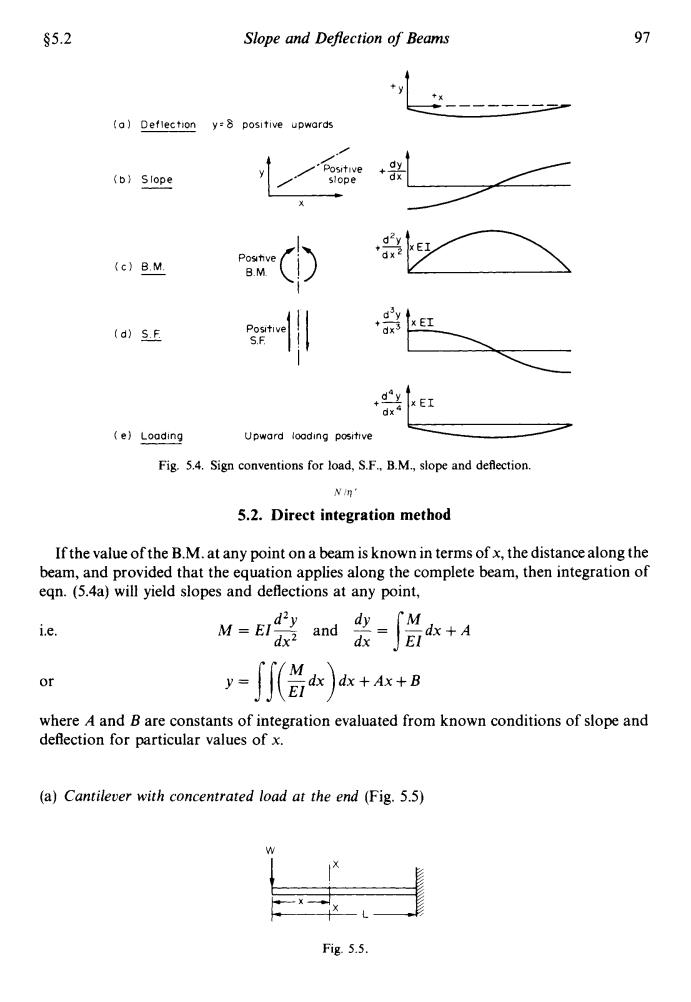
§5.2 Slope and Deflection of Beams 97 (a)Deflection y=8 positive upwards Positive dy (b)Slope slope d d" Postve dx (c)B.M. B.M (d)S.F (e)Loading Upward loading positive Fig.5.4.Sign conventions for load,S.F..B.M.,slope and deflection. N in' 5.2.Direct integration method If the value of the B.M.at any point on a beam is known in terms of x,the distance along the beam,and provided that the equation applies along the complete beam,then integration of eqn.(5.4a)will yield slopes and deflections at any point, ie. dx+A or y=∬(0x++8 where A and B are constants of integration evaluated from known conditions of slope and deflection for particular values of x. (a)Cantilever with concentrated load at the end (Fig.5.5) Fig.5.5.45.2 Slope and Deflection of Beams 97 (a) Deflection y=8 positive upwards (e) Loading Upward loading positive +a .,:.i XEI , Fig. 5.4. Sign conventions for load, S.F., B.M., slope and deflection. Nlq' 5.2. Direct integration method If the value ofthe B.M. at any point on a beam is known in terms of x, the distance along the beam, and provided that the equation applies along the complete beam, then integration of eqn. (5.4a) will yield slopes and deflections at any point, i.e. or dxy s" El y = Is( Zdx) dx +Ax + B d2Y M = EI, and -= --dx+A dx where A and B are constants of integration evaluated from known conditions of slope and deflection for particular values of x. (a) Cantilever with concentrated load at the end (Fig. 5.5) w Fig. 5.5